Annulenes can twist, and I have blogged about a number of examples (cations and neutrals). The twist can be portioned into a twist associated with the dihedral angle as one progresses around the compound and writhe which is associated with distortion of the annulene into a third dimension.¹

Herges has prepared the 36-annulene 1 where the anthracenyl units were introduced to force the loop out of plane.² Four different conformations of 1 where isolated by crystallization out of different solvents. 1a and 1b were produced in benzene, and they differ by having two half-twists (L_k=2) and one half-twist (L_k=1), respectively. 1c was isolated from DMF, with one half-twist. 1d was isolated from Et₂O/CH₂Cl₂.

Computations at the B3LYP/6-31g* level identified 10 low lying conformations, and the ones corresponding to the experimentally observed forms are shown in Figure 1. The computed conformer that matches with 1d differs form the experimental version by a rotation about one single bond, and the computed version has a different topology than the experiment. One item of note is that all of the 10 computed conformers are dominated by the twist (T_w) and have very small writhe.

1a	1b
1c	1d
1e

Figure 1. B3LYP/6-31G* optimized structures of 1a-e.

Though not isolated in experiment, one of the low lying conformers has three half-twists (L_k=3) and is also shown in Figure 1 as 1e. Identification of this highly twisted species would be quite interesting.

References

(1) Fowler, P. W.; Rzepa, H. S., "Aromaticity rules for cycles with arbitrary numbers of half-twists," Phys. Chem. Chem. Phys., 2006, 8, 1775-1777, DOI: 10.1039/b601655c.

(2) Mohebbi, A.; Mucke, E. K.; Schaller, G.; Köhler, F.; Sönnichsen, F.; Ernst, L.; Näther, C.; Herges, R., "Singly and Doubly Twisted [36]Annulenes: Synthesis and Calculations," Chem. Eur. J., 2010, 16, 7767-7772, DOI: 10.1002/chem.201000277,

InChIs

1: InChI=1/C80H52/c1-9-25-65-57(17-1)73-45-41-53-33-35-54(36-34-53)42-46-75-61-21-5-13-29-69(61)79(70-30-14-6-22-62(70)75)51-52-80-71-31-15-7-23-63(71)76(64-24-8-16-32-72(64)80)48-44-56-39-37-55(38-40-56)43-47-74-59-19-3-11-27-67(59)78(68-28-12-4-20-60(68)74)50-49-77(65)66-26-10-2-18-58(66)73/h1-52H/b45-41-,46-42+,47-43-,48-44+,50-49+,52-51-,53-41-,54-42-,55-43-,56-44-,73-45-,74-47-,75-46-,76-48-,77-49-,78-50+,79-51+,80-52+
InChIKey=KXWPWHPRFOXOIX-IFDMNBDGBZ

Here’s a nice example of the productive interplay between experiment and computations.¹ The dipeptide N-Acyl-Ala-Ala-Benzyl was prepared and subjected to UV and IR/UV analysis. The IR showed two separate structures with distinctly different environments for the NH bonds: one structure showed intramolecular hydrogen bonding while the other did not.

B97/TZVPP computations revealed two structures. The first is a linear dipeptide with intramolecular hydrogen bonding occurring in a 5,5 relationship. (There are actually three conformers of this but all have similar energy, only one is shown in Figure 1.) The second structure displays a bent shape with a NH-π interaction, also shown in Figure 1. The computed vibrational spectra for each structure matches up well with the NH region of the experimental IR.

Figure 1. B97-D/TZVPP optimized structures of N-Acyl-Ala-Ala-Benzyl.

The authors spend a great deal of time noting that the 0 K energies predict that the second structure, being 4 kcal mol^-1 more stable, should be the only one observed. However, since the jet cooling will likely trap the structures at their 300 K distribution, this could account for the existence of two structures. However, when the computations include entropy corrections, so now we’re looking at ΔG(200 K), B97-D and MO6-2x suggest that the two structures are very close in energy. But they caution that MP2 predicts a large energy gap unless atomic counterpoise corrections are used to account for intramolecular basis set superposition (see this post), a problem that appears to be much less severe with the DFT methods.

References

(1) Gloaguen, E.; de Courcy, B.; Piquemal, J. P.; Pilme, J.; Parisel, O.; Pollet, R.; Biswal, H. S.; Piuzzi, F.; Tardivel, B.; Broquier, M.; Mons, M. J. Am. Chem. Soc, 2010, 132, 11860-11863, DOI: 10.1021/ja103996q

InChIs

InChI=1/C15H20N2O4/c1-10(16-12(3)18)14(19)17-11(2)15(20)21-9-13-7-5-4-6-8-13/h4-8,10-11H,9H2,1-3H3,(H,16,18)(H,17,19)/t10-,11-/m0/s1/f/h16-17H
InChIKey=KRIKKPGWLXOEAS-VFIKCTIADD

Somehow I missed this paper when it came out a few months ago, even though I was aware it was coming – as I mentioned it in one of my previous posts!

Anyways, Schreiner and Allen reported on their third study of hydroxyl carbenes (see these posts on dihydroxymethylene and hydroxymethylene), this time examining phenylhydroxycarbene.¹ As I covered in my book, there is a lot of work on phenylcarbenes which typically ring expand to the cycloheptatetraene, see Reaction 1. One might expect phenylhydroxycarbene to do the same thing, i.e. 1 converting into 2 (Reaction 2). 1 is prepared by high-vacuum flash pyrolysis of phenylglyoxylic acid 3 and then capturing the product in an argon matrix at 11 K (Reaction 3).

The carbene 1 is identified through comparison of its experimental and computed (anharmonic frequencies at CCSD(T)/cc-pVDZ) IR frequencies.

No ring expansion is observed at all – Reaction 2 does not occur. Instead, 1 rearranges to benzaldehyde 4 (Reaction 4) at 11 K with a half life of 2.46 h (and a half life of 2.55 h at 20 K). The deuterated analogue does not convert to benzaldehyde and 1-d appears to be completely stable.

So, what is going on? The cis and trans forms of 1 interconvert through a barrier of 22.7 kcal mol^-1. The trans isomer can convert to benzaldehyde (the reaction is very exothermic: -50.8 kcal mol^-1) with a barrier of 28.8 kcal mol^-1 through TS1, shown in Figure 1. The cis isomer can cleave into benzene and CO (not observed) with a huge barrier of 55 kcal mol^-1. All of these barrier were computed at CCSD(T)/cc-pVQZ.

TS1

Figure 1. MP2/cc-pVDZ optimized transition state for the conversion of 1 into 4.

Benzaldehyde seems to be produced by passing through a huge barrier, something that is impossible from a thermal perspective (we’re at 11 K!). But this can be accomplished by tunneling. Tunneling probabilities were computed from the MP2/aug-cc-pVDZ intrinsic reaction path with barrier penetration integrals computed with the WKB approximation. The bottom line: the computed half-life is 3.3 h and the deuterated species is computed to have a half-life of 8700 years(!), both in excellent agreement with experimental observation. Quantum mechanical tunneling is clearly the explanation for this chemistry.

This is another fine example of the power of joint experimental/computational studies. And be on the look-out for an even more exciting case from this group. I met with Wes Allen on my recent trip to the University of Georgia and was entertained with another hydroxycarbene that undergoes quite novel tunneling!

References

(1) Gerbig, D.; Reisenauer, H. P.; Wu, C.-H.; Ley, D.; Allen, W. D.; Schreiner, P. R., "Phenylhydroxycarbene," J. Am. Chem. Soc., 2010, 132, 7273-7275, DOI: 10.1021/ja9107885

InChIs

1: InChI=1/C7H6O/c8-6-7-4-2-1-3-5-7/h1-5,8H
InChIKey=QVZIGMRPQWIGCV-UHFFFAOYAE

2: InChI=1/C7H6O/c8-7-5-3-1-2-4-6-7/h1-6H
InChIKey=QVWDCTQRORVHHT-UHFFFAOYAM

3: InChI=1/C8H6O3/c9-7(8(10)11)6-4-2-1-3-5-6/h1-5H,(H,10,11)/f/h10H
InChIKey=FAQJJMHZNSSFSM-KZFATGLACS

4: InChI=1/C7H6O/c8-6-7-4-2-1-3-5-7/h1-6H
InChIKey=HUMNYLRZRPPJDN-UHFFFAOYAE

Determination of absolute configuration remains a difficult undertaking, one usually solved by x-ray crystallography. In my book (Chapter 1.6.3) and blog (see these posts) I have noted the use of computations in conjunction with optical rotation or electronic circular dichroism as an alternative: possible configurations are optimized and their optical properties are computed and then matched against experimental spectra.

Neri and coworkers have utilized this approach to determine the absolute configuration of the chiral calix[4]arene 1.¹

Computed optical rotations (TDDFT/B3LYP/6-31G* at 5 frequencies) are compared with experimental values in Table 1. While the magnitude is off (as is typical) the sign of the activity along with the trend matches up very well for the cS configuration shown in Figure 1. It should be noted that a second conformation makes up about 10% of the Boltzmann population, and the contribution of this second configuration is included in the computed values shown in Table 1. In addition, computations at higher levels give very similar results. Lastly, the computed ECD spectrum of the cS isomer also matches up well with experiment.

Table 1. Optical rotation of the cS isomer of 1 compared with experiment

Figure 1. B3LYP/6-31G* optimized structure of the major conformation of 1.

Given the relatively low level of theory employed here, further use of this combined experimental/computational approach to obtaining absolute configurations of large molecules is encouraged.

References

(1) Talotta, C.; Gaeta, C.; Troisi, F.; Monaco, G.; Zanasi, R.; Mazzeo, G.; Rosini, C.; Neri, P., "Absolute Configuration Assignment of Inherently Chiral Calix[4]arenes using DFT Calculations of Chiroptical Properties," Org. Lett., 2010, 12, 2912-2915, DOI: 10.1021/ol101098x

InChIs

1: InChI=1/C59H78O7/c1-16-19-64-53-36-22-35-32-47(59(13,14)15)50(51-48(61)33-45(60)34-49(51)62)46(52(35)63)31-41-30-44(58(10,11)12)29-40(55(41)66-21-18-3)24-39-28-43(57(7,8)9)27-38(54(39)65-20-17-2)23-37(53)26-42(25-36)56(4,5)6/h25-30,32-34,60-63H,16-24,31H2,1-15H3
InChIKey=VGWOVZXMYMCPQO-UHFFFAOYAS

Since Heilbronner¹ proposed the Möbius annulene in 1964, organic chemists have been fascinated with this structure and many have tried to synthesize an example. I have written many blog posts (1, 2, 3, 4, 5) related to computed Möbius compounds. Now, Herges and Grimme and co-workers have looked at cationic Möbius annulenes.

For the [9]annulene cation,² a variety of DFT methods, along with SCS-MP2 and CCSSD(T) computations suggest that the lowest energy Hückel (1h) and Möbius (1m) structures, shown in Figure 1, are very close in energy. In fact, the best estimate (CCSD(T)/CBS) is that they differ by only 0.04 kcal mol^-1. Laser flash photolysis of 9-chlorobicyclo[6.1.0]nona-2,4,6-triene suggest however that only the Hückel structure is formed, and that its short lifetime is due to rapid electrocyclic ring closure.

In a follow-up study, Herges has examined the larger annulene cations, specifically [13]-, [17]- and [21]-annulenes. ³ The Möbius form of [13]-annulene cation (2m) is predicted to be 11.0 kcal mol^-1 lower in energy that the Hückel (2h) form at B3LYP/6-311+G**. The structures of these two cations are shown in Figure 1. The Möbius cation 2m is likely aromatic, having NICS(0)= -8.95. Electrocyclic ring closure of 2m requires passing through a barrier of at least 20 kcal mol^-1, suggesting that 2m is a realistic target for preparation and characterization.

1h	1m
2h	2m

Figure 1. Optimized structures of 1 (CCSD(T)/cc-pVTZ)² and 2 (B3LYP/6-311+G**)³.

The energy difference between the Möbius and Hückel structures of the larger annulenes is very dependent on computational method, but in all cases the difference is small. Thus, Herges concludes that [13]-annulene cation should be the sole target of synthetic effort toward identification of a Möbius annulene. Experimental studies are eagerly awaited!

References

(1) Heilbronner, E., “Huckel molecular orbitals of Mobius-type conformations of annulenes,” Tetrahedron Lett., 1964, 5, 1923-1928, DOI: 10.1016/S0040-4039(01)89474-0.

2) Bucher, G.; Grimme, S.; Huenerbein, R.; Auer, A. A.; Mucke, E.; Köhler, F.; Siegwarth, J.; Herges, R., "Is the [9]Annulene Cation a Möbius Annulene?," Angew. Chem. Int. Ed., 2009, 48, 9971-9974, DOI: http://dx.doi.org/10.1002/anie.200900886

(3) Mucke, E.-K.; Kohler, F.; Herges, R., "The [13]Annulene Cation Is a Stable Mobius Annulene Cation," Org. Lett., 2010, 12, 1708–1711, DOI: 10.1021/ol1002384

InChIs

1: InChI=1/C9H9/c1-2-4-6-8-9-7-5-3-1/h1-9H/q+1/b2-1-,5-3-,6-4-,9-7-
InChIKey=LIUDWUIEJKKGNI-BWYSQNKRBF

2: InChI=1/C13H13/c1-2-4-6-8-10-12-13-11-9-7-5-3-1/h1-13H/q+1/b2-1-,5-3-,6-4-,9-7-,10-8-,13-11-
InChIKey=FUBPZYTZTJGXKZ-OGBOFXOGBR

The nature of reactions of indolynes is the subject of two recent computational/experimental studies. There are three isomeric indolynes 1a-c which are analogues of the more famous benzyne (which I discuss in significant detail in Chapter 4.4 of my book).

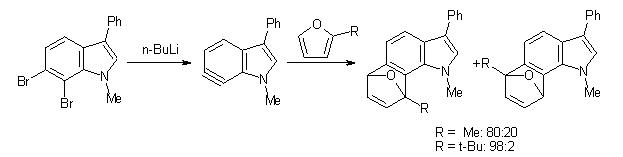
One might anticipate that the indolynes undergo comparable reactions as benzyne, like Diels-Alder reactions and nucleophilic attack. In fact the indolynes do undergo these reactions, with unusual regiospecificity. For example, the reaction of the substituted 6,7-indolyne undergoes regioselective Diels-Alder cycloaddition with substituted furans (Scheme 1), but the reaction with the other indolynes gives no regioselection. ¹ Note that the preferred product is the more sterically congested adduct.

Scheme 1

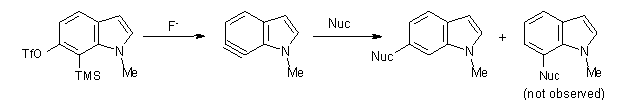
In the case of nucleophilic addition, the nucleophiles add specifically to C₆ with substituted 6,7-indolynes (Scheme 2), while addition to 4,5-indolynes preferentially gives the C₅-adduct (greater than 3:1) while addition to the 5,6-indolynes preferentially gives the C₅-adduct), but with small selectivity (less than 3:1).²

Scheme 2

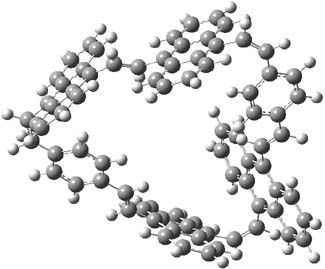
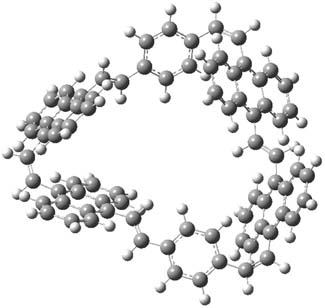
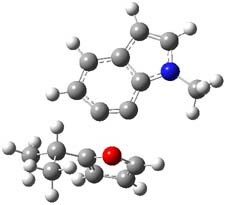
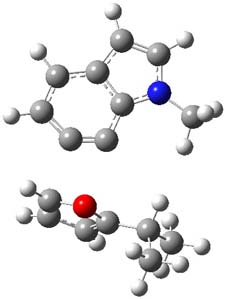
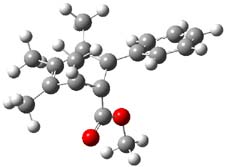
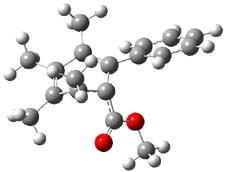
The authors of both papers – Chris Cramer studied the Diels-Alder chemistry and Ken Houk studied the nucleophilic reactions – employed DFT computations to examine the activation barriers leading to the two regioisomeric products. So for example, Figure 1 shows the two transition states for the reaction of 2c with 2-iso-propyl furan computed at MO6-2X/6-311+G(2df,p).

ΔG‡ = 9.7

ΔG‡ = 7.6

Figure 1. MO6-21/6-311+G(2df,p) optimized TSs for the reaction of 2-iso-propylfuran with 2c. Activation energy (kcal mol^-1) listed below each structure.¹

The computational results are completely consistent with the experiments. For the Diels-Alder reaction of 2-t-butylfuran with the three indolynes 2a-c, the lower computed TS always corresponds with the experimentally observed major product. The difference in the energy of the TSs leading to the two regioisomers for reaction with 2a and 2b is small (less than 1 kcal mol^-1), consistent with the small selectivity. On the other hand, no barrier could be found for the reaction of 2-t-butylfuran with 2c that leads to the major product. Similar results are also obtained for the nucleophilic addition – in all cases, the experimentally observed major product corresponds with the lower computed activation barrier.

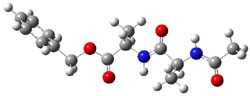
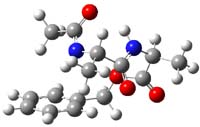
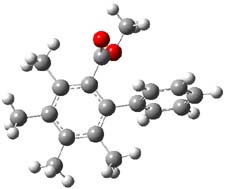
So what accounts for the regioselectivity? Both papers make the same argument, though couched in slightly different terms. Houk argues in terms of distortion energy – the energy needed to distort reactants to their geometries in the TS. As seen in Figure 2, the benzyne fragment of 2a is distorted, with the C-C-C angle at C₄ of 125° and at C₅ of 129°. In the transition states, the angle at the point of nucleophilic attack widens. Since the angle starts out wider at C₅, attack there is preferred, since less distortion is needed to achieve the geometry of the TS.

2a
TS at C4 ΔG‡ = 12.9	TS at C5 ΔG‡ = 9.9

Figure 2. B3LYP/6-31G(d) optimized structures of 2a and the TSs for the reaction of aniline with 2a. Activation energy in kcal mol^-1.²

Cramer argues in terms of the indolyne acting as an electrophile. Increasing substitution at the furan 2-position makes is better at stabilizing incipient positive charge that will build up there during a (very) asymmetric Diels-Alder transition state. This explains the increasing selectivity of the furan with increasing substitution. The indolyne acting as an electrophile means that the attack will lead from the center will lesser charge. In 2c, the C-C-C angle at C₆ is 135.3°, while that at C₇ is 117.2°. This makes C₇ more carbanionic and C₆ more carbocationic; therefore, the first bond made is to C₆, leading to the more sterically congested product. Note that Houk’s argument applies equally well, as C₆ is predistorted to the TS geometry.

References

(1) Garr, A. N.; Luo, D.; Brown, N.; Cramer, C. J.; Buszek, K. R.; VanderVelde, D., "Experimental and Theoretical Investigations into the Unusual Regioselectivity of 4,5-, 5,6-, and 6,7-Indole Aryne Cycloadditions," Org. Lett., 2010, 12, 96-99, DOI: 10.1021/ol902415s

(2) Cheong, P. H. Y.; Paton, R. S.; Bronner, S. M.; Im, G. Y. J.; Garg, N. K.; Houk, K. N., "Indolyne and Aryne Distortions and Nucleophilic Regioselectivites," J. Am. Chem. Soc., 2010, 132, 1267-1269, DOI: 10.1021/ja9098643

InChIs

1a: InChI=1/C8H5N/c1-2-4-8-7(3-1)5-6-9-8/h2,4-6,9H
InChIKey=RNDHGGYOIRREHC-UHFFFAOYAU

1b: InChI=1/C8H5N/c1-2-4-8-7(3-1)5-6-9-8/h3-6,9H
InChIKey=WWZQFJXNXMIWCD-UHFFFAOYAO

1c: InChI=1/C8H5N/c1-2-4-8-7(3-1)5-6-9-8/h1,3,5-6,9H
InChIKey=UHIRLIIPIXHWLT-UHFFFAOYAH

2a: InChI=1/C9H7N/c1-10-7-6-8-4-2-3-5-9(8)10/h3,5-7H,1H3
InChIKey=VTVUPAJGRVFCKI-UHFFFAOYAJ

2b: InChI=1/C9H7N/c1-10-7-6-8-4-2-3-5-9(8)10/h4-7H,1H3
InChIKey=KKPOWDDYMOXTFW-UHFFFAOYAN

2c: InChI=1/C9H7N/c1-10-7-6-8-4-2-3-5-9(8)10/h2,4,6-7H,1H3
InChIKey=MDAHOGWZOBLIEX-UHFFFAOYAZ

Here’s another attempt (almost successful!) in creating a planar cyclooctatetraene. Nishininaga and Iyoda have fused silicon and sulfur bridges to the COT framework, hoping to force the 8-member ring out of its preferred tub-shape into a planar structure.¹ They report the synthesis of 1, 2, and 3b along with their x-ray structures. They also calculated the structures at B3LYP/6-31G(d,p) for 1-4 , and these optimized structures are shown in Figure 1.

1 18° 19°	2 3.0° 4.3°
3a 7.0° (for 3b) 3.2° (for 3a)	4 39° 40°

Figure 1. B3LYP/6-31G(d,p) optimized geometries of 1-4. The experimental (top) and computed (Bottom in italics) value of α are listed for each compound.¹

The bent angle α is defined at the angle between the two planes that define the bottom of the tub and one of the sides. For COT itself, this angle is 40°, decidedly non-planar – as expected for a molecule avoiding the antiaromatic character it would have in its planar conformation. The computed and experimental values of α are shown in Figure 1. 4 is tub shaped. The value of α for 1 is about 18° – still tub shaped but flattened. But 2 and 3 are nearly planar, with experimental values of α about 3° and the computed values are similar.

So what is the character of the 8-member ring in these compounds. The computed NICS(0) values are 3.8 ppm for 4, the expected small value for a non-aromatic compound. (Note that the NICS value for COT is 2.9 ppm.) The values are much more positive for the other compounds: 12.7 ppm for 1, 17.4 ppm for 2, and 15.4 ppm for 3a. These compounds therefore display antiaromatic character yet they are isolable compounds!

References

(1) Ohmae, T.; Nishinaga, T.; Wu, M.; Iyoda, M., "Cyclic Tetrathiophenes Planarized by Silicon and Sulfur Bridges Bearing Antiaromatic Cyclooctatetraene Core: Syntheses, Structures, and Properties," J. Am. Chem. Soc., 2009, 132, 1066-1074, DOI: 10.1021/ja908161r

InChIs

1: InChI=1/C20H16S4Si2/c1-25(2)9-5-21-17-13(9)14-10(25)6-22-18(14)20-16-12(8-24-20)26(3,4)11-7-23-19(17)15(11)16/h5-8H,1-4H3/b19-17-,20-18-
InChIKey=DAVVQYAXJVCICC-CLFAGFIQBV

2: InChIKey=PBXVOLKKILUEGI-RFIZXXDFBX

3a: InChI=1/C16H4O4S6/c17-25(18)5-1-21-13-9(5)10-6(25)2-23-15(10)16-12-8(4-24-16)26(19,20)7-3-22-14(13)11(7)12/h1-4H/b14-13-,16-15-
InChIKey=VIDZCGPUBZEACC-RFIZXXDFBR

3b: InChI=1/C28H36O4S6Si4/c1-39(2,3)25-21-13-14-19(35-26(40(4,5)6)22(14)37(21,29)30)20-16-15-18(17(13)33-25)34-27(41(7,8)9)23(15)38(31,32)24(16)28(36-20)42(10,11)12/h1-12H3/b18-17-,20-19-
InChIKey=XXCFCYWSFICMIO-RXGVRZIVBS

4: InChI=1/C16H8S4/c1-5-17-13-9(1)10-2-6-18-14(10)16-12(4-8-20-16)11-3-7-19-15(11)13/h1-8H/b10-9-,12-11-,15-13-,16-14-
InChIKey=RSNUTSCZGMAXQJ-FNJUYVFOBD

Dewar benzene has fascinated physical organic chemists for a long time. Just how does this open up? And why is is stable, given its large strain and the aromaticity of the ring-opened product? Most had rationalized this by recognizing that the route that takes Dewar benzene into benzene is the symmetry-forbidden disrotatory path, and the symmetry allowed conrotatory path leads to the benzene with a trans double bond.

Johnson¹ discovered that the conrotatory TS is high in energy, but below the C_2v structure thought to be the disrotatory TS, but is in fact a saddle point. Further, the IRC path through the conrotatory TS connects Dewar benzene to benzene, avoiding the trans-benzene (which is sometimes referred to as Möbius benzene).

Now comes a report that the PES for opening of Dewar benzene is a bit more complicated.² B3LYP/6-311+G** computations of 1 going to 3 identifies not only the Möat;bius benzene intermediate 2 but a transition state connecting 1 to 2 and a second transition state that connects 2 with 3. These structures are shown in Figure 1.

1	TS1
2	TS2
3

Figure 1. B3LYP/6-311+G** optimized geometries of 1-3 and the two TSs.²

The first TS is 31.0 kcal mol^-1 above reactant and the Möbius benzene intermediate is only 2.1 kcal mol^-1 below this TS. The second TS is 1.6 kcal mol^-1 higher than the first TS, and so is rate-limiting.

The authors also examined a series of related Dewar benzenes and all have two TSs, with the second always higher than the first.

Molecular dynamics computations suggest that the Möbius benzene is likely avoided during the reaction. The fact that the two TSs are similar in energy disguised the fact that there is a second one – the energy of the first TS matches up nicely with experiments. Further MD studies would be interesting to see the interplay between the geometrically quite close intermediate and two TSs – some novel dynamics might be at work here.

References

(1) Johnson, R. P.; Daoust, K. J., "Electrocyclic Ring Opening Modes of Dewar Benzenes: Ab Initio Predictions for Möbius Benzene and trans-Dewar Benzene as New C₆H₆ Isomers," J. Am. Chem. Soc., 1996, 118, 7381-7385, DOI: 10.1021/ja961066q

(2) Dracinsky, M.; Castano, O.; Kotora, M.; Bour, P., "Rearrangement of Dewar Benzene Derivatives Studied by DFT," J. Org. Chem. 2010, ASAP, DOI: 10.1021/jo902065n

InChIs

1: InChI=1/C18H20O2/c1-11-12(2)18(4)15(16(19)20-5)14(17(11,18)3)13-9-7-6-8-10-13/h6-10H,1-5H3
InChIKey=LGAXKHBTTKUURV-UHFFFAOYAH

3: InChI=1/C18H20O2/c1-11-12(2)14(4)17(18(19)20-5)16(13(11)3)15-9-7-6-8-10-15/h6-10H,1-5H3
InChIKey=OUPUSCZNPIJJAH-UHFFFAOYAO

The longstanding unknown oxyallyl diradical (1) singlet-triplet gap has now been addressed with a very nice photoelectron spectroscopy study by Lineberger with interpretation greatly aided by computations provided by Hrovat and Borden.¹

The photoelectron detachment spectrum of oxyallyl radical anion shows 5 major peaks, one at 1.942 eV and a series of four peaks starting at 1.997 eV separated by 405 cm^-1.

B3LYP/6-311++G(d,p) computations indicate that the energy for electron detachment from the radical anion to triplet oxyallyl diradical is 1.979 eV. (The structure of triplet 1 is shown in Figure 1.) Further, the computed vibrational frequency of the C-C-C bend is 408 cm^-1. These computations suggest that the four peak sequence represents a vibrational progression in the C-C-C bend of the triplet oxyallyl diradical.

1A1

3B2

Figure 1. Structures of the singlet and triplet oxyallyl diradical 1.¹

CASPT2 computations on singlet oxyallayl diradical indicate that it lies in a very shallow well, lower than the zero-point energy. (This structure is shown in Figure 1.) In fact, the singlet diradical can collapse without a barrier to cyclopropanone. Interestingly, the C-O stretching frequency of 1 is computed to be 1731 cm^-1, and close inspection of the photoelectron spectrum does show a progression of this magnitude originating from peak A. Therefore, both the singlet and triplet states of 1 are identified and their gap is extraordinarily small – the singlet is only 0.055 eV lower in energy than the triplet.

References

(1) Ichino, T.; Villano, S. M.; Gianola, A. J.; Goebbert, D. J.; Velarde, L.; Sanov, A.; Blanksby, S. J.; Zhou, X.; Hrovat, D. A.; Borden, W. T.; Lineberger, W. C., "The Lowest Singlet and Triplet States of the Oxyallyl Diradical," Angew. Chem. Int. Ed., 2009, 48, 8509-8511, DOI: 10.1002/anie.200904417

Does the Carbon-Sulfur triple bond exist? There’s probably little doubt it does in the CS molecule. But now Schreiner and Mloston have offered up the H-C≡S-OH species as a possibility.¹ Obtained by flash photolysis of 1, giving 2, and upon irradiation at 254 nm, H-C≡S-OH 3 is the observed species and not the expected carbene HO-C-SH 4. 3 is confirmed by excellent agreement between the observed and computationally predicted IR spectra.

The CCSD(T)/cc-pVTZ structures of 3 and 4 are shown in Figure 1. It is interesting that the carbene is not observed, even though it is 26.6 kcal mol^-1 more stable than 3.

3

4

Figure 1. CCSD(T)/cc-PVTZ optimized structures of 3 and 4.¹

So is there a triple bond? The short C-S distance (1.547 Å) is very similar to that in CS (1.545 Å). NBO analysis indicates a triple bond. But the MOs indicate significant lone pair build-up on both C and S, consistent with the strongly non-linear angles about these two atoms. The authors conclude that 3 is a “structure with a rather strong CS double bond or a weak triple bond”.

References

(1) Schreiner, P. R.; Reisenauer, H. P.; Romanski, J.; Mloston, G., "A Formal Carbon-Sulfur Triple Bond: H-C≡S-O-H," Angew. Chem. Int. Ed., 2009, 48, 8133-8136, DOI: 10.1002/anie.200903969