I have written many posts on the use of computed NMR shifts as a tool for determining molecular structure, especially stereochemistry. All of these methods rely upon computing a bunch of alternative structures and then identifying the one whose chemical shifts (¹H and/or ¹³C) match up best with experiment. Many people have been interested in the first part of this process – the “computing a bunch of alternative structures” – testing the QM method, the basis set, the selection of conformation(s), and the method for computing chemical shifts. The subject of this post is the notion of “matching up best” and comes from of a recent article by Jonathan Goodman.¹

So in the typical procedure for deciding which structure (of many) best accounts for the experimental NMR spectra, the computed NMR shifts (and perhaps coupling constants) are compared to the experimental data. This comparison is done often by simply examining the correlation coefficient r between the experimental and calculated shifts. Some have used the mean absolute error between the computed and experimental shifts. Others have employed a corrected mean absolute error where scaled chemical shifts are first obtained from the plot of the calculated vs. experimental shifts, and then finding the average of the differences between these scaled shifts and the experimental ones.

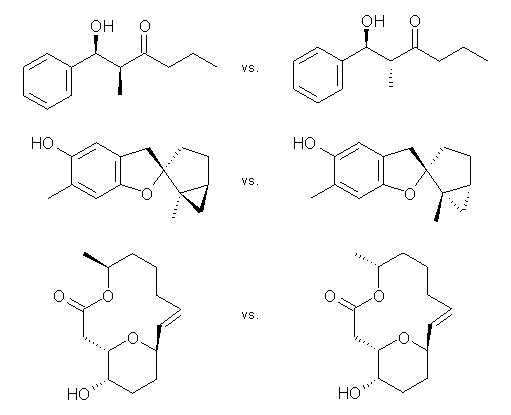
Goodman suggests that oftentimes what is of interest is not really the chemical shifts of a compound but rather identifying the structure of diastereomers, and then it’s really the differences in the chemical shifts of pairs of diastereomers that are really critical in identifying which one is which. Using Goodman’s notation, suppose you have experimental NMR data on diastereomers A and B and the computed NMR shifts for structures a and b. The key is deciding does A correlate with a or b and the same for B. Goodman proposes three variants on how to compare the chemical shift differences, but I’ll show just the first, which he calls CP1. Define Δ_expⁱ as the differences in the experimental chemical shifts of the two diastereomers for nucleus i: Δ_exp = δ_Aⁱ – δ_Bⁱ and a similar definition for the differences in the computed shifts: Δ_calc = δ_aⁱ – δ_bⁱ. CP1 is then defined as Σ (Δ_exp/Δ_calc)/Σ (Δ_exp)² where each sum is over the nuclei i. Goodman shows in a number of examples (some are shown below) that CP1 and its variants provides an excellent measure of when a computed structure’s chemical shifts agree with the experimental values, along with a means for noting the confidence in that assignment. These CP measures provide significantly better measures of agreement that the ones previous utilized, providing a real confidence level in assessing the quality of the prediction. I strongly urge all who are interested in the use of computed NMR in determining molecular structures to read this paper and consider adopting this approach.

References

(1) Smith, S. G.; Goodman, J. M., "Assigning the Stereochemistry of Pairs of Diastereoisomers Using GIAO NMR Shift Calculation," J. Org. Chem. 2009, 74, 4597-4607, DOI: 10.1021/jo900408d

Conjugated alkenes have played a major role in conceptualizing organic chemistry. Linear and cyclic unbranched conjugated alkenes have been well studied; the latter class comprising the aromatic and antiaromatic annulenes. The cyclic branched conjugate alkenes are known as radialenes and have been subject of some study. But the last category, the linear branched conjugated alkenes have been overlooked. Paddon-Row and Sherburn¹ now report a general synthetic method for preparing these species, which they call dendralenes, see Scheme 1.

Scheme 1. Classes of conjugated alkenes

The dendralenes fall into two groups – those with an odd number of double bonds and those with an even number. While the UV/Vis absorption maximum redshifts with increasing length, the molar extinctions coefficients are relatively constant for the odd denralenes but it increases by about 10,000 within the even dendralene family. The Diels-Alder chemistry is even more distinctive: the odd dendralenes react rapidly with an electron deficient dienophile (N-methylmaleimide), with rates decreasing slightly with increasing size, but the even dendralenes are significantly more sluggish.

The optimized B3LYP/6-31G(d) geometries of the lowest energy conformers of the [3]- to [8]dendralenes are shown in Figure 1. There are three types of butadiene fragments present in these structures: (a) near planar s-trans arrangement, (b) near perpendicular arrangement of the two double bonds, and (c) ­s-cis arrangement with the dihedral angle about 40°. The even dendralenes have only the first two type: alternating planar butadiene fragment that are more-or-less orthogonal to each other. The odd dendralenes all have at least one s-cis arrangement. Paddon-Row and Sherburn suggest that since the s-cis arrangement is necessary for the diene component of the Diels-SAlder reaction, the odd dendralenes are more reactive than the even ones since they have this arranegement in their ground state conformations, while the even dendralenes will have to react out of a higher energy conformation. This is a nice explanation readily formulated from simple computations.

[3]dendralene	[4]dendralene
[5]dendralene	[6]dendralene
[7]dendralene	[8]dendralene

Figure 1. B3LYP/6-31G(d) optimized structures of [3]- to [8]dendralene.¹

References

(1) Payne, A. D.; Bojase, G.; Paddon-Row, M. N.; Sherburn, M. S., "Practical Synthesis of the Dendralene Family Reveals Alternation in Behavior," Angew. Chem. Int. Ed. 2009, 48, 4836-4839, DOI: 10.1002/anie.200901733

InChIs

[3]dendralene: InChI=1/C6H8/c1-4-6(3)5-2/h4-5H,1-3H2
InChIKey=VXBVLYQDVVHAHZ-UHFFFAOYAS

[4]dendralene: InChI=1/C8H10/c1-5-7(3)8(4)6-2/h5-6H,1-4H2
InChIKey=DMCINEDFOKMBFI-UHFFFAOYAV

[5]dendralene: InChI=1/C10H12/c1-6-8(3)10(5)9(4)7-2/h6-7H,1-5H2
InChIKey=XEZCEXNNZGLEHB-UHFFFAOYAM

[6]dendralene: InChI=1/C12H14/c1-7-9(3)11(5)12(6)10(4)8-2/h7-8H,1-6H2
InChIKey=RBABOPLFRQKABD-UHFFFAOYAA

[7]dendralene: InChI=1/C14H16/c1-8-10(3)12(5)14(7)13(6)11(4)9-2/h8-9H,1-7H2
InChIKey=ZIBYAXDRKFGSBF-UHFFFAOYAH

[8]dendralene: InChI=1/C16H18/c1-9-11(3)13(5)15(7)16(8)14(6)12(4)10-2/h9-10H,1-8H2
InChIKey=YWPORNAHEZCVCQ-UHFFFAOYAR

Jan Martin and his group at the Weizmann Institute continue to push the envelope in developing a computational rubric that produces computed energies with experimental accuracy. Their latest attempt tries to balance off computational accuracy with performance, and they propose the W3.2lite composite method,¹ which includes, among other things, an empirical correction for including triples and quadruples configurations.

Amongst the test molecules they discuss are the benzynes (the ortho, meta, and para diradicals) discussed at great length in Chapter 4.4 of my book. The W3.2lite estimate heats of formations are 112.06 ± 0.5, 125.06 ± 0.5, and 139.03 ± 0.5 kcal mol^-1 for the o-, m-, and p-benzyne, respectively. This compares with the experimental² estimates of 108.8 ± 3, 124.1 ± 3.1, and 139.5 ± 3.3 kcal mol^-1, respectively. This demonstrates nice agreement between the computed and experimental values. A similar sized difference is obtained for the singlet-triplet gap of p-benzyne: 5.4 ± 0.6 with W3.2lite and 3.8 ± 0.5 kcal mol^-1 estimate from ultraviolet photoelectron spectroscopy.³

References

(1) Karton, A.; Kaminker, I.; Martin, J. M. L., "Economical Post-CCSD(T) Computational Thermochemistry Protocol and Applications to Some Aromatic Compounds," J. Phys. Chem. A 2009, DOI: 10.1021/jp900056w.

(2) Wenthold, P. G.; Squires, R. R., "Biradical Thermochemistry from Collision-Induced Dissociation Threshold Energy Measurements. Absolute Heats of Formation of ortho-, meta-, and para-Benzyne," J. Am. Chem. Soc. 1994, 116, 6401-6412, DOI: 10.1021/ja00093a047.

(3) Wenthold, P. G.; Squires, R. R.; Lineberger, W. C., "Ultraviolet Photoelectron Spectroscopy of the o-, m-, and p-Benzyne Negative Ions. Electron Affinities and Singlet-Triplet Splittings for o-, m-, and p-Benzyne," J. Am. Chem. Soc. 1998, 120, 5279-5290, DOI: 10.1021/ja9803355.

InChIs

o-benzyne: InChI=1/C6H4/c1-2-4-6-5-3-1/h1-4H
InChIKey=KLYCPFXDDDMZNQ-UHFFFAOYAO

m-benzyne: InChI=1/C6H4/c1-2-4-6-5-3-1/h1-3,6H
InChIKey=MDEXXEPHAKMVNO-UHFFFAOYAG

p-benzyne: InChI=1/C6H4/c1-2-4-6-5-3-1/h1-2,5-6H
InChIKey=AIESRBVWAFETPR-UHFFFAOYAI