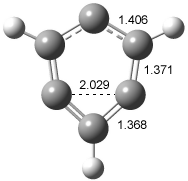
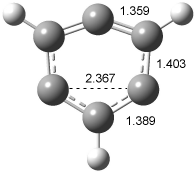
I have been delinquent in writing about the dehydro-Diels-Alder reactions, but really can’t put it off any further. These sets of reactions really deserve a fuller analysis than I am going to summarize here, but this post will provide a good jumping off point for anyone interested in further investigation.

So the Diels-Alder reaction is among the most famous and most important reactions in organic chemistry. The reaction creates a 6-member ring and sets up to four stereocenters. In the past couple of years many chemists have expressed interest in the variant where the four-carbon component is more highly unsaturated, i.e. enyne or diyne. I will summarize the results of three recent computational papers dealing with the reaction of a diyne with an yne.

The first paper is by Skraba-Joiner, Johnson, and Agarwal.¹ They discuss, among a number of interesting pericyclic reactions, the intramolecular Diels-Alder reaction of triyne 1 to give 2. They examined a concerted and stepwise pathway at (U)M05-2X/6-311+G(d,p) and find the concerted to be favored by 6.0 kcal mol^-1. CCSD(T) using these geometries increases the difference to 8.2 kcal mol^-1. The T₁ diagnostic is fairly large for both the concerted and stepwise transition states, so they also performed CCSD(T)/CBS computations, which had much lower T₁ values. The concerted TS remained favorable, but by only 2.7 kcal mol^-1.

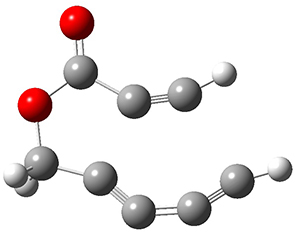
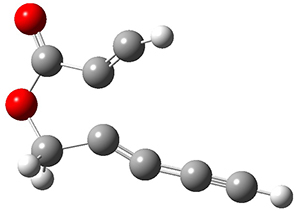
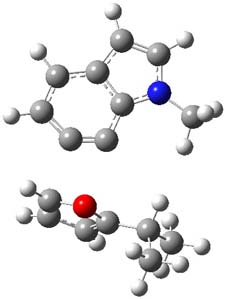
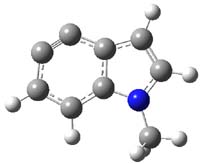
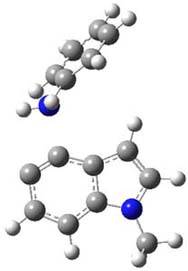
In the same special issue of the Journal of Organic Chemistry, Cramer, Hoye, and Kuwata examined a reaction closely related to what Johnson examined above.² They looked at the reaction taking 3 into 4 via both experiments and computations. The M06-2x/6-311+G(d,p) geometries for the concerted and first TS along the stepwise path (with R1=R2=H) are shown in Figure 1. Evaluating the energies at SMD(o-dichlorobenzene)/B3LYP-D3BJ/6-311+G-(d,p)//M06-2X/6-311+G(d,p) find in this case (along with all of the other R1/R2 variants they examined) that the stepwise path has a lower barrier than the concerted path. In the case where R1=R2=H, the stepwise path is favored by 6.0 kcal mol^-1. Additionally, these stepwise barriers are in reasonable agreement with the experimentally-derived barriers.

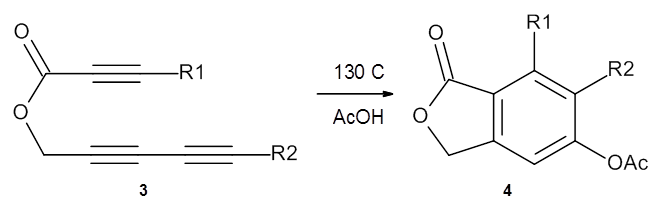
Concerted TS

Stepwise TS

Figure 1. M06-2x/6-311+G(d,p) optimized geometries of the concerted and stepwise TSs for the reaction of 3H going to 4H.

It should be pointed out that the wavefunctions for the concerted TSs were all found to be unstable with regard to a restricted to unrestricted relaxation. Given this problem, they also performed a CASPT2 energy evaluation of the concerted and stepwise transition states for the case R1=R2=H. CASPT2 finds the stepwise barrier to be 3.7 kcal mol^-1 lower than the concerted barrier.

The last paper comes from the Houk lab, and examines the simplest set of intermolecular dehdro-Diels-Alder reactions.³ I will focus here on the most unsaturated analogue, the reaction of 1,3-butadiyne 5 with ethyne to give benzyne 6.

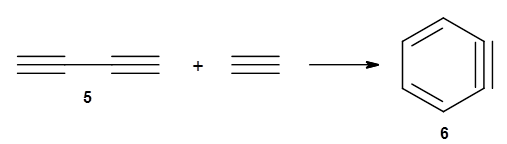
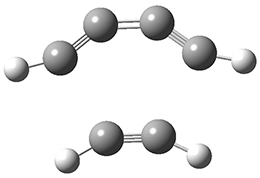
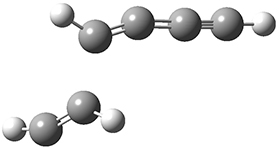
The concreted and stepwise transition states for this reaction (at (U)M06-2X/6-311+G(d,p)) are shown in Figure 2. The concerted barrier is 36.0 kcal moml^-1 while the stepwise barrier is slightly lower: 35.2 kcal mol^-1. The distortion energy for the concerted reaction is large (43.2 kcal mol^-1) due mostly to angle changes in the diyne. Its interaction energy is -7.2 kcal mol^-1, similar to the interaction energy in other similar Diels-Alder reactions. In contrast, the distortion energy for the stepwise pathway is 27.5 kcal mol^-1, but the interaction energy is +7.7 kcal mol^-1. These values are very similar to the distortion and interaction energy of the related (but less saturated DA reactions).

Concerted TS

Stepwise TS

Figure 2. (U)M06-2X/6-311+G(d,p) optimized concerted and stepwise TS for the reaction of 1,3-diyne with ethyne.

Molecular dynamics trajectories for both the concerted and stepwise paths reveal interesting differences. The concerted trajectories show an oscillatory behaviour of bending the angles at the C2 and C3 carbons prior to the TS, and then near synchronous formation of the new C-C bonds. The trajectories initiated at the stepwise TS show no systematic motion. Once the bond is formed, the biradical exhibits a long lifetime, on the order of picoseconds, much longer than the trajectory runs.

These three studies indicate the nature of the dehydro Diels-Alder reaction is very sensitive to reaction conditions, substituents, solvation, and all other manner of effects and will likely prove an area of interest for some time. It should keep a number of computational chemists busy for some time!

References

(1) Skraba-Joiner, S. L.; Johnson, R. P.; Agarwal, J. "Dehydropericyclic Reactions: Symmetry-Controlled Routes to Strained Reactive Intermediates," J. Org. Chem. 2015, 80, 11779-11787, DOI: 10.1021/acs.joc.5b01488.

(2) Marell, D. J.; Furan, L. R.; Woods, B. P.; Lei, X.; Bendelsmith, A. J.; Cramer, C. J.; Hoye, T. R.; Kuwata, K. T. "Mechanism of the Intramolecular Hexadehydro-Diels–Alder Reaction," J. Org. Chem. 2015, 80, 11744-11754, DOI: 10.1021/acs.joc.5b01356.

(3) Yu, P.; Yang, Z.; Liang, Y.; Hong, X.; Li, Y.; Houk, K. N. "Distortion-Controlled Reactivity and Molecular Dynamics of Dehydro-Diels–Alder Reactions," J. Am. Chem. Soc. 2016, 138, 8247-8252, DOI: 10.1021/jacs.6b04113.

InChIs

1: InChI=1S/C9H8/c1-3-5-7-9-8-6-4-2/h1-2H,5,7,9H2
InChIKey=IYZAZSVBWMMSLQ-UHFFFAOYSA-N

2: InChI=1S/C9H8/c1-2-5-9-7-3-6-8(9)4-1/h1,4H,3,6-7H2
InChIKey=PZJMTUKDGZUDBH-UHFFFAOYSA-N

3H: InChI=1S/C8H4O2/c1-3-5-6-7-10-8(9)4-2/h1-2H,7H2
InChIKey=MGXDIFXPYGGQLF-UHFFFAOYSA-N

4H: InChI=1S/C10H8O4/c1-6(11)14-8-2-3-9-7(4-8)5-13-10(9)12/h2-4H,5H2,1H3
InChIKey=GEFLHLNIKGXWCA-UHFFFAOYSA-N

5: InChI=1S/C4H2/c1-3-4-2/h1-2H
InChIKey=LLCSWKVOHICRDD-UHFFFAOYSA-N

6: InChI=1S/C6H4/c1-2-4-6-5-3-1/h1-4H
InChIKey=KLYCPFXDDDMZNQ-UHFFFAOYSA-N

I want to update my discussion of m-benzyne, which I present in my book in Chapter 5.5.3. The interesting question concerning m-benzyne concerns its structure: is it a single ring structure 1a or a bicyclic structure 1b? Single configuration methods including closed-shell DFT methods predict the bicylic structure, but multi-configuration methods and unrestricted DFT predict it to be 1a. Experiments support the single ring structure 1a.

The key measurement distinguishing these two structure type is the C₁-C₃ distance. Table 1 updates Table 5.11 from my book with the computed value of this distance using some new methods. In particular, the state-specific multireference coupled cluster Mk-MRCCSD method¹ with the cc-pCVTZ basis set indicates a distance of 2.014 Å.² The density cumulant functional theory³ ODC-12⁴ with the cc-pCVTZ basis set also predicts the single ring structure with a distance of 2.101 Å.⁵

Table 1. C₁-C₃ distance (Å) with different computational methods using the cc-pCVTZ basis set

References

(1) Evangelista, F. A.; Allen, W. D.; Schaefer III, H. F. "Coupling term derivation and general implementation of state-specific multireference coupled cluster theories," J. Chem. Phys 2007, 127, 024102-024117, DOI: 10.1063/1.2743014.

(2) Jagau, T.-C.; Prochnow, E.; Evangelista, F. A.; Gauss, J. "Analytic gradients for Mukherjee’s multireference coupled-cluster method using two-configurational self-consistent-field orbitals," J. Chem. Phys. 2010, 132, 144110, DOI: 10.1063/1.3370847.

(3) Kutzelnigg, W. "Density-cumulant functional theory," J. Chem. Phys. 2006, 125, 171101, DOI: 10.1063/1.2387955.

(4) Sokolov, A. Y.; Schaefer, H. F. "Orbital-optimized density cumulant functional theory," J. Chem. Phys. 2013, 139, 204110, DOI: 10.1063/1.4833138.

(5) Mullinax, J. W.; Sokolov, A. Y.; Schaefer, H. F. "Can Density Cumulant Functional Theory Describe Static Correlation Effects?," J. Chem. Theor. Comput. 2015, 11, 2487-2495, DOI: 10.1021/acs.jctc.5b00346.

InChIs

1a: InChI=1S/C6H4/c1-2-4-6-5-3-1/h1-3,6H

The nature of reactions of indolynes is the subject of two recent computational/experimental studies. There are three isomeric indolynes 1a-c which are analogues of the more famous benzyne (which I discuss in significant detail in Chapter 4.4 of my book).

One might anticipate that the indolynes undergo comparable reactions as benzyne, like Diels-Alder reactions and nucleophilic attack. In fact the indolynes do undergo these reactions, with unusual regiospecificity. For example, the reaction of the substituted 6,7-indolyne undergoes regioselective Diels-Alder cycloaddition with substituted furans (Scheme 1), but the reaction with the other indolynes gives no regioselection. ¹ Note that the preferred product is the more sterically congested adduct.

Scheme 1

In the case of nucleophilic addition, the nucleophiles add specifically to C₆ with substituted 6,7-indolynes (Scheme 2), while addition to 4,5-indolynes preferentially gives the C₅-adduct (greater than 3:1) while addition to the 5,6-indolynes preferentially gives the C₅-adduct), but with small selectivity (less than 3:1).²

Scheme 2

The authors of both papers – Chris Cramer studied the Diels-Alder chemistry and Ken Houk studied the nucleophilic reactions – employed DFT computations to examine the activation barriers leading to the two regioisomeric products. So for example, Figure 1 shows the two transition states for the reaction of 2c with 2-iso-propyl furan computed at MO6-2X/6-311+G(2df,p).

ΔG‡ = 9.7

ΔG‡ = 7.6

Figure 1. MO6-21/6-311+G(2df,p) optimized TSs for the reaction of 2-iso-propylfuran with 2c. Activation energy (kcal mol^-1) listed below each structure.¹

The computational results are completely consistent with the experiments. For the Diels-Alder reaction of 2-t-butylfuran with the three indolynes 2a-c, the lower computed TS always corresponds with the experimentally observed major product. The difference in the energy of the TSs leading to the two regioisomers for reaction with 2a and 2b is small (less than 1 kcal mol^-1), consistent with the small selectivity. On the other hand, no barrier could be found for the reaction of 2-t-butylfuran with 2c that leads to the major product. Similar results are also obtained for the nucleophilic addition – in all cases, the experimentally observed major product corresponds with the lower computed activation barrier.

So what accounts for the regioselectivity? Both papers make the same argument, though couched in slightly different terms. Houk argues in terms of distortion energy – the energy needed to distort reactants to their geometries in the TS. As seen in Figure 2, the benzyne fragment of 2a is distorted, with the C-C-C angle at C₄ of 125° and at C₅ of 129°. In the transition states, the angle at the point of nucleophilic attack widens. Since the angle starts out wider at C₅, attack there is preferred, since less distortion is needed to achieve the geometry of the TS.

2a
TS at C4 ΔG‡ = 12.9	TS at C5 ΔG‡ = 9.9

Figure 2. B3LYP/6-31G(d) optimized structures of 2a and the TSs for the reaction of aniline with 2a. Activation energy in kcal mol^-1.²

Cramer argues in terms of the indolyne acting as an electrophile. Increasing substitution at the furan 2-position makes is better at stabilizing incipient positive charge that will build up there during a (very) asymmetric Diels-Alder transition state. This explains the increasing selectivity of the furan with increasing substitution. The indolyne acting as an electrophile means that the attack will lead from the center will lesser charge. In 2c, the C-C-C angle at C₆ is 135.3°, while that at C₇ is 117.2°. This makes C₇ more carbanionic and C₆ more carbocationic; therefore, the first bond made is to C₆, leading to the more sterically congested product. Note that Houk’s argument applies equally well, as C₆ is predistorted to the TS geometry.

References

(1) Garr, A. N.; Luo, D.; Brown, N.; Cramer, C. J.; Buszek, K. R.; VanderVelde, D., "Experimental and Theoretical Investigations into the Unusual Regioselectivity of 4,5-, 5,6-, and 6,7-Indole Aryne Cycloadditions," Org. Lett., 2010, 12, 96-99, DOI: 10.1021/ol902415s

(2) Cheong, P. H. Y.; Paton, R. S.; Bronner, S. M.; Im, G. Y. J.; Garg, N. K.; Houk, K. N., "Indolyne and Aryne Distortions and Nucleophilic Regioselectivites," J. Am. Chem. Soc., 2010, 132, 1267-1269, DOI: 10.1021/ja9098643

InChIs

1a: InChI=1/C8H5N/c1-2-4-8-7(3-1)5-6-9-8/h2,4-6,9H
InChIKey=RNDHGGYOIRREHC-UHFFFAOYAU

1b: InChI=1/C8H5N/c1-2-4-8-7(3-1)5-6-9-8/h3-6,9H
InChIKey=WWZQFJXNXMIWCD-UHFFFAOYAO

1c: InChI=1/C8H5N/c1-2-4-8-7(3-1)5-6-9-8/h1,3,5-6,9H
InChIKey=UHIRLIIPIXHWLT-UHFFFAOYAH

2a: InChI=1/C9H7N/c1-10-7-6-8-4-2-3-5-9(8)10/h3,5-7H,1H3
InChIKey=VTVUPAJGRVFCKI-UHFFFAOYAJ

2b: InChI=1/C9H7N/c1-10-7-6-8-4-2-3-5-9(8)10/h4-7H,1H3
InChIKey=KKPOWDDYMOXTFW-UHFFFAOYAN

2c: InChI=1/C9H7N/c1-10-7-6-8-4-2-3-5-9(8)10/h2,4,6-7H,1H3
InChIKey=MDAHOGWZOBLIEX-UHFFFAOYAZ

In section 4.4 of the book, I discuss in great detail the computational (and some experimental) studies of the benzynes, the formal diradicals created by loss of two hydrogen atoms from benzene. Now comes a very nice experimental study on a molecule that takes the next step: 1,3,5-tridehydrobenzene 1, benzene that lacks three hydrogen atoms. Sander reports the preparation and characterization of trifluoro-1,3,5-tridehydrobenzene 2.¹ The characterization of this novel molecule is made through comparison with computed IR spectra.

2 is prepared by flash vapor pyrolysis of 1,3,5-triiodo-2,4,6-trifluorobenezene
and then trapping the products in a low temperature matrix. Sander identifies five IR peaks of a product he believes is 2. These IR frequencies are listed in Table 1.

Table 1. Experimental and computed^a IR frequencies (cm^-1) and relative intensities of 2.

^aUBLYP/cc-pVTZ. ^bTransition state.

In order to confirm that this IR spectra comes from 2, Sander computed the structure and IR frequencies of both 1 and 2. The ²A₁ structure of 1 had been studied previously², but what had gone unnoticed is that another structure is possible, the ²B₂ state. These two states differ in the separation between C₁ and C₃. When the distance is short, the SOMO is of a₁ symmetry and this orbital has bonding character between these two carbon centers, giving rise to the ²A₁ state (1a). As the distance gets longer between C₁ and C₃, a b₂ orbital, having antibonding character between C₁and C₃, becomes lower in energy than the a₁ orbital, so that the structure is ²B₂ (1b). The UBLYP/cc-pVTZ optimized structures are shown in Figure 1. 1a is 2-3 kcal mol^-1 lower in energy than 1b. Furthermore, 1b has one imaginary frequency and is not a local energy minimum. Sander also optimized the structures of 2a and 2b¸ finding little effect due to the fluorine substitution.

1a

1b

Figure 1. UBLYP/cc-pVTZ optimized structures of 1a (²A₁) and 1b (²B₁).

The computed IR frequencies are listed in Table 1. The computed frequencies (and their relative intensities) of 2a match up strikingly well with those of the experiment. Sander concludes that 2a has in fact been prepared and characterized.

References

(1) Venkataramani, S.; Winkler, M.; Sander, W., "Trifluoro-1,3,5-tridehydrobenzene," Angew. Chem. Int. Ed. 2007, 46, 4888-4893, DOI: 10.1002/anie.200700536

(2) Cristian, A. M. C.; Shao, Y.; Krylov, A. I., "Bonding Patterns in Benzene Triradicals from Structural, Spectroscopic, and Thermochemical Perspectives," J. Phys. Chem. A 2004, 108, 6581-6588, DOI: 10.1021/jp049007j.

InChI:

1: InChI=1/C6H3/c1-2-4-6-5-3-1/h1,4-5H
2: InChI=1/C6F3/c7-4-1-5(8)3-6(9)2-4