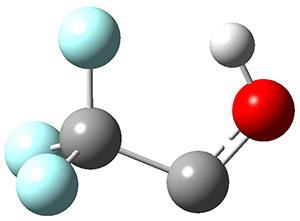
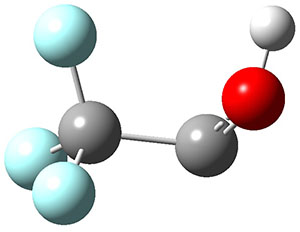
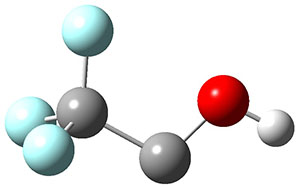
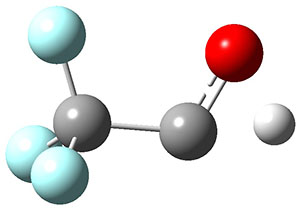
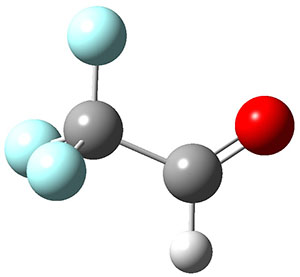
Eckhardt and Schreiner have spectroscopically characterized the aminomethylene carbene 1.¹ Their characterization rests on IR spectra, with comparison to the computed AE-CCSD(T)/cc-pCVQZ anharmonic vibrational frequencies, and the UV-Vis spectra, with comparison to the computed B3LYP/6–311++G(2d,2p) transitions.

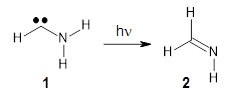
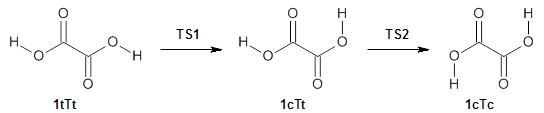
1 can be converted to 2 by photolysis. Interestingly, 1 does not convert to 2 after 5 days on the matrix in the dark. This is in distinct contrast to hydroxycarbene and related other carbene which undergo quantum mechanical tunneling (see this post and this post). Examination of the potential energy surface for the reaction of 1 to 2 at AE-CCSD(T)/cc-pCVQZ (see Figure 1) identifies that the lowest barrier is 45.8 kcal mol^-1, about 15 kcal mol^-1 larger than the barrier for the hydroxycarbene rearrangement. Additionally, the barrier width for 1 → 2 is 25% larger than for the hydroxycarbenes. Both of these suggest substantially reduced tunneling, and WKB analysis predicts a tunneling half-life of more than a billion years. The stability of 1 is attributed to the strong π-donor ability of nitrogen to the electron-poor carbene. This is reflected in a very short C-N bond (1.27 Å).

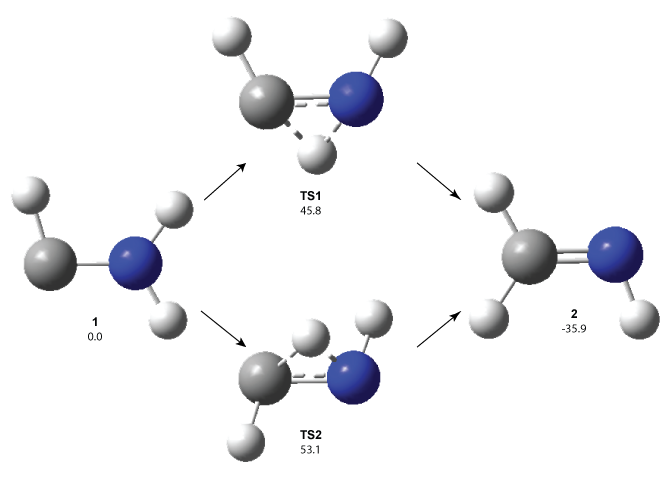
Figure 1. Structures and energies of 1 and 2 and the transition states that connect them. The relative energies (kcal mol^-1) are computed at AE-CCSD(T)/cc-pCVQZ.

References

1) Eckhardt, A. K.; Schreiner, P. R., "Spectroscopic Evidence for Aminomethylene (H−C̈−NH2)—The
Simplest Amino Carbene." Angew. Chem. Int. Ed. 2018, 57, 5248-5252, DOI: 10.1002/anie.201800679.

InChIs

1: InChI=1S/CH3N/c1-2/h1H,2H2
InChIKey=KASBEVXLSPWGFS-UHFFFAOYSA-N

2: InChI=1S/CH3N/c1-2/h2H,1H2
InChIKey=WDWDWGRYHDPSDS-UHFFFAOYSA-N

Schreiner and Grimme have examined a few compounds (see these previous posts) with long C-C bonds that are found in congested systems where dispersion greatly aids in stabilizing the stretched bond. Their new paper¹ continues this theme by examining 1 (again) and 2, using computations, and x-ray crystallography and gas-phase rotational spectroscopy and electron diffraction to establish the long C-C bond.

The distance of the long central bond in 1 is 1.647 Å (x-ray) and 1.630 Å (electron diffraction). Similarly, this distance in 2 is 1.642 Å (x-ray) and 1.632 Å (ED). These experiments discount any role for crystal packing forces in leading to the long bond.

A very nice result from the computations is that most functionals that include some dispersion correction predict the C-C distance in the optimized structures with an error of no more than 0.01 Å. (PW6B95-D3/DEF2-QZVP structures are shown in Figure 1.) Not surprisingly, HF and B3LYP without a dispersion correction predict a bond that is too long.) MP2 predicts a distance that is too short, but SCS-MP2 does a very good job.

Figure 1. PW6B95-D3/DEF2-QZVP optimized structures of 1 and 2.

References

1) Fokin, A. A.; Zhuk, T. S.; Blomeyer, S.; Pérez, C.; Chernish, L. V.; Pashenko, A. E.; Antony, J.; Vishnevskiy, Y. V.; Berger, R. J. F.; Grimme, S.; Logemann, C.; Schnell, M.; Mitzel, N. W.; Schreiner, P. R., "Intramolecular London Dispersion Interaction Effects on Gas-Phase and Solid-State Structures of Diamondoid Dimers." J. Am. Chem. Soc. 2017, 139, 16696-16707, DOI: 10.1021/jacs.7b07884.

InChIs

1: InChI=1S/C28H38/c1-13-7-23-19-3-15-4-20(17(1)19)24(8-13)27(23,11-15)28-12-16-5-21-18-2-14(9-25(21)28)10-26(28)22(18)6-16/h13-26H,1-12H2
InChIKey=MMYAZLNWLGPULP-UHFFFAOYSA-N

2: InChI=1S/C26H34O2/c1-11-3-19-15-7-13-9-25(19,21(5-11)23(27-13)17(1)15)26-10-14-8-16-18-2-12(4-20(16)26)6-22(26)24(18)28-14/h11-24H,1-10H2
InChIKey=VPBJYHMTINJMAE-UHFFFAOYSA-N

I seem to be recently flooded with papers dealing with tunneling in organic systems. Well, here’s one more! Kozuch, Borden, Schreiner and co-workers seek out systems whereby isotopic substitution might lead to reaction selectivity.¹ Their base system is cyclopropylmethylcarbene 1, which can undergo three different reactions: (a) the ring can expand to give 1-methylcyclobut-1-ene 2, (b) a hydrogen can shift from the terminal methyl group to give vinylcyclopropane 3, or (c) the methane hydrogen can shift to produce ethylidenecyclopropane 4. This last option can be neglected since its barrier (20.5 kcal mol^-1) is so much higher than for the other two, 7.5 kcal mol^-1 for the ring expansion and 12.1 kcal mol^-1 for the [1,2]H-shift converting 1 → 3.

At high temperature, the ring expansion to 2 will dominate, but at low temperature the hydrogen shift to 3 might dominate by tunneling through the barrier due to the low mass and short distances involved. The reaction rates were computed using B3LYP/6-31G(d,p) and small-curvature tunneling. At low temperature, the rate for the hydrogen shift is 10 orders of magnitude faster than the ring expansion. Thinking that deuterium substitution of the terminal methyl group might slow down the rate of the [1,2]-shift, they computed the rates for the reactions of 1-d3, and in fact the rate of this shift does reduce by 10⁴ but it is still much faster than the rate for ring expansion. What is needed is a system where the rate for ring expansion is slower than the rate for hydrogen migration but faster than the rate of deuterium migration.

They examine a number of different substituents that may help to lower the barrier for the ring expansion. The methoxy derivative 5 turns out to suit the bill perfectly. The methoxy group reduces the barrier for ring expansion from 7.5 kcal mol^-1 with 1 to 2.5 kcal mol^-1 with 5. With hydrogenated 5, the [1,2]H-shift is 10³ times faster than ring expansion, but with deuterated 5, ring expansion is twice as fast as the deuterium migration.

The authors call this isotope controlled selectivity (ICS), and this is the first example of this type of control.

References

1. Nandi, A.; Gerbig, D.; Schreiner, P. R.; Borden, W. T.; Kozuch, S., Isotope-Controlled Selectivity by Quantum Tunneling: Hydrogen Migration versus Ring Expansion in Cyclopropylmethylcarbenes. J. Am. Chem. Soc. 2017, 139, 9097-9099, DOI: 10.1021/jacs.7b04593.

InChIs

1: InChI=1S/C5H8/c1-2-5-3-4-5/h5H,3-4H2,1H3
InChIKey=KJIJNBZLGHBOTI-UHFFFAOYSA-N

2: InChIInChIKey=AVPHQXWAMGTQPF-UHFFFAOYSA-N

3: InChI=1S/C5H8/c1-2-5-3-4-5/h2,5H,1,3-4H2
InChIKey=YIWFBNMYFYINAD-UHFFFAOYSA-N

4: InChI=1S/C5H8/c1-2-5-3-4-5/h2H,3-4H2,1H3
InChIKey=ZIFNDRXSSPCNID-UHFFFAOYSA-N

5: InChI=1S/C6H10O/c1-3-6(7-2)4-5-6/h4-5H2,1-2H3
InChIKey=YMBSTCICUAORNN-UHFFFAOYSA-N

6: InChIInChIKey=QBLNAZHAVPMLHB-UHFFFAOYSA-N

7: InChIInChIKey=FHYLDABSPVPDTJ-UHFFFAOYSA-N

Over the past nine years the Schreiner group, often in collaboration with the Allen group, have produced some remarkable studies demonstrating the role of tunneling control. (I have made quite a number of posts on this topics.) Tunneling control is a third mechanism for dictating product formation, in tandem with kinetic control (the favored product is the one that results from the lowest barrier) and thermodynamic control (the favored product is the one that has the lowest energy). Tunneling control has the favored product resulting from the narrowest mass-considered barrier.

Schreiner has written a very clear perspective on tunneling control. It is framed quite interestingly by some fascinating quotes:

It is probably fair to say that many organic chemists view the concept of tunneling, even of hydrogen atoms, with some skepticism. – Carpenter 1983²

Reaction processes have been considered as taking place according to the laws of classical mechanics, quantum mechanical theory being only employed in calculating interatomic forces. – Bell 1933³

Schreiner’s article makes it very clear how critical it is to really think about reactions from a truly quantum mechanical perspective. He notes the predominance of potential energy diagrams that focus exclusively on the relative energies and omits any serious consideration of the reaction coordinate metrics, like barrier width. When one also considers the rise in our understanding of the role of reaction dynamics in organic chemistry (see, for example, these many posts), just how long will it take for these critical notions to penetrate into standard organic chemical thinking? As Schreiner puts it:

It should begin by including quantum phenomena in introductory textbooks, where they are, at least in organic chemistry, blatantly absent. To put this oversight in words similar to those used much earlier by Frank Weinhold in a different context: “When will chemistry textbooks begin to serve as aids, rather than barriers, to this enriched quantum-mechanical perspective?”⁴

References

1) Schreiner, P. R., "Tunneling Control of Chemical Reactions: The Third Reactivity Paradigm." J. Am. Chem. Soc. 2017, 139, 15276-15283, DOI: 10.1021/jacs.7b06035.

2) Carpenter, B. K., "Heavy-atom tunneling as the dominant pathway in a solution-phase reaction? Bond shift in antiaromatic annulenes." J. Am. Chem. Soc. 1983, 105, 1700-1701, DOI: 10.1021/ja00344a073.

3) Bell, R. P., "The Application of Quantum Mechanics to Chemical Kinetics." Proc. R. Soc. London, Ser. A 1933, 139 (838), 466-474, DOI: 10.1098/rspa.1933.0031.

4) Weinhold, F., "Chemistry: A new twist on molecular shape." Nature 2001, 411, 539-541, DOI: 10.1038/35079225.

Following on previous work (see these posts on ladderane and hexaphenylethane), Schreiner, Grimme and co-workers have examined the structure of the all-meta tri(di-t-butylphenyl)methane dimer 1₂.¹ In the study of hexaphenylethane,² Schreiner and Grimme note that t-butyl groups stabilize highly congested structures through dispersion, identifying them as “dispersion energy donors”.³ The idea here is that the dimer of 1 will be stabilized by these many t-butyl groups. In fact, the neutron diffraction study of the crystal structure of 1₂ shows an extremely close approach of the two methane hydrogens of only 1.566 Å, the record holder for the closest approach of two formally non-bonding hydrogen atoms.

To understand the nature of this dimeric structure, they employed a variety of computational techniques. (Shown in Figure 1 is the B3LYPD3^ATM(BJ)/def2-TZVPP optimized geometry of 1₂.) The HSE-3c (a DFT composite method) optimized crystal structure predicts the H^…H distance is 1.555 Å. The computed gas phase structure lengthens the distance to 1.634 Å, indicating a small, but essential, role for packing forces. Energy decomposition analysis of 1₂ at B3LYP-D3^ATM(BJ)/def2-TZVPP indicates a dominant role for dispersion in holding the dimer together. While 1₂ is bound by about 8 kcal mol^-1, the analogue of 1₂ lacking all of the t-butyl groups (the dimer of triphenylmethane 2₂) is unbound by over 8 kcal mol^-1. Topological electron density analysis does show a bond critical point between the two formally unbound hydrogen atoms, and the noncovalent interaction plot shows an attractive region between these two atoms.

Figure 1. ATM(BJ)/def2-TZVPP optimized geometry of 1₂, with most of the hydrogens suppressed for clarity. (Selecting the molecule will launch Jmol with the full structure, including the hydrogens.)

References

1) Rösel, S.; Quanz, H.; Logemann, C.; Becker, J.; Mossou, E.; Cañadillas-Delgado, L.; Caldeweyher, E.; Grimme, S.; Schreiner, P. R., "London Dispersion Enables the Shortest Intermolecular Hydrocarbon H···H Contact." J. Am. Chem. Soc. 2017, 139, 7428–7431, DOI: 10.1021/jacs.7b01879.

2) Grimme, S.; Schreiner, P. R., "Steric Crowding Can Stabilize a Labile Molecule: Solving the Hexaphenylethane Riddle." Angew. Chem. Int. Ed. 2011, 50 (52), 12639-12642, DOI: 10.1002/anie.201103615.

3) Grimme, S.; Huenerbein, R.; Ehrlich, S., "On the Importance of the Dispersion Energy for the Thermodynamic Stability of Molecules." ChemPhysChem 2011, 12 (7), 1258-1261, DOI: 10.1002/cphc.201100127.

InChIs

1: InChI=1S/C43H64/c1-38(2,3)31-19-28(20-32(25-31)39(4,5)6)37(29-21-33(40(7,8)9)26-34(22-29)41(10,11)12)30-23-35(42(13,14)15)27-36(24-30)43(16,17)18/h19-27,37H,1-18H3
InChIKey=VFNQDWKFTWSJAU-UHFFFAOYSA-N

The Schreiner group has again reported an amazing experimental and computational study demonstrating a fascinating quantum mechanical tunneling effect, this time for the trifluoromethylhydroxycarbene (CF₃COH) 2.¹ (I have made on a number of posts discussing a series of important studies in this field by Schreiner.) Carbene 2 is formed, in analogy to many other hydroxycarbenes, by flash vapor pyrolysis of the appropriate oxoacid 1 and capturing the products on a noble gas matrix.

Carbene 2t is observed by IR spectroscopy, and its structure is identified by comparison with the computed CCSD(T)/cc-pVTZ frequencies. When 2t is subjected to 465 nm light, the signals for 2t disappear within 30s, and two new species are observed. The first species is the cis conformer 2c, confirmed by comparison with its computed CCSD(T)/cc-pVTZ frequencies. This cis conformer remains even with continued photolysis. The other product is determined to be trifluoroacetaldehyde 3. Perhaps most interesting is that 2t will convert to 3 in the absence of light at temperatures between 3 and 30 K, with a half-life of about 144 h. There is little rate difference at these temperatures. These results are quite indicative of quantum mechanical tunneling.

To aid in confirming tunneling, they computed the potential energy surface at CCSD(T)/cc-pVTZ. The trans isomer is 0.8 kcal mol^-1 lower in energy that the cis isomer, and this is much smaller than for other hydroxycarbenes they have examined. The rotational barrier TS1 between the two isomer is quite large, 26.4 kcal mol^-1, precluding their interchange by classical means at matrix temperatures. The barrier for conversion of 2t to 3 (TS2) is also quite large, 30.7 kcal mol^-1, and insurmountable at 10K by classical means. No transition state connecting 2c to 3 could be located. These geometries and energies are shown in Figure 1.

2c 0.8

TS1 26.4

2t 0.0

TS2 30.7

3 -49.7

Figure 1. Optimized geometries at CCSD(T)/cc-pVTZ. Relative energies (kcal mol^-1) of each species are listed as well.

WKB computations at M06-2X/6-311++G(d,p) predict a half-life of 172 h, in nice agreement with experiment. The computed half-life for deuterated 2t is 10⁶ years, and the experiment on the deuterated analogue revealed no formation of deuterated 3.

The novel component of this study is that tunneling is conformationally selective. The CF₃ group stabilizes the cis form probably through some weak H^…F interaction, so that the cis isomer can be observed, but no tunneling is observed from this isomer. Only the trans isomer has the migrating hydrogen atom properly arranged for a short hop over to the carbon, allowing the tunneling process to take place.

References

1) Mardyukov, A.; Quanz, H.; Schreiner, P. R., "Conformer-specific hydrogen atom tunnelling in trifluoromethylhydroxycarbene." Nat. Chem. 2017, 9, 71–76, DOI: 10.1038/nchem.2609.

InChIs

1: =1S/C3HF3O3/c4-3(5,6)1(7)2(8)9/h(H,8,9)
InChIKey=GVDJEHMDNREMFA-UHFFFAOYSA-N

2: InChI=1S/C2HF3O/c3-2(4,5)1-6/h6H
InChIKey=FVJVNIREIXAWKU-UHFFFAOYSA-N

3: InChI=1S/C2HF3O/c3-2(4,5)1-6/h1H
InChIKey=JVTSHOJDBRTPHD-UHFFFAOYSA-N

Cyclopropyl rings can be joined together in a spiro fashion to form triangulanes. An interesting topology can be made by joining the rings to form a helical pattern, as shown in the [9]triangulane 1 below. Allen, Quanz, and Schreiner¹ have examined the notion of an infinite helical molecule formed in this way.

First, they describe how one can generate the coordinates of such a beast using a closed analytical expression, which is a really nice demonstration of applied geometry. Next, they compute the geometry of a series of [n]triangulanes at M06-2x/6-31G(d). The geometries of [9]triangulane and their largest example, [42]triangulane 2 are shown in Figure 1.

1

2

Figure 1. M06-2x/6-31G(d) optimized geometries of 1 and 2.

They show that the geometry of 2 exhibits a structure that has two different C-C distances: one between the spiro carbons, and the second between the spiro carbon and the methylene carbon. The distance between the spiro carbons is rather short (1.458 Å), suggesting that the bonding here is between carbons that are nearly sp²-hybridized.

Lastly, they discuss the thermodynamics of polytriangulane. They employ a series of homodesmotic reactions to attempt to determine the enthalpy for adding another cyclopropyl ring to an extended triangulane. Unfortunately, the computed enthalpy is quite dependent on functional used. Similar attempts to define the strain energy is also flawed in this way. However, regardless of the functional the enthalpy for adding a cyclopropane ring appears to reach an asymptote rather quickly. So, using [3]triangulane they estimate that the strain energy per mole of cyclopropane in triangulane is about 42.7 kcal mol^-1, or about 14 kcal mol^-1 of strain due to the spiroannulation.

References

(1) Allen, W. D.; Quanz, H.; Schreiner, P. R. “Polytriangulane,” J. Chem. Theory Comput. 2016, 12, 4707–4716, DOI: 10.1021/acs.jctc.6b00669.

InChIs

1: InChI=1S/C19H22/c1-2-12(1)5-14(12)7-16(14)9-18(16)11-19(18)10-17(19)8-15(17)6-13(15)3-4-13/h1-11H2/t14-,15-,16-,17-,18-,19-/m0/s1
InChIKey=XBTZCZDSKVTALB-DYKIIFRCSA-N

Karton, Schreiner, and Martin have benchmarked the heats of formation of some Platonic Solids and related hydrocarbons.¹ The molecules examined are tetrahedrane 1, cubane 2, dodecahedrane 3, trisprismane 4, pentaprismane 5, and octahedrane 6.

The optimized structures (B3LYP-D3BJ/def2-TZVPP) of these compounds are shown in Figure 1.

1	2
3	4
5	6

Figure 1. B3LYP-D3BJ/def2-TZVPP optimized geometries of 1-6.

Using the W1-F12 and W2-F12 composite methods, the estimated the heats of formation of these hydrocarbons are listed in Table 1. Experimental values are available only for 2 and 3; the computed values are off by about 2 kcal mol^-1, which the authors argue is just outside the error bars of the computations. They suggest that the experiments might need to be revisited.

Table 1. Heats of formation (kcal mol^-1) of 1-6.

They conclude with a comparison of strain energies computed using isogyric, isodesmic, and homodesmotic reactions with a variety of computational methods. Somewhat disappointingly, most DFT methods have appreciable errors compared with the W1-F12 results, and the errors vary depend on the chemical reaction employed. However, the double hybrid method DSD-PBEP86-D3BJ consistently reproduces the W1-F12 results.

References

(1)  Karton, A.; Schreiner, P. R.; Martin, J. M. L. "Heats of formation of platonic hydrocarbon cages by means of high-level thermochemical procedures," J. Comput. Chem. 2016, 37, 49-58, DOI: 10.1002/jcc.23963.

InChIs

1: InChI=1S/C4H4/c1-2-3(1)4(1)2/h1-4H
InChIKey=FJGIHZCEZAZPSP-UHFFFAOYSA-N

2: InChI=1S/C8H8/c1-2-5-3(1)7-4(1)6(2)8(5)7/h1-8H
InChIKey=TXWRERCHRDBNLG-UHFFFAOYSA-N

3: InChI=1S/C20H20/c1-2-5-7-3(1)9-10-4(1)8-6(2)12-11(5)17-13(7)15(9)19-16(10)14(8)18(12)20(17)19/h1-20H
InChIKey=OOHPORRAEMMMCX-UHFFFAOYSA-N

4: InChI=1S/C6H6/c1-2-3(1)6-4(1)5(2)6/h1-6H
InChIKey=RCJOMOPNGOSMJU-UHFFFAOYSA-N

5: InChI=1S/C10H10/c1-2-5-3(1)7-8-4(1)6(2)10(8)9(5)7/h1-10H
InChIKey=TXSUZIHPJIZTJT-UHFFFAOYSA-N

6: InChI=1S/C12H12/c1-2-4-6-5(11-7(1)10(4)11)3(1)9-8(2)12(6)9/h1-12H
InChIKey=DUDCBTWUEJEMGD-UHFFFAOYSA-N

The role of dispersion in organic chemistry has been slowly recognized as being quite critical in a variety of systems. I have blogged on this subject many times, discussing new methods for properly treating dispersion within quantum computations along with a variety of molecular systems where dispersion plays a critical role. Schreiner¹ has recently published a very nice review of molecular systems where dispersion is a key component towards understanding structure and/or properties.

In a similar vein, Wegner and coworkers have examined the Z to E transition of azobenzene systems (1a-g → 2a-g) using both experiment and computation.² They excited the azobenzenes to the Z conformation and then monitored the rate for conversion to the E conformation. In addition they optimized the geometries of the two conformers and the transition state for their interconversion at both B3LYP/6-311G(d,p) and B3LYP-D3/6-311G(d,p). The optimized structure of the t-butyl-substituted system is shown in Figure 1.

a: R=H; b: R=tBu; c: R=Me; d: R=iPr; e: R=Cyclohexyl; f: R=Adamantyl; g: R=Ph

1b

1b-TS-2b

2b

Figure 1. B3LYP-D3/6-311G(d,p) optimized geometries of 1a, 2a, and the TS connecting them.

The experiment finds that the largest activation barriers are for the adamantly 1f and t-butyl 1b azobenzenes, while the lowest barriers are for the parent 1a and methylated 1c azobenzenes.

The trends in these barriers are not reproduced at B3LYP but are reproduced at B3LYP-D3. This suggests that dispersion is playing a role. In the Z conformations, the two phenyl groups are close together, and if appropriately substituted with bulky substituents, contrary to what might be traditionally thought, the steric bulk does not destabilize the Z form but actually serves to increase the dispersion stabilization between these groups. This leads to a higher barrier for conversion from the Z conformer to the E conformer with increasing steric bulk.

References

(1) Wagner, J. P.; Schreiner, P. R. "London Dispersion in Molecular Chemistry—Reconsidering Steric Effects," Angew. Chem. Int. Ed. 2015, 54, 12274-12296, DOI: 10.1002/anie.201503476.

(2) Schweighauser, L.; Strauss, M. A.; Bellotto, S.; Wegner, H. A. "Attraction or Repulsion? London Dispersion Forces Control Azobenzene Switches," Angew. Chem. Int. Ed. 2015, 54, 13436-13439, DOI: 10.1002/anie.201506126.

InChIs

1b: InChI=1S/C28H42N2/c1-25(2,3)19-13-20(26(4,5)6)16-23(15-19)29-30-24-17-21(27(7,8)9)14-22(18-24)28(10,11)12/h13-18H,1-12H3/b30-29-
InChIKey=SOCNVTNVHBWFKC-FLWNBWAVSA-N

2b: InChI=1S/C28H42N2/c1-25(2,3)19-13-20(26(4,5)6)16-23(15-19)29-30-24-17-21(27(7,8)9)14-22(18-24)28(10,11)12/h13-18H,1-12H3/b30-29+
InChIKey=SOCNVTNVHBWFKC-QVIHXGFCSA-N

A 2013 study of oxalic acid 1 failed to uncover any tunneling between its conformations,¹ despite observation of tunneling in other carboxylic acids (see this post). This was rationalized by computations which suggested rather high rearrangement barriers. Schreiner, Csaszar, and Allen have now re-examined oxalic acid using both experiments and computations and find what they call domino tunneling.²

First, they determined the structures of the three conformations of 1 along with the two transition states interconnecting them using the focal point method. These geometries and relative energies are shown in Figure 1. The barrier for the two rearrangement steps are smaller than previous computations suggest, which suggests that tunneling may be possible.

1tTt (0.0)	TS1 (9.7)
1cTt (-1.4)	TS2 (9.0)
1cTc (-4.0)

Figure 1. Geometries of the conformers of 1 and the TS for rearrangement and relative energies (kcal mol^-1)

Placing oxalic acid in a neon matrix at 3 K and then exposing it to IR radiation populates the excited 1tTt conformation. This state then decays to both 1cTt and 1cTc, which can only happen through a tunneling process at this very cold temperature. Kinetic analysis indicates that there are two different rates for decay from both 1tTt and 1cTc, with the two rates associated with different types of sites within the matrix.

The intrinsic reaction paths for the two rearrangements: 1tTt → 1cTt and → 1cTc were obtained at MP2/aug-cc-pVTZ. Numerical integration and the WKB method yield similar half-lives: about 42 h for the first reaction and 23 h for the second reaction. These match up very well with the experimental half-lives from the fast matrix sites of 43 ± 4 h and 30 ± 20 h, respectively. Thus, the two steps take place sequentially via tunneling, like dominos falling over.

References

(1) Olbert-Majkut, A.; Ahokas, J.; Pettersson, M.; Lundell, J. "Visible Light-Driven Chemistry of Oxalic Acid in Solid Argon, Probed by Raman Spectroscopy," J. Phys. Chem. A 2013, 117, 1492-1502, DOI: 10.1021/jp311749z.

(2) Schreiner, P. R.; Wagner, J. P.; Reisenauer, H. P.; Gerbig, D.; Ley, D.; Sarka, J.; Császár, A. G.; Vaughn, A.; Allen, W. D. "Domino Tunneling," J. Am. Chem. Soc. 2015, 137, 7828-7834, DOI: 10.1021/jacs.5b03322.

InChIs

1: InChI=1S/C2H2O4/c3-1(4)2(5)6/h(H,3,4)(H,5,6)
InChIKey=MUBZPKHOEPUJKR-UHFFFAOYSA-N