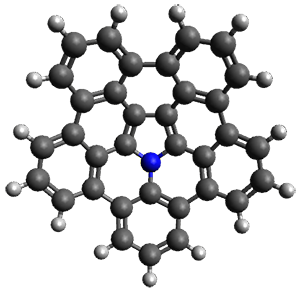
A heterosubstituted corranulene analogue has now been prepared. Ito, Tokimaru, and Nozaki report the synthesis of 1 and compare it with corranulene.¹ The x-ray structure of 1 shows it to be a deeper bowl than corranulene, and the bond distances suggest the Kekule structure with a central pyrrole and five Clar-type phenyl rings.

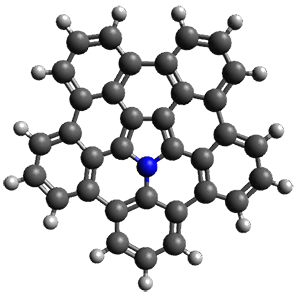
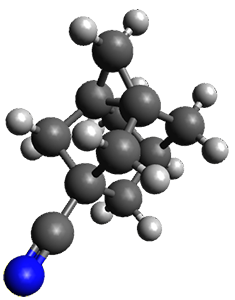
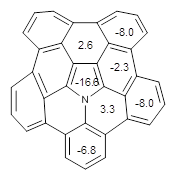
The B3LYP/6-311+G(2d,p) optimized structure of 2, then analogue of 1 missing the t-butyl group, is shown in Figure 1. Its geometry is very similar to that of 1 observed in the crystal structure. The NICS(0) values are shown in Scheme 1. These values support the notion of a central (aromatic) pyrrole surrounded by a periphery of five aromatic phenyl rings.

Scheme 1. NICS(0) values

An interesting feature of bowl compounds is their inversion. The inversion barrier, through the planar TS shown in Figure 2, is computed to be 17.0 kcal mol^-1 at B3LYP/6-311+G(2d,p). This is 6-7 kcal mol^-1 larger than the inversion barrier of corranulene, which is not surprising given the additional phenyl groups about the periphery.

2	bowl inversion TS

Figure 1. B3LYP/6-311+G(2d,p) optimized geometry of 2.

References

(1) Ito, S.; Tokimaru, Y.; Nozaki, K. "Benzene-Fused Azacorannulene Bearing an Internal Nitrogen Atom," Angew. Chem. Int. Ed. 2015, 54, 7256-7260, DOI: 10.1002/anie.201502599.

InChI

1: InChI=1S/C38H23N/c1-38(2,3)18-16-27-25-14-6-12-23-21-10-4-8-19-20-9-5-11-22-24-13-7-15-26-28(17-18)35(27)39-36(31(23)25)33(29(19)21)34(30(20)22)37(39)32(24)26/h4-17H,1-3H3
InChIKey= JJPREOOFFSVARV-UHFFFAOYSA-N

2: InChI=1S/C34H15N/c1-6-16-17-7-2-9-19-21-11-4-13-23-25-15-5-14-24-22-12-3-10-20-18(8-1)26(16)30-31(27(17)19)34(29(21)23)35(32(24)25)33(30)28(20)22/h1-15H
InChIKey= FFFUKUDWQMOFQQ-UHFFFAOYSA-N

Last year I wrote a post on the possibility of a stable hypercoordinated carbon in the C(CH₃)₅⁺ molecule as proposed by Schleyer and Schaefer.¹ Kozuch has re-examined this molecule with an eye towards examining the lifetime of this proposed “fleeting” molecule.²

The computed barriers for either (1) loss of a methane molecule leaving behind the (CH₃)₂C⁺CH₂CH₃ cation or (2) loss of an ethane molecule leaving behind the t-butyl cation are small: 1.65 and 1.37 kcal mol^-1, respectively. Kozuch employed canonical variational theory with and without small curvature tunneling (SCT). Without the tunneling correction, the pentamethylmethyl cation is predicted to have a long (millennia) lifetime at very low temperatures (<20 K). However, when tunneling is included, the half-life is reduced to 6 and 40 μs for degradation along the two pathways. Clearly, this is not a fleeting molecule – its lifetime is really too short to consider it as anything.

Interestingly, perdeuterating the molecule ((CD₃)₅C⁺) substantially increases the half-life to 4 ms, a thousand-fold increase. Tritium substitution would further increase the half-life to 0.2 s – a long enough time to really identify it and perhaps justify the name “molecule”. What is perhaps the most interesting aspect here is that H/D substitution has such a large effect on the tunneling rate even though no C-H bond is broken in the TS! This results from a mass effect (a CH₃ vs. a CD₃ group is migrating) along with a zero-point vibrational energy effect.

References

(1) McKee, W. C.; Agarwal, J.; Schaefer, H. F.; Schleyer, P. v. R. "Covalent Hypercoordination: Can Carbon Bind Five Methyl Ligands?," Angew. Chem. Int. Ed. 2014, 53, 7875-7878, DOI: 10.1002/anie.201403314.

(2) Kozuch, S. "On the tunneling instability of a hypercoordinated carbocation," Phys. Chem. Chem. Phys. 2015, 17, 16688-16691, DOI: 10.1039/C5CP02080H.

InChIs

C(CH₃)₅⁺: InChI=1S/C6H15/c1-6(2,3,4)5/h1-5H3/q+1
InChIKey=GGCBGJZCTGZYFV-UHFFFAOYSA-N

Inverted carbon atoms, where the bonds from a single carbon atom are made to four other atoms which all on one side of a plane, remain a subject of fascination for organic chemists. We simply like to put carbon into unusual environments! Bremer, Fokin, and Schreiner have examined a selection of molecules possessing inverted carbon atoms and highlights some problems both with experiments and computations.¹

The prototype of the inverted carbon is propellane 1. The ­C_inv-C_inv bond distance is 1.594 Å as determined in a gas-phase electron diffraction experiment.² A selection of bond distance computed with various methods is shown in Figure 1. Note that CASPT2/6-31G(d), CCSD(t)/cc-pVTZ and MP2 does a very fine job in predicting the structure. However, a selection of DFT methods predict a distance that is too short, and these methods include functionals that include dispersion corrections or have been designed to account for medium-range electron correlation.

CASPT2/6-31G(d) CCSD(T)/cc-pVTZ MP2/cc-pVTZ MP2/cc-pVQZ B3LYP/6-311+G(d,p) B3LYP-D3BJ/6-311+G(d,p) M06-2x/6-311+G(d,p)	1.596 1.595 1.596 1.590 1.575 1.575 1.550

Figure 1. Optimized Structure of 1 at MP2/cc-pVTZ, along with C_inv-C_inv distances (Å) computed with different methods.

Propellanes without an inverted carbon, like 2, are properly described by these DFT methods; the C-C distance predicted by the DFT methods is close to that predicted by the post-HF methods.

The propellane 3 has been referred to many times for its seemingly very long C_inv-C_inv bond: an x-ray study from 1973 indicates it is 1.643 Å.³ However, this distance is computed at MP2/cc-pVTZ to be considerably shorter: 1.571 Å (Figure 2). Bremer, Fokin, and Schreiner resynthesized 3 and conducted a new x-ray study, and find that the C_inv-C_inv distance is 1.5838 Å, in reasonable agreement with the computation. This is yet another example of where computation has pointed towards experimental errors in chemical structure.

Figure 2. MP2/cc-pVTZ optimized structure of 3.

However, DFT methods fail to properly predict the C_inv-C_inv distance in 3. The functionals B3LYP, B3LYP-D3BJ and M06-2x (with the cc-pVTZ basis set) predict a distance of 1.560, 1.555, and 1.545 Å, respectively. Bremer, Folkin and Schreiner did not consider the ωB97X-D functional, so I optimized the structure of 3 at ωB97X-D/cc-pVTZ and the distance is 1.546 Å.

Inverted carbon atoms appear to be a significant challenge for DFT methods.

References

(1) Bremer, M.; Untenecker, H.; Gunchenko, P. A.; Fokin, A. A.; Schreiner, P. R. "Inverted Carbon Geometries: Challenges to Experiment and Theory," J. Org. Chem. 2015, 80, 6520–6524, DOI: 10.1021/acs.joc.5b00845.

(2) Hedberg, L.; Hedberg, K. "The molecular structure of gaseous [1.1.1]propellane: an electron-diffraction investigation," J. Am. Chem. Soc. 1985, 107, 7257-7260, DOI: 10.1021/ja00311a004.

(3) Gibbons, C. S.; Trotter, J. "Crystal Structure of 1-Cyanotetracyclo[3.3.1.1^3,7.0^3,7]decane," Can. J. Chem. 1973, 51, 87-91, DOI: 10.1139/v73-012.

InChIs

1: InChI=1S/C5H6/c1-4-2-5(1,4)3-4/h1-3H2
InChIKey=ZTXSPLGEGCABFL-UHFFFAOYSA-N

3: InChI=1S/C11H13N/c12-7-9-1-8-2-10(4-9)6-11(10,3-8)5-9/h8H,1-6H2
InChIKey=KTXBGPGYWQAZAS-UHFFFAOYSA-N