Optical activity is a major tool for identifying enantiomers. With recent developments in computational techniques, it is hoped that experiments combined with computations will be a powerful tool for determining absolute configuration. The recent work of Lahiri, et al. demonstrates the scope of theoretical work that is still needed to really make this approach broadly applicable.¹

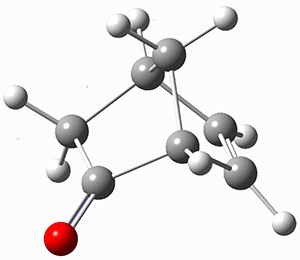
They prepared (1R,4R)-norbornenone 1 and measured its optical rotation in the gas phase and in dilute solutions of acetonitrile and cyclohexane. The specific rotations at three different wavelengths are listed in Table 1. Of first note is that though there is some small differences in solution, as expected, there really is substantial differences between the gas- and solution phases. Thus cautionary point 1: be very careful of comparing solution phase experimental optical activity with computed gas phase predictions.

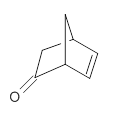
1

Table 1. Experimental and computed specific rotation of 1.

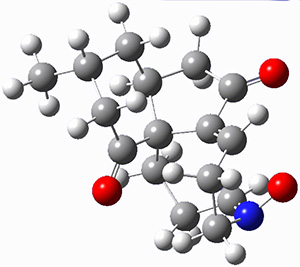
For the computations, the geometry of 1 was optimized at B3LYP/aug-cc-pVTZ (see Figure 1. The OR was computed at B3LYP with different basis sets, finding that the difference in the predicted specific rotation at 598.3nm differs only quite little (90.6 deg dm^-1 (g/mL)^-1) between the computations using aug-cc-pVTZ and aug-cc-pVQZ) suggesting that the basis set limit has been reached. The gas –phase computed values at B3LYP and CCSD are also shown in Table 1. Though these computations do get the correct sign of the rotation, they are appreciably off of the experimental values, and the percent error varies with wavelength. Cautionary point 2: it is not obvious what is the appropriate computational method to compute OR, and beware of values that seem reasonable at one wavelength – this does not predict a good agreement at a different wavelength.

Figure 1. Optimized geometry of 1 at B3LYP/aug-cc-pVTZ.

Lastly, computed solution values of the OR were performed with PCM and B3LYP. These values are given in Table 1. Again the agreement is poor. So cautionary point 3: computed (PCM) solution OR
may be in quite poor agreement with experiment.

Often the culprit to poor agreement between computed and experimental OR is attributed to omitted vibrational effects, but in this case, because 1 is so rigid, one might not expect too much error to be caused by the effects of vibrations. So the overall result – we need considerable work towards addressing how to accurately compute optical activity!

References

(1) Lahiri, P.; Wiberg, K. B.; Vaccaro, P. H.; Caricato, M.; Crawford, T. D. "Large Solvation Effect in the Optical Rotatory Dispersion of Norbornenone," Angew. Chem. Int. Ed. 2014, 53, 1386-1389, DOI: 10.1002/anie.201306339.

InChIs

1: InChI=1S/C7H8O/c8-7-4-5-1-2-6(7)3-5/h1-2,5-6H,3-4H2/t5-,6+/m1/s1
InChIKey=HUQXEIFQYCVOPD-RITPCOANSA-N

The conformational space of monosaccharides is amazingly complex. If we consider just the pyranose form, the ring can in principal exist as a chair, a half-chair, skew (or twist boat) and boat form, for a total of 38 puckering configurations. Layer on top of this the axial and equatorial positions of the hydroxyl and methylhydroxyl groups, and then the rotamers of these substituents, and one is faced with a dauntingly vast space. It is just this space that Beckham and co-workers¹ take on for α- and β-glucose, β-xylose, β-mannose and β-acetylglucosamine.

For each sugar, and for each of the 38 puckering configurations, full rotamer scans for each of the substituents led to 27,702 conformations of each of the four monosaccharides, and 36,936 conformations of β-acetylglucosamine. This totals to over 123,000 geometry optimizations that were carried out at M06-2x/6-31G(d). Then taking the structures within 5 kcal mol^-1 of the lowest energy structure for ­each pucker, they reoptimized at M06-2X/6-31+G(d,p). Pruning once again those structures that were above 5 kcal mol^-1 of the minimum, they performed CCSD(T)/6-311+G(d,p)//B3LYP/6-311+G(2df,p) computations. What a tour de force!

The results of these conformational space surveys are not terribly exciting. The substituents do make a difference in dictating the most and least favorable structures and the activation barriers for interconversion of ring forms.

These PESs will be quite useful in understanding carbohydrate conformations and the role these may play in their chemistry. But the point of bringing this paper to your attention is the tremendously complex, detailed PES that is uncovered, representing the scale of what can be done with modern computers and modern algorithms.

References

(1) Mayes, H. B.; Broadbelt, L. J.; Beckham, G. T. "How Sugars Pucker: Electronic Structure Calculations Map the Kinetic Landscape of Five Biologically Paramount Monosaccharides and Their Implications for Enzymatic Catalysis," Journal of the American Chemical Society 2013, 136, 1008-1022, DOI: 10.1021/ja410264d.

InChIs

α-glucose: InChI=1S/C6H12O6/c7-1-2-3(8)4(9)5(10)6(11)12-2/h2-11H,1H2/t2-,3-,4+,5-,6+/m1/s1
InChIKey: WQZGKKKJIJFFOK-DVKNGEFBSA-N

β-glucose: InChI=1S/C6H12O6/c7-1-2-3(8)4(9)5(10)6(11)12-2/h2-11H,1H2/t2-,3-,4+,5-,6-/m1/s1
InChIKey=WQZGKKKJIJFFOK-VFUOTHLCSA-N

β-xylose: InChI=1S/C5H10O5/c6-2-1-10-5(9)4(8)3(2)7/h2-9H,1H2/t2-,3+,4-,5-/m1/s1
InChIKey=SRBFZHDQGSBBOR-KKQCNMDGSA-N

β-mannose: InChI=1S/C6H12O6/c7-1-2-3(8)4(9)5(10)6(11)12-2/h2-11H,1H2/t2-,3-,4+,5+,6-/m1/s1
InChIKey=WQZGKKKJIJFFOK-RWOPYEJCSA-N

β-acetylglucosamine: InChI=1S/C8H15NO6/c1-3(11)9-5-7(13)6(12)4(2-10)15-8(5)14/h4-8,10,12-14H,2H2,1H3,(H,9,11)/t4-,5-,6-,7-,8-/m1/s1
InChIKey=OVRNDRQMDRJTHS-FMDGEEDCSA-N

The importance of dispersion in determining molecular structure, even the structure of a single medium-sized molecule, is now well recognized. This means that quantum methods that do not account for dispersion might give very poor structures.

Grimme¹ takes an interesting new twist towards assessing the geometries produced by computational methods by evaluating the structures based on their rotational constants B₀ obtained from microwave experiments. He uses nine different molecules in his test set, shown in Scheme 1. This yields 25 different rotational constants (only one rotational constant is available from the experiment on triethylamine). He evaluates a number of different computational methods, particularly DFT with and without a dispersion correction (either the D3 or the non-local correction). The fully optimized geometry of each compound with each method is located to then the rotational constants are computed. Since this provides B_e values, he has computed the vibrational correction to each rotational constant for each molecule, in order to get “experimental” B_e values for comparisons.

Scheme 1.

Grimme first examines the basis set effect for vitamin C and aspirin using B3LYP-D3. He concludes that def2-TZVP or lager basis sets are necessary for reliable structures. However, the errors in the rotational constant obtained at B3LYP-D3/6-31G* is at most 1.7%, and even with CBS the error can be as large as 1.1%, so to my eye even this very small basis set may be completely adequate for many purposes.

In terms of the different functionals (using the DZVP basis set), the best results are obtained with the double hybrid B2PLYP-D3 functional where the mean relative deviation is only 0.3%; omitting the dispersion correction only increases the mean error to 0.6%. Common functionals lacking the dispersion correction have mean errors of about 2-3%, but with the correction, the error is appreciably diminished. In fact B3LYP-D3 has a mean error of 0.9% and B3LYP-NL has an error of only 0.6%. In general, the performance follows the Jacob’s Ladder hierarchy.

References

(1) Grimme, S.; Steinmetz, M. "Effects of London dispersion correction in density functional theory on the structures of organic molecules in the gas phase," Phys. Chem. Chem. Phys. 2013, 15, 16031-16042, DOI: 10.1039/C3CP52293H.

The click reaction has become a major workhorse of synthetic chemists since its proposal in 2001.¹ Despite its efficiencies, no clear-cut example of its use in nature has been reported until 2012, where Yu and co-workers speculated that it might be utilized in the biosynthesis of lycojaponicumin A and B.² Krenske, Patel, and Houk have examined the possibility of an enzyme activated click process in forming this natural product.³

First they examined the gas-phase intramolecular [3+2] reaction that takes 1 into 2.

They identified (at M06-2X/def2-TZVPP/M06-2X/6-31+G(d,p)) four different low-energy conformations of 1, of which three have the proper orientation for the cyclization to occur. The lowest energy conformer, the TS, and the product 2 are shown in Figure 1. The free energy activation barrier in the gas phase is 19.8 kcal mol^-1. Inclusion of water as an implicit solvent (through a TS starting from a different initial conformation) increases the barrier to 20.0 kcal mol^-1. Inclusion of four explicit water molecules, hydrogen bonded to the nitrone and enone, predicts a barrier of 20.5 kcal mol^-1. These values predict a slow reaction, but not totally impossible. In fact, Tantillo in a closely related work reported a theoretical study of the possibility of a [3+2] cyclization in the natural synthesis of flueggine A and virosaine, and found barriers of comparable size as here. Tantillo concludes that enzymatic activation is not essential.⁴

1

TS12

3

Table 1. M06-2X/6-31+G(d,p) optimized geometries of 1, TS12, and 2.

To model a potential enzyme, the Houk group created a theozyme whereby two water molecules act as hydrogen bond donors to the enone and the use of implicit solvent (diethyl ether) to mimic the interior of an enzyme. This theozyme model predicts a barrier of 15.3 kcal mol^-1, or a 2000 fold acceleration of the click reaction. The search for such an enzyme might prove quite intriguing.

References

(1) Kolb, H. C.; Finn, M. G.; Sharpless, K. B. "Click Chemistry: Diverse Chemical Function from a Few Good Reactions," Angew. Chem. Int. Ed. 2001, 40, 2004-2021, DOI: 10.1002/1521-3773(20010601)40:11<2004::AID-ANIE2004>3.0.CO;2-5.

(2) Wang, X.-J.; Zhang, G.-J.; Zhuang, P.-Y.; Zhang, Y.; Yu, S.-S.; Bao, X.-Q.; Zhang, D.; Yuan, Y.-H.; Chen, N.-H.; Ma, S.-g.; Qu, J.; Li, Y. "Lycojaponicumins A–C, Three Alkaloids with an Unprecedented Skeleton from Lycopodium japonicum," Org. Lett. 2012, 14, 2614-2617, DOI: 10.1021/ol3009478.

(3) Krenske, E. H.; Patel, A.; Houk, K. N. "Does Nature Click? Theoretical Prediction of an Enzyme-Catalyzed Transannular 1,3-Dipolar Cycloaddition in the Biosynthesis of Lycojaponicumins A and B," J. Am. Chem. Soc. 2013, 135, 17638-17642, DOI: 10.1021/ja409928z.

(4) Painter, P. P.; Pemberton, R. P.; Wong, B. M.; Ho, K. C.; Tantillo, D. J. "The Viability of Nitrone–Alkene (3 + 2) Cycloadditions in Alkaloid Biosynthesis," J. Org. Chem. 2014, 79, 432–435, DOI: 10.1021/jo402487d.

InChIs

1: InChI=1S/C16H21NO3/c1-11-8-12-10-14(18)13-4-2-6-17(20)7-3-5-16(12,13)15(19)9-11/h4,7,11-12H,2-3,5-6,8-10H2,1H3/b13-4-,17-7+
InChIKey=GVEYMXKHRRHCLV-KYGYAMEJSA-N

2: InChI=1S/C16H21NO3/c1-9-6-10-8-13(19)16-11(17-5-3-14(16)20-17)2-4-15(10,16)12(18)7-9/h9-11,14H,2-8H2,1H3/t9?,10-,11?,14?,15+,16-/m0/s1
InChIKey=QKFAOJHYKPBTKX-SGVNFLFUSA-N