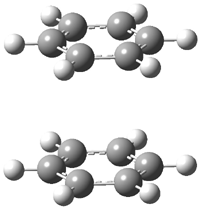
The nature of the bridgehead-bridgehead bond in [1.1.1]propellane 1 poses an interesting quandary. The bond involves two inverted carbon atoms, whose hybrids should point away from each other. The internuclear region has in fact much less electron density than for an ordinary C-C bond. Nonetheless, the molecule is stable and the C-C bond is estimated to have a strength of about 6 kcal mol^-1.

Shaik and Hiberty¹ have now proposed that the central C-C bond of [1.1.1]propellane is a charge-shift bond. In classical valence bond theory, we have three configurations for a bond: the covalent structure A↑↓B ↔ A↓↑B, and the two ionic structures A↑↓ B and A B↑↓. The description of a typical covalent bond is dominated by the covalent VB structure with a little bit of the ionic structures mixed in. A charge-shift bond is one where the resonance energy due to the mixing of the covalent and ionic structures mostly accounts for the stabilization of the bond.² Just such a case is found in the F-F bond, and also to for the central C-C bond of [1.1.1]propellane!

References

(1) Wu, W.; Gu, J.; Song, J.; Shaik, S.; Hiberty, P. C., "The Inverted Bond in [1.1.1]Propellane is a Charge-Shift Bond," Angew. Chem. Int. Ed., 2008, ASAP DOI: 10.1002/anie.200804965

(2) Shaik, S.; Danovich, D.; Silvi, B.; Lauvergnat, D. L.; Hiberty, P. C., "Charge-Shift Bonding – A Class of Electron-Pair Bonds That Emerges from Valence Bond Theory and Is Supported by the Electron Localization Function Approach," Chem. Eur. J., 2005, 11, 6358-6371, DOI: 10.1002/chem.200500265

InChI

1: InChI=1/C5H6/c1-4-2-5(1,4)3-4/h1-3H2
InChIKey=ZTXSPLGEGCABFL-UHFFFAOYAJ

In sort of an afterword to a recent publication, Stanger¹ points out an error made by Hückel in arguing for stability of D_6h benzene over the hypothetical D_3h cyclohexatriene. Hückel constructed the first two matrices shown below to describe each molecule. The energy of benzene, predicted by this matrix, is 6α-8β, which is lower than that for cyclohexatriene, 6α-6β.

Stanger points out that implicit in Hückel’s argument is that the value of the H_ij element for the double bond is identical in value for the two compounds, (namely β), and the element is zero for the single bonds. Considering that the C-C double bond in cyclohexatriene should be shorter than that in benzene, Stanger suggests that its H_ij element should be larger than β and the H_ij element for the single bond should not be zero, but some small value reflecting the overlap between the p-orbitals. He suggests that the values for the single and double bond elements should be 0.4589β and 1.5050β, giving the bottom matrix below. This leads to an electronic energy of 6α-9.212β. In other words, this more appropriate model of cyclohexatriene has a lower π energy than does D_6h benzene! This is in accord with Shaik’s argument² that the π system of benzene acts to localize the bonds and it’s the σ system that is responsible for its delocalized structure.

Figure 1. Hückel matrices and eigenvalues for benzene (top),
traditional cyclohexatriene model (middle), and revised cyclohexatriene model (bottom).¹

References

(1) Stanger, A., "The Different Aromatic Characters of Some Localized Benzene Derivatives&#x2020," J. Phys. Chem. A, 2008, 112, 12849-12854, DOI: 10.1021/jp801634x

(2) Shaik, S.; Shurki, A.; Danovich, D.; Hiberty, P. C., "A different story of benzene," Journal of Molecular Structure: THEOCHEM, 1997, 398-399, 155-167, DOI: 10.1016/S0166-1280(96)04934-2.

Hobza¹ has published a very high-level computational study of the benzene dimer as a benchmark for this model of π-π stacking – a topic I have touched upon a number of times in this blog (post 1, post 2) . There are four local energy minima, shown in Figure 1. The most stable dimer is the tilted T-structure (TT), a structure often overlooked. Its complexation energy, computed at CCSD(T)/CBS, is 2.78 kcal mol^-1. Only slightly higher in energy is the parallel displaced structure (PD), with a stabilization energy of 2.70 kcal mol^-1. The T structure (T) is essentially isoenergetic with the PD one. The perfectly stacked structure (S) is much less stable, with a dimerization energy of 1.64 kcal mol^-1. The DTF-D method, using the BLYP functional with dispersion parameters optimized for the benzene dimer provide energies within 0.2 kcal mol^-1 of the computationally much more expensive benchmark values. As a word of caution though: use of more general dispersion parameters gives energies far worse and predicts the wrong energy order of the dimers.

TT 2.78 2.93 2.33	PD 2.70 2.88 2.57
T 2.69 2.80 2.03	S 1.64 1.84 1.45

Figure 1. Structures of the benzene dimer with stabilization energy (kcal mol^-1) computed at CCSD(T)/CBS (bold), DFT-D/BLYP with optimized parameters (italics), and DFT-D/BLYP with general parameters (plain).¹

References

(1) Pitonak, M.; Neogrady, P.; Rexac, J.; Jurecka, P.; Urban, M.; Hobza, P., "Benzene Dimer: High-Level Wave Function and Density Functional Theory Calculations," J. Chem. Theory Comput., 2008, 4, 1829-1834, DOI: 10.1021/ct800229h.

Rzepa has extended the concept of Möbius aromaticity to homoaromaticity.¹ 1 is the homoaromatic analogue of the tropylium cation. Topoligical electron density analysis, also known as Atoms-In-Molecules (AIM), indicates no bond path connecting C₁and C₇. However, the NICS value at the ring critical point of 1 is -11.5 ppm, indicative of aromaticity. 2 is the potential Möbius aromatic analogue of 1. Unlike 1 which has a plane of symmetry, 2 has a C₂ rotational axis of symmetry, as anticipated for a Möbius homoaromatic compound. However, there is no bond path connecting C₁ with C₉. But, the NICS value at the ring critical point of 2 is -11.3 ppm, supporting the notion of aromatic character! Suprisingly, the AIM analysis of the larger homologue 3 does have a bond path connecting C₁ to C₉, even though the distance separating these compounds is larger than in 2! Again the NICS value for 3 is negative (-9.8) and so it certainly appears to be Möbius homoaromatic.

The B3LYP/aug-cc-pVYZ structures of 1-3 are shown in Figure 1. As is Rzepa’s practice, he provides an extensive collection of data on the molecules he reports making great use of electronic depositories, and it looks like the ACS has now moved this “web-enhanced table” out into the open part of its web site: http://pubs.acs.org/doi/suppl/10.1021/ct8001915/suppl_file/index.html.

1	2
3

Figure 1. B3LYP/aug-cc-pVYZ optimized structures of 1-3.¹

References

(1) Allan, C. S. M.; Rzepa, H. S., "Chiral Aromaticities. A Topological Exploration of Möbius Homoaromaticity," J. Chem. Theory Comput., 2008, 4, 1841-1848, DOI: 10.1021/ct8001915

InChIs

1: InChI=1/C8H9/c1-2-4-6-8-7-5-3-1/h1-7H,8H2/q+1/b2-1-,5-3-,6-4-
InChIKey=ZINXKSGXPFSBNB-XCADPSHZBA

2: InChI=1/C10H11/c1-2-4-6-8-10-9-7-5-3-1/h1-9H,10H2/q+1/b2-1-,5-3-,6-4-,9-7-
InChIKey=HBJCUFQWAIKURE-BWYSQNKRBE

3: InChI=1/C11H13/c1-2-4-6-8-10-11-9-7-5-3-1/h1-9H,10-11H2/q+1/b2-1-,5-3-,6-4-,9-7-InChIKey=LUGAIBOLHSLVBJ-BWYSQNKRBF

Here’s another nice example of computed NMR spectra being
used to identify complex organic structures.¹

An alkaloid isolated from the custard apple tree was assigned the structure 1 and christened with the name samoquasine A.² Two years later, the authors determined that samoquasine A was actually identical to perlolidine 2.³ Independent synthesis of the compound with structure 1 showed that its properties were not identical to that of samoquasine A.^4,5 The properties of perlolidine were then found to differ from that of samoquasine A,⁴ leaving a void as to just what is the structure of samoquasine A.

Given that compounds 1 and the related compounds 3 and 4 had been prepared and their NMR spectra obtained, Timmons and Wipf¹ decided to compute the ¹³C NMR spectra of 48 related compounds at B3LYP/6-311+G(2d,p)//B3LYP/6-31G(d). The mean absolute difference between the computed and experimental chemical shifts for 1, 3 and 4 are less than 2 ppm. Of the remaining 45 compounds, the one whose chemical shifts match best with that of samoquasine A is 2, with a mean absolute deviation of 1.8 ppm. This agreement supports the contention that samoquasine A and perlolidine are in fact identical. The authors contend that the experimental data used to conjecture that they were not identical is in fact faulty.

References

(1) Timmons, C.; Wipf, P., "Density Functional Theory Calculation of 13C NMR Shifts of Diazaphenanthrene Alkaloids: Reinvestigation of the Structure of Samoquasine A," J. Org. Chem., 2008, 73, 9168-9170, DOI: 10.1021/jo801735e.

(2) Morita, H.; Sato, Y.; Chan, K.-L.; Choo, C.-Y.; Itokawa, H.; Takeya, K.; Kobayashi, J. i., "Samoquasine A, a Benzoquinazoline Alkaloid from the Seeds of Annona squamosa," J. Nat. Prod., 2000, 63, 1707-1708, DOI: 10.1021/np000342i.

(3) Morita, H.; Sato, Y.; Chan, K.-L.; Choo, C.-Y.; Itokawa, H.; Takeya, K.; Kobayashi, J. i., "Samoquasine A, a Benzoquinazoline Alkaloid from the Seeds of Annona squamosa," J. Nat. Prod., 2002, 65, 1748-1748, DOI: 10.1021/np0204343.

(4) Yang, Y.-L.; Chang, F.-R.; Wu, Y.-C., "Total synthesis of 3,4-dihydrobenzo[h]quinazolin-4-one
and structure elucidation of perlolidine and samoquasine A," Tetrahedron Letters, 2003, 44, 319-322, DOI: 10.1016/S0040-4039(02)02577-7.

(5) Chakrabarty, M.; Sarkara, S.; Harigaya, Y., "An Expedient Synthesis of Benzo[h]quinazolin-4(3H)-one: Structure of Samoquasine A Revisited," Synthesis, 2003, 2292-2294, DOI: 10.1055/s-2003-42409.

InChIs

1: InChI=1/C12H8N2O/c15-12-10-6-5-8-3-1-2-4-9(8)11(10)13-7-14-12/h1-7H,(H,13,14,15)/f/h14H
InChIKey=BJVYARVTSUNBMW-YHMJCDSICO

2: InChI=1/C12H8N2O/c15-12-10-7-14-11-4-2-1-3-9(11)8(10)5-6-13-12/h1-7H,(H,13,15)/f/h13H
InChIKey=ULIAUQBOGQCMQM-NDKGDYFDCS

3: InChI=1/C12H8N2O/c15-12-10-6-5-8-3-1-2-4-9(8)11(10)7-13-14-12/h1-7H,(H,14,15)/f/h14H
InChIKey=JFJSVLSRVOIBPG-YHMJCDSICJ

4: InChI=1/C12H8N2O/c15-12-11-9(7-13-14-12)6-5-8-3-1-2-4-10(8)11/h1-7H,(H,14,15)/f/h14H
InChIKey=BGYAATPHYRJYHZ-YHMJCDSICO

Here is another benchmark of the performance of DFT in handling difficult situations, in this case the interaction between nucleic acid base pairs. Sherrill¹ has examined the 124 nucleic acid base pairs from the JSCH-2005 database² compiled by Hobza and coworkers. This database includes 36 hydrogen bonded complexes, and example of which is shown in Figure 1a, and 54 stacked complex, one example of which is shown in Figure 1b.

(a)

(b)

Figure 1. Optimized geometries (RI-MP2/cc-pVTZ) of two representative structures of base pairs: (a) hydrogen bonded pair and (c) stacked pair.

The energies of these base pairs computed with four different functionals: PBE, PBE-D (where Grimme’s empirical dispersion correction³), and the recently developed MO5-2X⁴ and MO6-2X⁵ methods which attempt to treat mid-range electron correlation. The aug-cc-pVDZ basis set was used. These DFT energies are compared with the CCSD(T) energies of Hobza. The mean unsigned error (MUE) for the 28 hydrogen bonded complexes and the 54 stacked complexes are listed in Table 1.

Table 1. Mean unsigned error (kcal mol^-1) of the four DFT
methods (relative to CCSD(T)) for the hydrogen bonded and stacked base pairs.

A few interesting trends are readily apparent. First, PBE (representing standard GGA DFT methods) poorly describes the energy of the hydrogen bonded complexes, but utterly fails to treat the stacking interaction. Inclusion of the dispersion correction (PBE-D) results in excellent energies for the HB cases and quite reasonable results for the stacked pairs. Both of Truhlar’s functionals dramatically outperform PBE, though MO5-2X is probably still not appropriate for the stacked case. MO6-2X however seems to be a very reasonable functional for dealing with base pair interactions, indicating that mid-range correlation correction is sufficient to describe these complexes, and that the long-range correlation correction included in the dispersion correction, while giving improved results, is not essential.

References

(1) Hohenstein, E. G.; Chill, S. T.; Sherrill, C. D., "Assessment of the Performance of the M05-2X and M06-2X Exchange-Correlation Functionals for Noncovalent Interactions in Biomolecules," J. Chem. Theory Comput., 2008, 4, 1996-2000, DOI: 10.1021/ct800308k

(2) Jurecka, P.; Sponer, J.; Cerny, J.; P., H., "Benchmark database of accurate (MP2 and CCSD(T) complete basis set limit) interaction energies of small model complexes, DNA base pairs, and amino acid pairs," Phys. Chem. Chem. Phys., 2006, 8, 1985-1993, DOI: 10.1039/b600027d.

(3) Grimme, S., "Semiempirical GGA-type density functional constructed with a long-range dispersion correction," J. Comput. Chem., 2006, 27, 1787-1799, DOI: 10.1002/jcc.20495

(4) Zhao, Y.; Schultz, N. E.; Truhlar, D. G., "Design of Density Functionals by Combining the Method of Constraint Satisfaction with Parametrization for Thermochemistry, Thermochemical Kinetics, and Noncovalent Interactions," J. Chem. Theory Comput., 2006, 2, 364-382, DOI: 10.1021/ct0502763.

(5) Zhao, Y.; Truhlar, D. G., "The M06 suite of density functionals for main group thermochemistry, thermochemical kinetics, noncovalent interactions, excited states, and transition elements: two new functionals and systematic testing of four M06-class functionals and 12 other functionals," Theor. Chem. Acc., 2008, 120, 215-241, DOI: 10.1007/s00214-007-0310-x.

Computed optical activity was utilized in establishing the absolute configuration of the [3,3]paracyclophane 1.¹ The helical twist of this molecule makes it chiral.

The specific rotation of (-)-1 was measured to be -123 ° [dm (g/cm³) ^-1]. Seven different conformations of R–1 were optimized, having either D₂, C₂, or C₁ symmetry, at B3LYP/TZVP. The two lowest energy conformers (at B3LYP/6-31G(d) – the authors did not supply coordinates in their supporting materials!) are shown in Figure 1.

1a (0.0)

1b (0.49)

Figure 1. B3LYP/6-31G(d) optimized structures and relative energy (kcal mol^-1 of the two lowest energy conformers of 1.

The TDDFT computed value for [α]_D for the lowest energy conformer is -171.7 ° [dm (g/cm³)^-1]. In fact, the range of [α]_D for seven conformers is -124.4 to -221.8. These values are consistent with the experimental observation in both sign and magnitude. The computed CD spectrum of the seven R–1 conformations are similar to the experimental spectra of (-)-1. Thus, one can conclude that the two enantiomers are R-(-)-1 and S-(+)-1.

References

(1) Muranaka, A.; Shibahara, M.; Watanabe, M.; Matsumoto, T.; Shinmyozu, T.; Kobayashi, N., "Optical Resolution, Absolute Configuration, and Chiroptical Properties of Three-Layered [3.3]Paracyclophane(1)," J. Org. Chem., 2008, 73, 9125-9128, DOI: 10.1021/jo801441h

InChIs

1: InChI=1/C31H38/c1-3-7-28-22-30-12-6-10-27-20-18-26(19-21-27)9-5-11-29(28)23-31(30)13-4-8-25-16-14-24(2)15-17-25/h14-23H,3-13H2,1-2H3
InChIKey=GFTKQZANYFICNS-UHFFFAOYAN