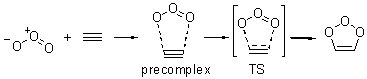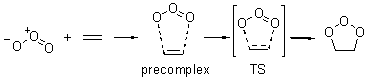The composite methods (exemplified by the Gaussian-n methods, the Weizmann-n methods and CNS-Q methods) are popularly held to be perhaps the best (if not the most straightforward and mechanical) procedures for obtaining accurate energetics. Errors are generally thought to be in the 1-2 kcal mol^-1 range – quite suitable for comparisons with experiment. The recent study of the 1,3-dipolar cycloaddition of ozone with ethene or ethyne offers serious food for thought about considering these composite methods as simple “black-box” solutions towards obtaining good results.
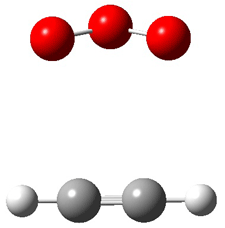
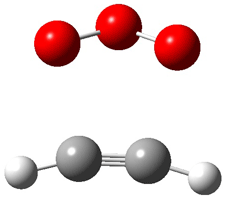
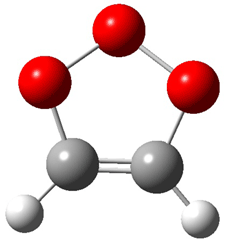
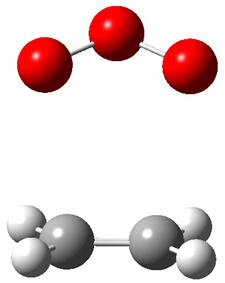
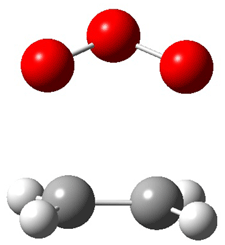
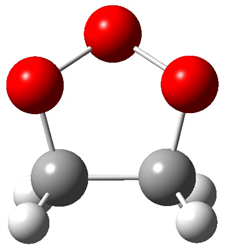
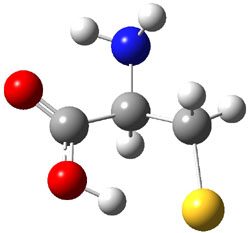
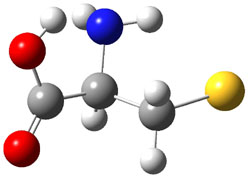
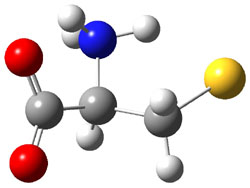
Wheeler, Ess, and Houk have examined the cycloaddition of ozone with ethyne (Reaction 1) and ethane (Reaction 2) with a variety of different computational techniques.¹ Shown in Figure 1 are the CCSD(T)/cc-pVTZ optimized geometries of the pre-complex, transition state and product for both reactions. One of the potential challenges of these reactions is that ozone has appreciable radical character which is also likely to be true in the pre-complex and transition state but the product should have little to no radical character.

Reaction 1
Precomplex	TS	Product
Reaction 2
Precomplex	TS	Product

The relative enthalphies (0K) of the complex, TS and product were computed using a number of different composite methods. The CBS-QB3, G3, G3B3, G3MP2B3 and G4^2,3 results are listed in Table 1 for both reactions. All of these methods claim a small error – perhaps 1-2 kcal mol^-1. The Gaussian-n methods nicely cluster for the reaction energy for both reactions, while CBS-QB3 predicts both reactions are more exothermic. (The same is also true of CBS-APNO.) The G-n methods indicate that the precomplex is essentially unbound. More concerning is the spread in the enthalpy values of the reaction barrier: the G-n methods predict a barrier that ranges over 5 kcal mol^-1.

Table 1. Relative enthalpies (kcal mol^-1) of the critical points for Reactions 1 and 2.¹

So what is the correct barrier height? A focal point extrapolation procedure was then performed. This seeks to extrapolate to infinite basis set and estimate the effects of correlation through CCSDT(Q) and includes corrections for the Born-Oppenheimer approximation and special relativistic effects. The focal point results are also shown in Table 1. The new G4 composite method gives enthalpies in very nice agreement with those obtained with the much more expensive focal point procedure. However, the other composite methods fair much worse, especially for the activation barrier.
The convergence of the focal point method for the reaction energy is slow, leading to a large error bar of 2 kcal mol^-1. This appears to relate to the radical character difference between the reactant and product. The convergence for the activation barrier is much better (an error of only 0.2 kcal mol^-1) and here the comparison is between reactant and TS which have similar radical character.
Perhaps the most discouraging aspect of this study is that the relative energy predicted using the highest computational component of the composite method – so, CCSD(T)/6-31+G* for the CBS-QB3 method and QCISD(T)/6-31G(d) for the G3 methods – are more accurate than the composite method itself. But, the reasonable performance of the new G4 method^2,3 does offer some glimmer of hope here.

References

(1) Wheeler, S. E.; Ess, D. H.; Houk, K. N., "Thinking
Out of the Black Box: Accurate Barrier Heights of 1,3-Dipolar Cycloadditions of Ozone with Acetylene and Ethylene," J. Phys. Chem. A, 2008, 112, 1798-1807, DOI: 10.1021/jp710104d.

(2) Curtiss, L. A.; Redfern, P. C.; Raghavachari, K., "Gaussian-4 theory," J. Chem. Phys., 2007, 126, 084108, DOI: 10.1063/1.2436888

(3) Curtiss, L. A.; Redfern, P. C.; Raghavachari, K., "Gaussian-4 theory using
reduced order perturbation theory," J. Chem. Phys., 2007, 127, 124105-8, DOI: 10.1063/1.2770701.

InChIs

Reaction 1 product: InChI=1/C2H2O3/c1-2-4-5-3-1/h1-2H

Reaction 2 product: InChI=1/C2H4O3/c1-2-4-5-3-1/h1-2H2

Kass has once again uncovered a simple system that challenges our notions of basic chemical concepts. It is a well accepted notion that the most acidic proton of all of the amino acids is the carboxylic acid one. However, acidities are strongly influenced by the solvent, and the absence of solvent in the gas phase can dramatically alter things.

Kass and co-workers examined the gas-phase acidity of cysteine with computational and
experimental techniques.¹ The lowest energy conformer of cysteine is 1a, characterized by having three intramolecular hydrogen bonds (Figure 1). The next lowest conformer, 1b, has only two intramolecular hydrogen bonds and is 1.5 kcal mol^-1 higher in energy at G3B3.

1a xyz	1b xyz

Figure 1. B3LYP/aug-cc-pVDZ optimized structures of cysteine 1.¹

They optimized a number of different configurations of the conjugate base of cysteine: two conformers from the loss of the carboxylate proton (2a and 2b), two conformers from the loss of the thiol proton (2c and 2d), and one conformer from the loss of the thiol proton of the zwitterion (2e). These structures are shown in Figure 2 along with their relative energies. All of these structures possess two intramolecular hydrogen bonds.

2a (3.1) xyz	2b (3.4) xyz
2c (0.0) xyz	2d (5.1) xyz
2e (10.1) xyz

Figure 2. B3LYP/aug-cc-pVDZ optimized structures of the conjugate base of cysteine 2. Relative energies (kcal mol^-1) in parenthesis computed at G3B3.¹

The gas phase acidity of carboxylic acids is greater than thiols; the deprotonation energy of propanoic acid (CH₃CH₂CO₂H) is 347.7 kcal mol^-1 at G3B3 (347.2 expt.²), about 6 kcal mol^-1 less than that of ethanethiol (CH₂CH₂SH: 355.0 at G3B3 and 354.2 expt.²). However, the computations indicate that 2c is the lowest energy structure of deprotonated cysteine, and 2c comes about by loss of the thiol proton! Te lowest energy cysteine conjugate base from loss of the carboxylate proton is 1a, which is 3.1 kcal mol^-1 higher in energy. Apparently, the hydrogen bonding network in 2c is quite favorable, able to make up for the inherent favorability of a carboxylate over a thiolate anion.

The G3B3 computed deprotonation energy of cysteine is 333.3 kcal mol^-1 (for removal of the thiol proton). Kass determined the deprotonation energy of cysteine using a kinetic and a thermodynamic method. The kinetic method gives a value of 332.9 ± 3.3 kcal mol^-1­, while the thermodynamic method gives 334.4 ± 3.3 kcal mol^-1­. These are in fine agreement with the computed value.

This study ably demonstrates the dramatic role that solvent can play in determining molecular properties. Kass titled the article “Are carboxyl groups the most acidic sites in amino acids?” and answers with “no” – in the gas phase the thiol group is more acidic. He ends the article with an indication that the alcohol of tyrosine may be competitive in acidity with its carboxylic group, too.

References

(1) Tian, Z.; Pawlow, A.; Poutsma, J. C.; Kass, S. R., "Are Carboxyl Groups the Most Acidic Sites in Amino Acids? Gas-Phase Acidity, H/D Exchange Experiments, and Computations on Cysteine and Its Conjugate Base," J. Am. Chem. Soc., 2007, 129, 5403-5407, DOI: 10.1021/ja0666194.

(2) NIST, NIST Chemistry WebBook, 2005, http://webbook.nist.gov/.

InChIs

1: InChI=1/C3H7NO2S/c4-2(1-7)3(5)6/h2,7H,1,4H2,(H,5,6)

In Chapter 2.2, we suggest that the experimental deprotonation energy (DPE) of cyclohexane is in doubt. G2MP2 predicts the DPE of cyclohexane is 414.5 kcal mol^-1, a figure significantly higher than the experimental¹ value of 404 kcal mol^-1. Given that the deviation between the G2MP2 computed DPE and experiment is about 2 kcal mol^-1, we suggest that cyclohexane should be re-examined.

In a recent JACS article,² Kass calls into question the experimental bond dissociation energies (BDE) of the small cycloalkanes. With his experimental determination of the BDE of both the vinyl and allylic positions of cyclobutene, Kass can compare experimental and computed BDEs for a range of hydrocarbon environments, as listed in Table 1. The two composite methods G3 and W1 provide excellent BDE values for the small alkanes, one acyclic alkene, and the small cyclic alkenes. These composite methods appear to accurately predict BDEs of hydrocarbons.

However, the small cyclic alkanes are dramatic outliers. The well-accepted experimental BDEs of cyclopropane, cyclobutane, and cyclohexane are 3-5 kcal mol^-1 lower than those predicted by the composite methods. Given the strong performance of the computational methods, and the difficulties associated with experimental determinations of BDEs, Kass suggests that the BDEs of these cycloalkanes are in error. Further experiments are deserved.

References

(1) NIST webbook, 2005, http://webbook.nist.gov/.

(2) Tian, Z.; Fattahi, A.; Lis, L.; Kass, S. R., "Cycloalkane and Cycloalkene C-H Bond Dissociation Energies," J. Am. Chem. Soc. 2006, 128, 17087-17092, DOI: 10.1021/ja065348u

(3) Yao, Y.-R. Handbook of Bond Dissociation Energies in Organic Compounds; CRC Press: Boca Raton, FL, 2003.

(4) CRC Handbook of Chemistry and Physics; 85th ed.; Lide, D. R., Ed.; CRC Press: Boca Raton, FL, 2004.

(5) Tsang, W. In Energetics of Stable Molecules and Reactive Intermediates, NATO Science Series C; Minas da Piedade, M. E., Ed.; Kluwer Academic Publishers: Dordrecht, Netherlands, 1999; Vol. 535.

(6) Fattahi, A.; McCarthy, R. E.; Ahmad, M. R.; Kass, S. R., "Why Does Cyclopropene Have the Acidity of an Acetylene but the Bond Energy of Methane?," J. Am. Chem. Soc. 2003, 125, 11746-11750, DOI: 10.1021/ja035725s.

(7) DeFrees, D. J.; McIver, R. T., Jr.; Hehre, W. J., "Heats of Formation of Gaseous Free Radicals via Ion Cyclotron Double Resonance Spectroscopy," J. Am. Chem. Soc. 1980, 102, 3334-3338, DOI: 10.1021/ja00530a005.

(8) Furuyama, S.; Golden, D. M.; Benson, S. W., "Kinetic Study of the Gas-Phase Reaction c-C₅H₈+I₂ c-C₅H₆+2HI. Heat of Formation and the Stabilization Energy of the Cyclopentenyl Radical," Int. J. Chem. Kinet. 1970, 2, 93-99.

(9) Alfassi, Z. B.; Feldman, L., "The Kinetics of Radiation-Induced Hydrogen Abstraction
by Trichloromethyl Radicals in the Liquid Phase: Cyclohexene," Int. J. Chem. Kinet. 1981, 13, 771-783.