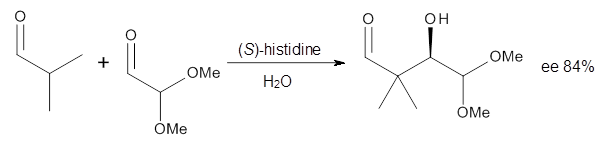
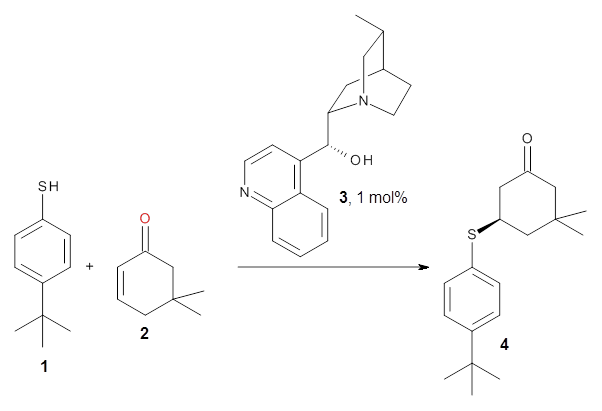
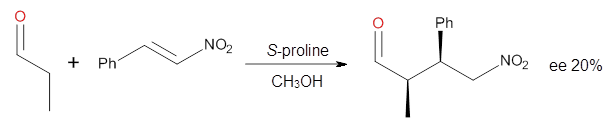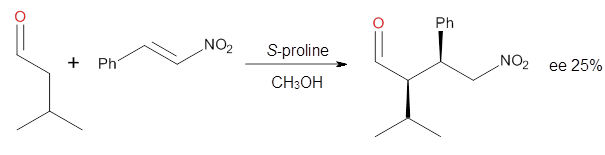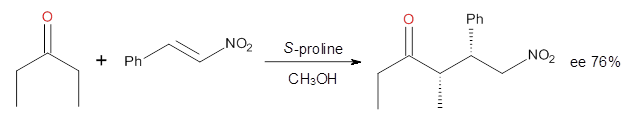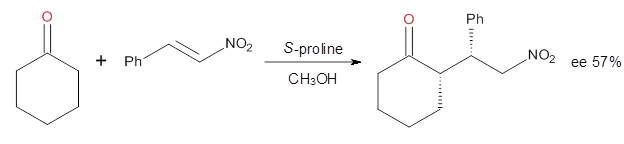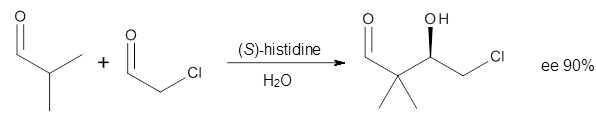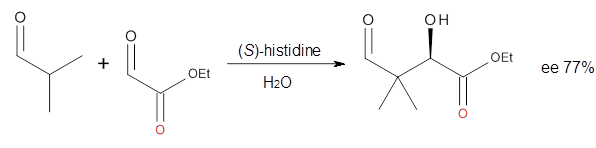Cinchona alkaloids cat catalyze reactions, such as shown in Reaction 1. Wynberg¹ proposed a model to explain the reaction, shown in Scheme 1, based on NMR. Grayson and Houk have now used DFT computations to show that the mechanism actually reverses the arrangements of the substrates.²

Reaction 1

Scheme 1.

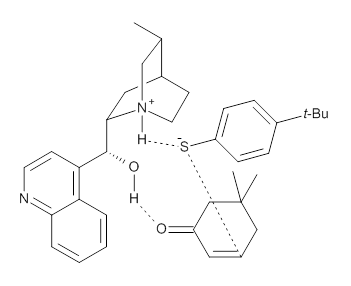
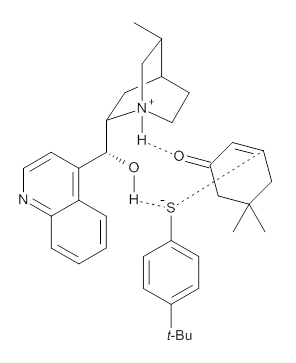
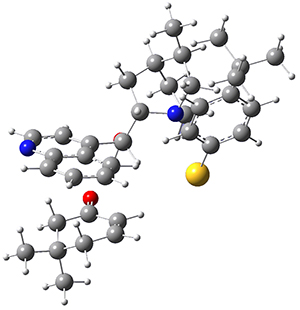
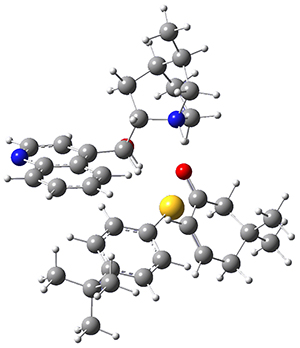
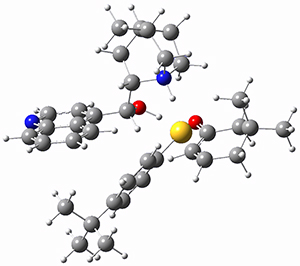
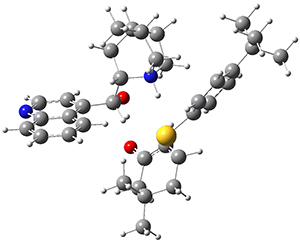
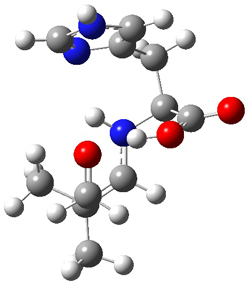
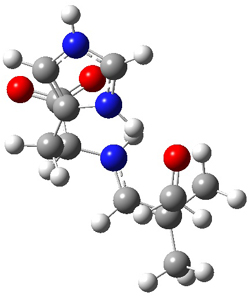
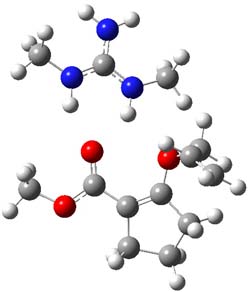
M06-2X/def2-TZVPP−IEFPCM(benzene)//M06-2X/6-31G(d)−IEFPCM(benzene) computations show that the precomplex of catalyst 3 with nucleophile 1 and Michael acceptor 2 is consistent with Wynberg’s model. The alternate precomplex is 5.6 kcal mol^-1 higher in energy. These precomplexes are shown in Figure 1.

Wynberg precomplex

Grayson/Houk precomplex

Figure 1. Precomplexes structures

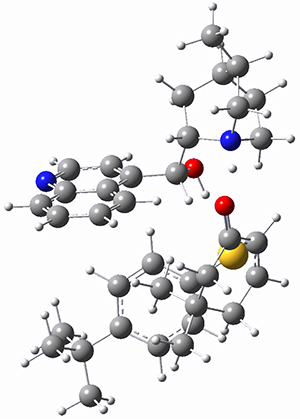
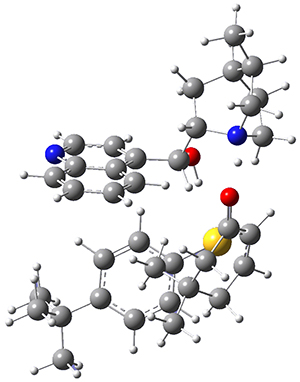
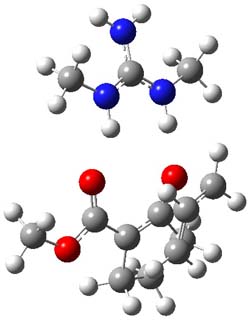
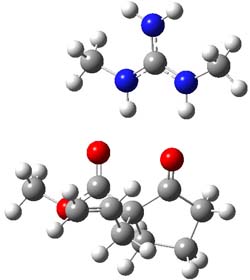
However, the lowest energy transition state takes the Grayson/Houk pathway and leads to the major isomer observed in the reaction. The Grayson/Houk TS that leads to the minor product has a barrier that is 3 kcal mol^-1 higher in energy. The lowest energy TS following the Wynberg path leads to the minor product, and is 2.2 kcal mol^-1 higher than the Grayson/Houk path. These transition states are shown in Figure 2. The upshot is that complex formation is not necessarily indicative of the transition state structure.

Wynberg TS (major) Rel ΔG = 5.3	Wynberg TS (minor) Rel ΔG = 2.2
Grayson/Houk TS (major) Rel ΔG = 0.0	Grayson/Houk TS (minor) Rel ΔG = 3.0

Figure 2. TS structures and relative free energies (kcal mol^-1).

References

(1) Hiemstra, H.; Wynberg, H. "Addition of aromatic thiols to conjugated cycloalkenones, catalyzed by chiral .beta.-hydroxy amines. A mechanistic study of homogeneous catalytic asymmetric synthesis," J. Am. Chem. Soc. 1981, 103, 417-430, DOI: 10.1021/ja00392a029.

(2) Grayson, M. N.; Houk, K. N. "Cinchona Alkaloid-Catalyzed Asymmetric Conjugate Additions: The Bifunctional Brønsted Acid–Hydrogen Bonding Model," J. Am. Chem. Soc. 2016, 138, 1170-1173, DOI: 10.1021/jacs.5b13275.

InChIs

1: InChI=1S/C10H14S/c1-10(2,3)8-4-6-9(11)7-5-8/h4-7,11H,1-3H3
InChIKey=GNXBFFHXJDZGEK-UHFFFAOYSA-N

2: InChI=1S/C8H12O/c1-8(2)5-3-4-7(9)6-8/h3-4H,5-6H2,1-2H3
InChIKey=CDDGRARTNILYAB-UHFFFAOYSA-N

3: InChI=1S/C18H22N2O/c1-12-11-20-9-7-13(12)10-17(20)18(21)15-6-8-19-16-5-3-2-4-14(15)16/h2-6,8,12-13,17-18,21H,7,9-11H2,1H3/t12?,13?,17?,18-/m1/s1
InChIKey=ZOZLJWFJLBUKKL-NKHWWFDVSA-N

4: InChI=1S/C18H26OS/c1-17(2,3)13-6-8-15(9-7-13)20-16-10-14(19)11-18(4,5)12-16/h6-9,16H,10-12H2,1-5H3/t16-/m0/s1
InChIKey=XUTYYZOSKLYWLW-INIZCTEOSA-N

Here are a couple of articles describing computational approaches to catalytic enantioselective reactions using variations upon the classic proline-catalyzed aldol reaction of List and Barbas¹ that started the whole parade. I have discussed the major computational papers on that system in my book (Chapter 5.3).

Yang and Wong² investigated the proline-catalyzed nitro-Michael reaction, looking at four examples, two with aldehydes and two with ketones (Reactions 1-4).

These four reactions were examined atMP2/311+G**//M06-2x/6-31G**, and PCM was also applied. The key element of this study is that they examined two different types of transition states: (a) based on the Houk-List model involving a hydrogen bond and (b) an electrostatic based model with no hydrogen bond. These are sketched in Scheme 1. For each of the reactions 1-4 there are 8 located transition states differing in the orientation of the attack on to the syn or anti enamine.

Scheme 1. TS models

The two lowest energy TS are shown in Figure 1. TS1-β1-RS is the lowest TS and it leads to the major enantiomer. The second lowest TS, TS1-β3-SR, lies 2.9 kJ mol^-1 above the other TS, and it leads to the minor enantiomer. This lowest TS is of the Houk-List type (Model A) while the other TS is of the Model B type. The enthalpies of activation suggest an ee of 54%, in reasonable
agreement with experiment.

TS1-β1-RS

TS1-β1-RS

Figure 1. M06-2x/6-31G** optimized geometries of TS1-β1-RS and TS1-β1-RS.

The computations of the other three reactions are equally good in terms of agreement with experiment, and importantly the computations indicate the reversal of stereoselection between the aldehydes and the ketones. These computations clearly implicate both the Houk-List and the non-hydrogen bonding TSs in the catalyzed Michael additions.

Houk in collaboration with Scheffler and Mahrwald investigate the use of histidine as a catalyst for the asymmetricaldol reaction.³ Examples of the histidine-catalyzed aldol are shown in Reactions 5-7.

	Reaction 5
	Reaction 6
	Reaction 7

The interesting twist here is whether the imidazole can also be involved in hydrogen bonding to the acceptor carbonyl group, serving the purpose of the carboxylic acid group in the Houk-List TS when proline is the catalyst (Model A). The transition states for these and a few other reactions were computed at M06-2x/6-31+G(d,p) including with the SMD continuum solvent model for water. The two lowest energy TSs for the reaction of isobutyraldehde and formaldehyde are shown in Figure 2; TS1 has the carboxylic acid group as the hydrogen donor while the imidazole is the donor in TS2. Of note is that these two TS are isoenergetic, indicating that both modes of stabilization are at play with histidine as the catalyst.

TS1

TS2

Figure 2. M06-2x/6-31+G(d,p) geometries of the two lowest energy TSs for the reaction of isobutyraldehde and formaldehyde catalyzed by histidine.

The possible TSs for Reactions 5-7 were also located. For example, with Reaction 5, the lowest energy TS involves the imidazole as the hydrogen donor and it leads to the major product. The lowest energy TS that leads to the minor product involves the carboxylic acid as the donor. The computed ee’s for Reactions 5-7 are in very good, if not excellent, agreement with the experimental values. The study should spur further activity in which one might tune the stereoselectivity by using catalysts with multiple binding opportunities.

References

(1) List, B.; Lerner, R. A.; Barbas, C. F., III; "Proline-Catalyzed Direct Asymmetric Aldol Reactions," J. Am. Chem. Soc., 2000, 122, 2395-2396, DOI: 10.1021/ja994280y.

(2) Yang, H.; Wong, M. W. "(S)-Proline-catalyzed nitro-Michael reactions: towards a better understanding of the catalytic mechanism and enantioselectivity," Org. Biomol. Chem.,
2012, 10, 3229-3235, DOI: 10.1039/C2OB06993H

(3) Lam, Y.-h.; Houk, K. N.; Scheffler, U.; Mahrwald, R. "Stereoselectivities of Histidine-Catalyzed Asymmetric Aldol Additions and Contrasts with Proline Catalysis: A Quantum Mechanical Analysis," J. Am. Chem. Soc. 2012, 134, 6286-6295, DOI: 10.1021/ja2118392

Jacobsen reports another interesting example of organocatalysis, here using a chiral guanadinium salt to catalyze the enantioselective Claisen rearrangement.¹ As an example, Reaction 1 proceeds in 6 days at 30 °C to give 81% yield with an ee of 84%. The system is also diastereoselective, so that Reaction 2, run for 6 days at 40 °C, gives an 82% yield with a diastereomeric ratio of 16:1 and an ee of 81%.

B3LYP/6-31G(d,p) computations provide some insight. The uncatalyzed reaction of 1 to give 2 is predicted to be exothermic by 16.1 kcal mol^-1, with an activation energy of 25.9 kcal mol^-1. Using N,N’-dimethylguanidnium as a model for the catalyst (and with no counter anion and no treatment of solvent – hexanes in this case), they find a complexation energy of almost 27 kcal mol^-1 for forming 3. 3 exhibits (See Figure 1) three hydrogen bond-like interactions – one N-H bifurcates to interact with the carbonyl oxygen and (a very long interaction) to the other oxygen. The product complex 4 also shows three hydrogen bond-like interactions, with an overall exothermicity of -14.7 kcal mol^-1. The complexed transition state 5 has two normal length hydrogen bonds, with an activation energy above 3 of 20.6 kcal mol^-1. Thus the complex lowers the barrier by about 5 kcal mol^-1, indicating the catalytic effect. They have not however addressed the enantioselectivity.

3	5
4

Figure 1. B3LYP/6-31G(d,p) optimized geometries of 3-5.

References

(1) Uyeda, C.; Rötheli, A. R.; Jacobsen, E. N., "Catalytic Enantioselective Claisen Rearrangements of O-Allyl β-Ketoesters," Angew. Chem. Int. Ed., 2010, 49, 9753–9756, DOI: 10.1002/anie.201005183

InChIs

1: InChI=1/C10H14O3/c1-3-7-13-9-6-4-5-8(9)10(11)12-2/h3H,1,4-7H2,2H3
InChIKey=NASFSRKGDOBHIX-UHFFFAOYAC

2: InChI=1/C10H14O3/c1-3-6-10(9(12)13-2)7-4-5-8(10)11/h3H,1,4-7H2,2H3/t10-/m0/s1
InChIKey=QXKXLNGEBVMWLH-JTQLQIEIBT

What gives rise to the face selectivity in the epoxidation of the alkene of 1 and 2? And why is the epoxidation of 3 of opposite selectivity? Williams¹ argues that the stereoinduction is due to distortional asymmetry, an argument similar to one made recently by Houk^2,3 (see this post) and others for cycloaddition reactions.

The major conclusion from this paper is drawn from the potential energy curve that results from out-of-plane bending of the alkenyl hydrogens, as in Figure 1. The bending curves (computed at B3LYP/6-31g(2d,2p)//B3LYP/6-31+G(d))) are asymmetric: bending the hydrogens away from the three-member ring requires less energy than bending them towards the cyclopropyl ring. However, for 3, bending in the two directions is pretty similar, with a slight preference for bending towards the four-member ring.

Fig. 1 Energy (kcal mol^-1) vs distortion angle of alkenyl hydrogens

This type of bending is part of the distortions that have to occur to reach the transition state, and so Williams argues that the attack from the cyclopropyl face by the oxidant is preferred because of the easier geometric distortion of moving the hydrogen away. Williams makes standard orbital interaction arguments to rationalize the distortion preference.

References

(1) Kolakowski, R. V.; Williams, L. J., "Stereoinduction by distortional asymmetry," Nat. Chem. 2010, 2, 303-307, DOI: 10.1038/nchem.577.

(2)
Xu, L.; Doubleday, C. E.; Houk, K. N., "Dynamics of 1,3-Dipolar Cycloaddition Reactions of Diazonium Betaines to Acetylene and Ethylene: Bending Vibrations Facilitate Reaction," Angew. Chem. Int. Ed. 2009, 48, 2746-2748, DOI: 10.1002/anie.200805906

(3) Xu, L.; Doubleday, C. E.; Houk, K. N., "Dynamics of 1,3-Dipolar Cycloadditions: Energy Partitioning of Reactants and Quantitation of Synchronicity," J. Am. Chem. Soc., 2010, 132, 3029–3037, DOI: http://dx.doi.org/10.1021/ja909372f

InChIs

1: InChI=1/C9H12/c1-2-7-4-3-6(1)8-5-9(7)8/h1-2,6-9H,3-5H2
InChIKey=YNSKHNKUOPTLCL-UHFFFAOYAA

2: InChI=1/C10H11N/c11-5-8-9-6-1-2-7(4-3-6)10(8)9/h1-2,6-10H,3-4H2
InChIKey=XHTNELKCCFLXEU-UHFFFAOYAJ

3: InChI=1/C10H14/c1-2-8-4-3-7(1)9-5-6-10(8)9/h1-2,7-10H,3-6H2
InChIKey=OYPVZSANECKQOK-UHFFFAOYAR