I think most organic chemists hold dear to their hearts the notion that selectivity is due to crossing over different transition states. Readers of my book and this blog know of the many examples where this notion simply is not true (see here). This post discusses yet another example taking place in a seemingly simple reaction.

Singleton has examined the nucleophilic substitution reaction of 1 with sodium tolylsulfide.¹ The mono substitution gives potentially two different stereoproducts 2 and 3. The experimental ratio of these products 2:3 is 81:19. (Note that things are a bit more complicated because disubstitution can also occur, but this has been factored into the product ratio.)

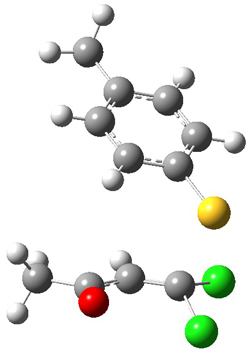
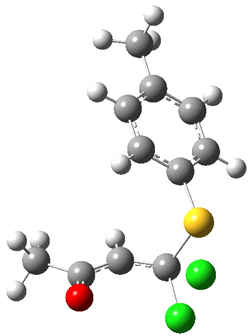
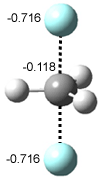
Based on previous literature, this reaction is likely to proceed in a concerted fashion, and so one might anticipate running computations to locate a transition state leading to 2 and a transition state leading to 3. In fact, Singleton finds six different TSs (the lowest energy TS 4 is shown in Figure 1), all within 2 kcal mol^-1 of each other at PCM(ethanol)/B3LYP/6-31+G**. However, the intrinsic reaction coordinate going forward from each of these six TSs leads solely to 2; no TS could be located that connects to 3! (Computations were also performed at PCM(ethanol)/M06-2x/6-31+G** which give very similar results.) Classical transition state theory would lead
one to conclude that only 2 should be formed, inconsistent with experiment.

4

5

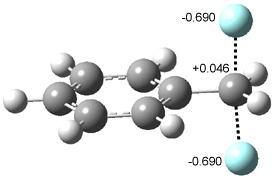
Figure 1. PCM/B3LYP/6-31+G** optimized structures of TSs 4 and 5.

Furthermore, no intermediate could be located. This is consistent with a concerted mechanism. A second transition state was located which interconverts 2 and 3 with the involvement of a chloride – a sort of addition/rotation/elimination process. This TS 5 is also shown in Figure 1.

A direct dynamics study was performed, and 197 trajectories were computed. Of these, 185 trajectories went to product: 156 to 2 and 29 to 3, for a ratio of 84:16 – in amazing agreement with experiment! The product selectivity is due entirely to dynamic effects. In fact, it is one vibrational mode that dictates the product distribution. Essentially, the nature of the rotation about the C=C bond differentiates the eventual route, with a clockwise rotation leading always to 2 and a counterclockwise rotation leading about a third of the time to 3.

References

(1) Bogle, X. S.; Singleton, D. A. "Dynamic Origin of the Stereoselectivity of a Nucleophilic Substitution Reaction," Org. Lett., 2012, 14, 2528-2531, DOI: 10.1021/ol300817a.

InChIs

1: InChI=1S/C4H4Cl2O/c1-3(7)2-4(5)6/h2H,1H3
InChIKey=NXDUHPYJFYSBCT-UHFFFAOYSA-N

2: InChI=1S/C11H11ClOS/c1-8-3-5-10(6-4-8)14-11(12)7-9(2)13/h3-7H,1-2H3/b11-7-
InChIKey=NCXXSKTZGJETLW-XFFZJAGNSA-N

3: InChI=1S/C11H11ClOS/c1-8-3-5-10(6-4-8)14-11(12)7-9(2)13/h3-7H,1-2H3/b11-7+
InChIKey=NCXXSKTZGJETLW-YRNVUSSQSA-N

Reactions whose outcomes depend on dynamic processes is a major theme of my book and this blog. The recent study of the reaction of a nucleophile (hydroxide) with bromoacetophenones adds yet another case for post-transition state product determination.

Itoh and Yamataka have examined the reaction of hydroxide with substitutes α-bromoacetophenones 1.¹ The nucleophile can attack at the carbonyl carbon or the α-carbon, though both lead ultimately to the same product, as shown in Scheme 1.

Scheme 1

B3LYP/6-31+G* computations of the reaction surface with a variety of different substituents on the phenyl ring of 1 located in all cases a single transition state for the two different reactions (addition and substitution). This TS is shown in Figure 1 for the parent case (X=H).

Figure 1. The single transition state for the addition and substitution reaction of 1 and hydroxide.

Tracing the IRC forward leads to either the carbonyl addition product or the substitution product, and which path is traced depends to some extent on the nature of the substituent. Most intriguing is that trajectories initiated at the transition state lead to both products. So once again, we see a case where a single transition state leads to two products, and product selectivity is determine by the dynamics – the initial conditions at the TS dictate which of the two products is eventually obtained.

References

(1) Itoh, S.; Yoshimura, N.; Sato, M.; Yamataka, H., "Computational Study on the Reaction Pathway of α-Bromoacetophenones with Hydroxide Ion: Possible Path Bifurcation in the Addition/Substitution Mechanism," J. Org. Chem., 2011, 70, 8294–8299, DOI: 10.1021/jo201485y

Schaefer and Allen have applied their focal point method to the question of the benzylic effect in the S_N2 reaction.¹ S_N2 reactions are accelerated when the attack occurs at the benzylic carbon, a well-known phenomenon yet the reason for this remains unclear. The standard textbook-like argument has been that the negative charge built up in the S_N2 transition state can be delocalized into the phenyl ring. However, solution phase Hammett studies are often U-shaped, indicating that both electron donating and withdrawing group accelerate the substitution reaction. (This is usually argued as indicative of a mechanism change from S_N2 to S_N1.)

The focal point method involves a series of very large computations where both basis set size and degree of electron correlation are systematically increased, allowing for an extrapolation to essentially infinite basis set and complete correlation energy. The energy of the transition state (relative to separated reactants) for four simple S_N2 reactions evaluated with the focal point method are listed in Table 1. The barrier for the benzylic substitutions is lower than for the methyl cases, indicative of the benzylic effect.

Table 1. Energy (kcal mol^-1) of the transition state relative to reactants.¹

To answer the question of why the benzylic substitution reactions are faster, they examined the charge distribution evaluated at B3LYP/DZP++. As seen in Table 1, this method does not accurately reproduce the activation barriers, but the errors are not terrible, and the trends are correct.

In Figure 1 are the geometries of the transition states for the reaction of fluoride with methylflouride or benzylfluoride. The NBO atomic charges show that the phenyl ring acquired very little negative charge at the transition state. Rather, the electric potential at the carbon under attack is much more revealing. The potential is significantly more positive for the benzylic carbon than the methyl carbon in both the reactant and transition states.

VC = -405.156 V	VC = -404.379 V

Figure 1. MP2/DZP++ transition states for the reaction of fluoride with methylfluoride and benzylflouride. NBO charges on F and C and the electrostatic potential in Volts.¹

They next examined the reaction of fluoride with a series of para-substituted benzylfluorides. The relation between the Hammet σ constants and the activation energy is fair (r = 0.971). But the relation between the electrostatic potential at the benzylic carbon (in either the reactant or transition state) with the activation energy is excellent (r = 0.994 or 0.998). Thus, they argue that it is the increased electrostatic potential at the benzylic carbon that accounts for the increased rate of the S_N2 reaction.

References

(1) Galabov, B.; Nikolova, V.; Wilke, J. J.; Schaefer III, H. F.; Allen, W. D., "Origin of the S_N2 Benzylic Effect," J. Am. Chem. Soc., 2008, 130, 9887-9896, DOI: 10.1021/ja802246y.

Bickelhaupt has reported a broad benchmark study of the prototype S_N2 and E2 reactions.¹ These are the reactions of ethyl fluoride with fluoride and ethyl chloride with chloride (Scheme A). The critical points were optimized at OLYP/TZ2P and then CCSD(T)/CBS energies are used as benchmark. A variety of different density functionals were then used to obtain single-point energies.

Scheme A

The relative energies of the transition states for the six different reactions are listed for some of the functionals in Table 1. (These are energies relative to separated reactants – and keep in mind that an ion dipole complex is formed between the reactants and the transition states – Bickelhaupt calls this a “reaction complex”.)

Table 1. Relative energies (kcal mol^-1) of the transition states for the six reactions shown in Scheme A.

There is a lot more data in this paper, along with a summary of the mean absolute errors in the overall and central barriers that mimics the data I show in Table 1. The trends are pretty clear. Generalized gradient approximation (GGA) functions – like BLYP, PW91, and PBE – dramatically underestimate the barriers. The hybrid functionals perform much better. The recently maligned B3LYP functional gets the correct trend and provides reasonable estimates of the barriers. Truhlar’s MO5-2X and MO6-2X functionals do very well in matching up the barrier heights along with getting the correct trends in the relative barriers. Simply looking for the functional with the lowest absolute error is not sufficient; BHandH and MO6-L have small errors but give a wrong trend in barriers, predicting that the S_N2 reaction is preferred over the E2 for the fluoride reaction.

Reference

(1) Bento, A. P.; Sola, M.; Bickelhaupt, F. M., "E2 and S_N2 Reactions of X^– + CH₃CH₂X (X = F, Cl); an ab Initio and DFT Benchmark Study," J. Chem. Theory Comput., 2008, 4, 929-940, DOI: 10.1021/ct700318e.

I guess one can never know enough about the S_N2 reaction! Wester and co-workers have performed careful crossed molecular beam imagining on the reaction Cl^– + CH₃I.¹ In collaboration with Hase, they have employed MP2/ECP/aug-cc-pVDZ computations to get the potential energy surface for the reaction and direct molecular dynamics. The PES is exactly as one would expect for a gas phase ion-molecule reaction: the transition state has backside attack of the nucleophile and it connects to two ion-dipole complexes (see Chapter 5.1.1).

The experiments are interpreted with the help of the MD computations. At low energy one sees formation of the complex. At higher energies, the direct backside attack reaction occurs. And at higher energies a new reaction path emerges, as sketched out in Figure 1. As the nucleophile (chloride) approaches methyl iodide, the methyl group rotates towards the nucleophile. The methyl group then collides with the nucleophile, which sends the methyl group spinning about the iodine atom in the opposite direction. The methyl group rotates all the way around the iodine atom and when it approaches the chloride a second time, the displacement reaction occurs and product is formed. They term this process a “roundabout mechanism”, and they have some experimental evidence for the occurrence of the double roundabout (two rotations of the methyl group about the iodine)! I think we should anticipate seeing more and more interesting reaction pathways as experimental and theoretical techniques continue to allow us a more detailed and precise view of motion of individual molecules across barriers.

Figure 1. Schematic of the trajectory illustrating the roundabout mechanism.
Chlorine is yellow, iodine is pink and carbon is black.

References

(1) Mikosch, J.; Trippel, S.; Eichhorn, C.; Otto, R.; Lourderaj, U.; Zhang, J. X.; Hase, W. L.; Weidemüller, M.; Wester, R., "Imaging Nucleophilic Substitution Dynamics," Science 2008, 319, 183-186, DOI: 10.1126/science.1150238.

Just a short update here. In Chapter 5.1.2 we discuss nucleophilic substitution at heteroatoms. Unlike the paradigmatic case for substitution at carbon, which proceeds via the S_N2 mechanism. Nucleophilic substitution at second-row atoms (S, Si, P) appears to follow an addition-elimination pathway. Bickelhaupt¹ now adds a more thorough computational examination of nucleophilic substitution at phosphorus. He looked at a few identity reactions involving tricoordinate P, namely

X^– + PH₂X → PH₂X + X^–

X^– + PF₂X → PF₂X + X^–

X^– + PCl₂X → PCl₂X + X^–

where X is chloride or hydroxide. In all cases the only critical point located on the potential energy surface is for a tetracoordinate intermediate. Shown in Figure 1 are the intermediates for the reaction OH^– + PH₂OH and Cl^– + PCl₃. This result is consistent with the studies of nucleophilic substitution at sulfur and silicon.

(a) xyz file

(b) xyz file

Figure 1. OLYP/TZ2P optimized intermediate for the reaction (a) OH^– + PH₂OH
and (b) Cl^– + PCl₃.

References:

(1) vanBochove, M. A.; Swart, M.; Bickelhaupt, F. M., "Nucleophilic Substitution at Phosphorus (S_N2@P): Disappearance and Reappearance of Reaction Barriers," J. Am. Chem. Soc. 2006, 128, 10738-10744, DOI: 10.1021/ja0606529