Biosynthesis of ladder polyethers is the topic of a very nice experimental/computational study by Chen and Houk.¹ The x-ray structure of the enzyme that catalyzes the nucleophilic attack on epoxides to create the 6-member ring ether was determined, but the geometry did not completely indicate the mechanism.

Gas phase computations of the 5-exo-tet and 6-endo-tet ring openings of 1 were examined for both the acid and base catalyzed routes at B2PLYP/6-311++G(d,p)//B2PLYP/6-31G(d).
The results are summarized in Figure 1. Basically, as expected by Baldwin’s rules, the closure to the tetrahydrofuran (5-exo-tet) is favored under both catalyzed conditions. However, the preference is small under base conditions, with the difference in the free energy of activation of only 1.2 kcal mol^-1.

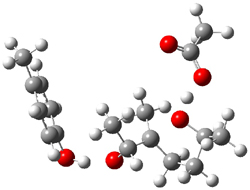
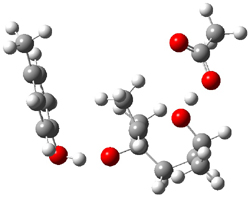
Figure 1. Gas phase energies (kcal mol^-1) for the acid an base catalyzed reactions of 1 to 2 or 3.

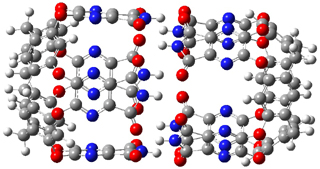
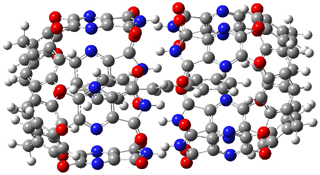
The enzyme Lsd19B produces just the analogue of 3. So, the two regioisomeric TSs were reoptimized with an aspartic acid group and a tyrosine group in the positions they occupy in the active site of the enzyme Lsd19b. The two resulting transition states, evaluated at B2LYP/6-311++G(d,p)//MO6-2x/6-31G(d), are shown in Figure 2. The activation energy for the 6-endo-tet reaction is 18.0 kcal mol^-1, 2.5 kcal mol^-1 lower than for the 5-exo-tet route. This energy difference would give rise to a 100:1 selectivity for the tetrahydropyran product, in accord with experiment. The enzyme preorganizes for and favors the base catalyzed path that leads to 3.

5-exo-tet TS model ΔG‡ = 20.5

6-endo-tet TS model ΔG‡ = 18.0

Figure 2. Transition state models of the active site. Activation energies in kcal mol^-1.

References

(1) Hotta, K.; Chen, X.; Paton, R. S.; Minami, A.; Li, H.; Swaminathan, K.; Mathews, I. I.; Watanabe, K.; Oikawa, H.; Houk, K. N.; Kim, C.-Y., "Enzymatic catalysis of anti-Baldwin ring closure in polyether biosynthesis," Nature, 2012, 483, 355-358, DOI: 10.1038/nature10865.

InChIs

1: InChI=1/C8H16O2/c1-6(9)4-5-8(3)7(2)10-8/h6-7,9H,4-5H2,1-3H3/t6-,7?,8+/m0/s1
InChIKey=YEAGKBPXLVGCPK-YPVSKDHRBB

2: InChI=1/C8H16O2/c1-6-4-5-8(3,10-6)7(2)9/h6-7,9H,4-5H2,1-3H3/t6-,7-,8-/m0/s1
InChIKey=RRDMOYCGUXNJSL-FXQIFTODBG

3: InChI=1/C8H16O2/c1-6-4-5-8(3,9)7(2)10-6/h6-7,9H,4-5H2,1-3H3/t6-,7-,8+/m0/s1
InChIKey=WWLWIBPPUNCAQU-BIIVOSGPBQ

Petsalakis and Rebek have explored the fluorescence of stilbene inside a couple of different kinds of capsules.¹ trans-Stilbene exhibits weak fluorescence in solution, but when placed inside a small capsule, the fluorescence disappears almost entirely, while in a large capsule, the fluorescence returns to normal. They examined stilbene inside two different capsules using a variety of DFT and ONIOM techniques.

The optimized geometries of trans- and cis-stilbene optimized at CAM-B3LYP/6-31G(d,p) are displayed in Figure 1. As expected, the trans conformer is planar and the cis conformer is twisted to avoid clashes between the phenyl rings. The optimized structure of the 1.1 capsule is also shown in Figure 1. All of these structures are fairly insensitive to computational method. (They have also looked at an even larger capsule, but I have omitted displaying its structure here.)

(a)	(b)
(c)	(d)

Figure 1. CAM-B3LYP/6-31G(d,p) optimized structures of (a) trans-stilbene, (b) cis-stilbene , (c) the 1.1 capsule, and (d) trans-stilbene inside the 1.1 capsule.

The structure of trans- stilbene inside the 1.1 capsule is shown in Figure 1. Of particular note is that the stilbene is no longer planar. (This twisting is perhaps better observed by an end-on view, which the reader can obtain by clicking on the picture and then manipulating the full 3-D structure using the Jmol applet.) The different computational methods give slightly different encapsulated structures, and vary a bit in their binding energies, but the twisting of the stilbene is reproduced by each method. Though not shown here, trans-stilbene in the larger capsule is again a nearly planar structure.

The structure of the S₁ state of trans-stilbene in the large capsule is the same as for free trans-stilbene. However, the geometry of the S₁ state in the smaller 1.1 capsule is twisted and corresponds to the conical intersection geometry.

The absorption spectra and the emission spectra were computed for the free and encapsulated structures. The absorption and emission spectra for free stilbene and stilbene in the larger capsule are nearly identical, corresponding to the experimental observation of similar fluorescence behavior. The absorption spectra of stilbene in the 1.1 capsule has a small blue shift of 8 nm due to the twisted geometry. But the major result is that the S₁ state of stilbene inside the 1.1 capsule distorts to the conical intersection, allowing for radiationless return to the ground state. This means that there would be no fluorescence, and that is exactly what is observed.

References

(1) Tzeli, D.; Theodorakopoulos, G.; Petsalakis, I. D.; Ajami, D.; Rebek, J., "Conformations and Fluorescence of Encapsulated Stilbene," J. Am. Chem. Soc., 2012, 134, 4346-4354, DOI: 10.1021/ja211164b

InChIs

trans-stilbene: InChI=1/C14H12/c1-3-7-13(8-4-1)11-12-14-9-5-2-6-10-14/h1-12H/b12-11+
InChIKey=PJANXHGTPQOBST-VAWYXSNFBV

cis-stilbene: InChI=1/C14H12/c1-3-7-13(8-4-1)11-12-14-9-5-2-6-10-14/h1-12H/b12-11-
InChIKey=PJANXHGTPQOBST-QXMHVHEDBW

Inspired by a blog post of Henry Rzepa (see here) Shaik and co-workers examined the C₂ species with an eye towards the nature of the bond between the two carbon atoms.¹ Using both a valence bond approach and a full CI approach, they end up at the same place: there is a quadruple bond here!

The argument rests largely on a definition of of an in situ bond energy. For the VB approach, this requires choosing as a reference a non-bonding interaction between the atoms with regards to a pair of electrons. For the CI approach, the bond energy is half the energy of the singlet-triplet gap. So, for C₂, the VB/6-31G* estimate of the bond energy of the putative fourth bond is 14.3 kcal mol^-1. For the full CI/6-31G* computations of the singlet-triplet gap, the bond energy estimate is 14.8 kcal mol^-1, and using the experimental value of the gap, the estimate is 13.2 kcal mol^-1. Not a strong bond, but certainly meaningful!

In the VB approach, the fourth bond is a weighted sum of the antibonding 2σ_u and bonding 3σ_g orbitals – a combination that gives rise to small constructive overlap between the two C atoms. In the CI model, the wavefunction is dominated by the first two configurations; the first configuration, with a coefficient of C₀=0.828 has 2σ_u doubly occupied and the second coefficient, with C_D=0.324, has the 3σ_g orbital doubly occupied. Considering that 3σ_g is a bonding orbital, the significant contribution of this configuration gives rise to the fourth bond.

References

(1) Shaik, S.; Danovich, D.; Wu, W.; Su, P.; Rzepa, H. S.; Hiberty, P. C., "Quadruple bonding in C2 and analogous eight-valence electron species," Nat. Chem., 2012, 4, 195-200, DOI: 10.1038/nchem.1263.

InChIs

C₂: InChI=1/C2/c1-2
InChIKey=LBVWYGNGGJURHQ-UHFFFAOYAE