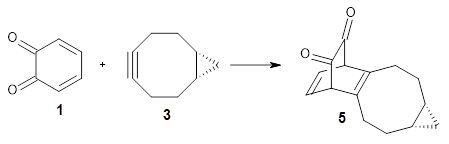
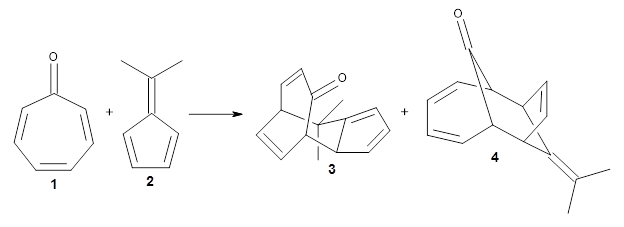
Pericyclic reactions remain a fruitful area of research despite the seminal publication of the Woodward-Hoffmann rules decades ago. Here are two related papers of pericyclic reactions that violate the Woodward-Hoffmann rules.

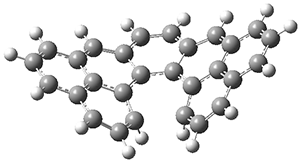
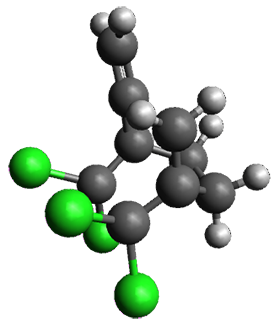
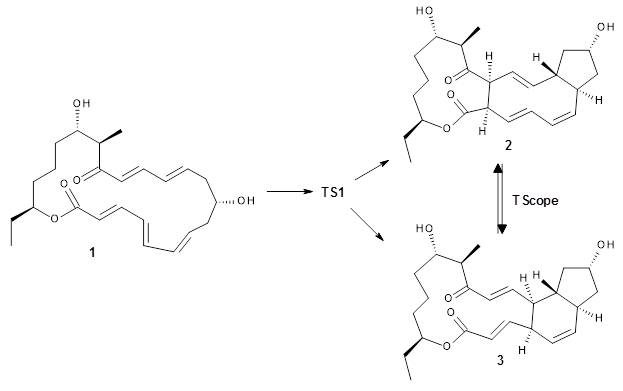
First, Solomek, Ravat, Mou, Kertesz, and Jurícek reported on the thermal and photochemical electrocyclization reaction of diphenylcetherene 1a.¹ Though they were not able to directly detect the intermediate 2, through careful examination of the photochemical reaction, they were able to infer that the thermal cyclization goes via the formally forbidden conrotatory pathway (see Scheme 1).

Scheme 2.

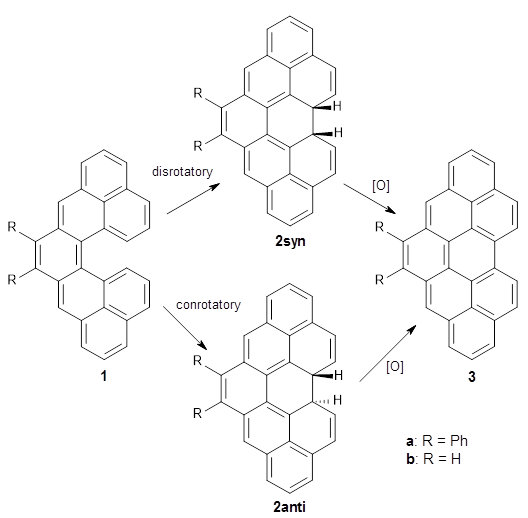
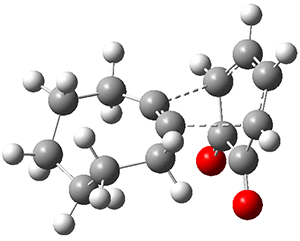
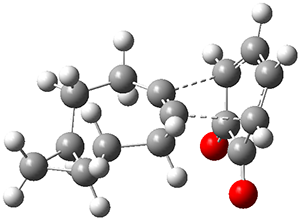
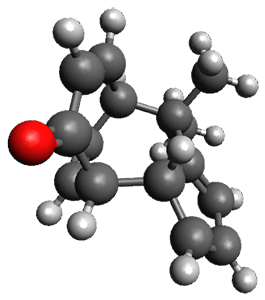
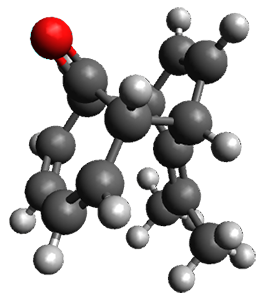
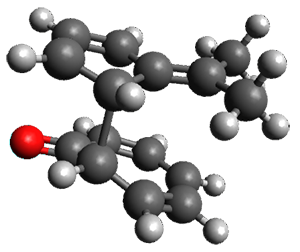
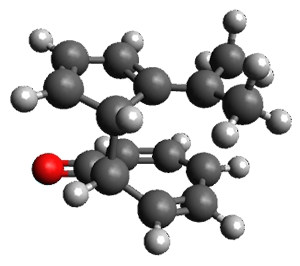
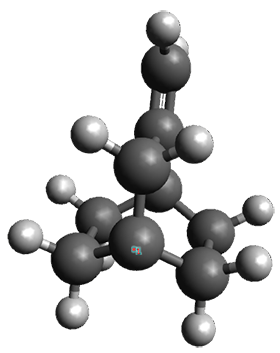
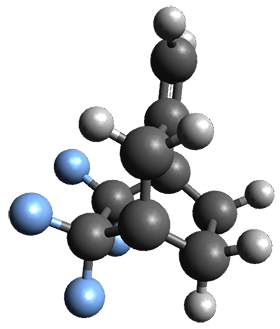
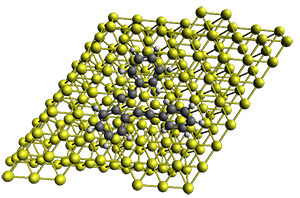
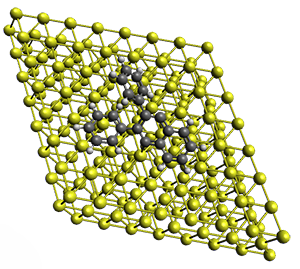
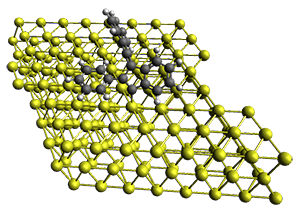
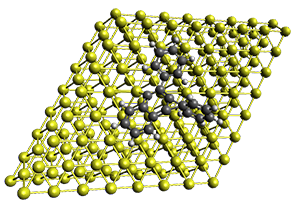
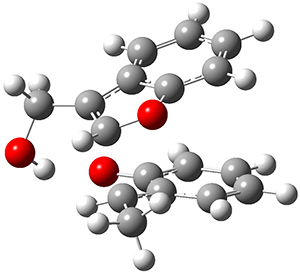
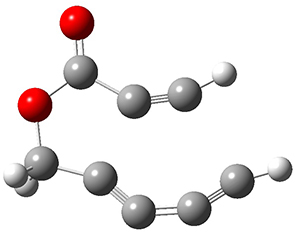
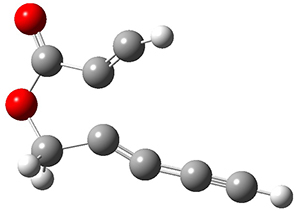
Kinetic studies estimate the activation barrier is 14.1 kcal mol^-1. They performed DFT computations of the parent 1b using a variety of functionals with both restricted and unrestricted wavefunctions. The allowed pathway to 2syn is predicted to be greater than 27 kcal mol^-1, while the formally forbidden pathway to 2anti is estimated to have a lower barrier of about 23 kcal mol^-1. The two transition states for these different pathways are shown in Figure 1, and the sterics that force a helical structure to 1 help make the forbidden pathway more favorable.

Figure 1. (U)B3LYP/6-31G optimized geometries of the transition states taking 1 into 2.

Nonetheless, all of the DFT computations significantly overestimate the activation barrier. The authors make the case that a low-lying singlet excited state results in an early conical intersection that reduces the symmetry from C₂ to C₁. In this lower symmetry pathway, all of the states can mix, leading to a lower barrier. However, since DFT is intrinsically a single Slater configuration, the mixing of the other states is not accounted for, leading to the overestimated barrier height.

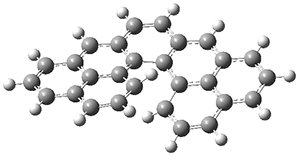
In a follow up study, this group examined the thermal and photo cyclization of 13,14-dimethylcethrene 4.² The added methyl groups make the centhrene backbone more helical, and this precludes the formal allowed disrotatory process. The methyl groups also prohibit the oxidation that occurs with 1, driven by aromatization, allowing for the isolation of the direct product of the cyclization 5. This anti stereochemistry is confirmed by NMR and x-ray crystallography. The interconversion between 4 and 5 can be controlled by heat and light, making the system an interesting photoswitch.

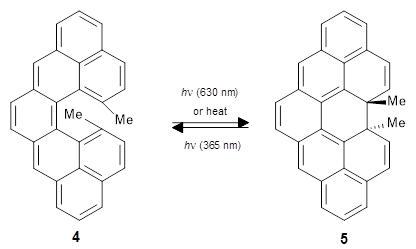
Also of interest is the singlet-triplet gap of 1 and 4. The DFT computed ΔE_ST is about 10 kcal mol^-1 for 4, larger than the computed value of 6 kcal mol^-1 for 1b. The EPR of 1b does show a signal while that of 4 has no signal. To assess the role of the methyl group, they computed the singlet triplet gaps for 1b and 4 at two different geometries: where the distance between the carbons bearing the methyl groups is that in 1b (3.03 Å) and in 4 (3.37 Å). The lengthening of this distance by the methyl substituents is due to increased helical twist in 4 than in 1b. For 1b, the gap increases with twisting, from 7.1 to 8.3 kcal mol^-1, while for 4 the gap increases by 1.8 kcal mol^-1 with the increased twisting. This change is less than the effect of methyl substitution, which increases the gap by 2.2 kcal mol^-1 at the shorter distance and 2.8 kcal mol^-1 at the longer distance. Thus, the electronic (orbital) effect of methyl substitution affects the singlet-triplet gap more than the geometric twisting.

References

1) Šolomek, T.; Ravat, P.; Mou, Z.; Kertesz, M.; Juríček, M., "Cethrene: The Chameleon of Woodward–Hoffmann Rules." J. Org. Chem. 2018, 83, 4769-4774, DOI: 10.1021/acs.joc.8b00656.

2) Ravat, P.; Šolomek, T.; Häussinger, D.; Blacque, O.; Juríček, M., "Dimethylcethrene: A Chiroptical Diradicaloid Photoswitch." J. Am. Chem. Soc. 2018, 140, 10839-10847, DOI: 10.1021/jacs.8b05465.

InChIs

1b: InChI=1S/C28H16/c1-5-17-7-3-11-23-25(17)19(9-1)15-21-13-14-22-16-20-10-2-6-18-8-4-12-24(26(18)20)28(22)27(21)23/h1-16H
InChIKey=GBMHAGKZRAVBDO-UHFFFAOYSA-N

4: InChI=1S/C30H20/c1-17-9-11-19-5-3-7-21-15-23-13-14-24-16-22-8-4-6-20-12-10-18(2)26(28(20)22)30(24)29(23)25(17)27(19)21/h3-16H,1-2H3
InChIKey=MXTVFWTUCPRNIW-UHFFFAOYSA-N

5: nChI=1S/C30H20/c1-29-13-11-17-5-3-7-19-15-21-9-10-22-16-20-8-4-6-18-12-14-30(29,2)28(24(18)20)26(22)25(21)27(29)23(17)19/h3-16H,1-2H3/t29-,30-/m0/s1
InChIKey=SUMMGEBJORQMAI-KYJUHHDHSA-N

I recently posted on a paper proposing 1,2-benzoquinone and related compounds as the diene component for bioorthogonal labeling. Levandowski, Gamache, Murphy, and Houk report on tetrachlorocyclopentadiene ketal 1 as an active ambiphilic diene component.¹

1 is sterically congested to diminish self-dimerization and will react with both electron-rich and electron-poor dienes. To test it as an active diene in bioorthogonal labeling applications, they optimized the structures of the transition states at CPCM(water)/M06-2X/6-311+G(d,p)//CPCM(water)/M06-2X/6-31G(d) for the Diels-Alder reaction of 1 with a variety of dienophiles including trans-cyclooctene 2 and endo-bicyclononyne 3. These transition states are shown in Figure 1. The activation free energy is quite low for each: 18.1 kcal mol^-1 with 2 and 18.9 kcal mol^-1 with 3.

Figure 1. CPCM(water)/M06-2X/6-31G(d) optimized geometries for the TSs of the reaction of 1 with 2 and 3.

Experiments were successfully run using 1 as a label on a neuropeptide.

References

1) Levandowski, B. J.; Gamache, R. F.; Murphy, J. M.; Houk, K. N., "Readily Accessible Ambiphilic Cyclopentadienes for Bioorthogonal Labeling." J. Am. Chem. Soc. 2018, 140, 6426-6431, DOI: 10.1021/jacs.8b02978.

InChIs

1:InChI=1S/C7H4Cl4O2/c8-3-4(9)6(11)7(5(3)10)12-1-2-13-7/h1-2H2
InChIkey=DXQQKKGWMVTLOJ-UHFFFAOYSA-N

At the recent ACS meeting in New Orleans, Ken Houk spoke at the Dreyfus award session in honor of Michele Parrinello. Ken’s talk included discussion of some recent molecular dynamics studies of pericyclic reactions. Because of their similarities in approaches and observations, I will discuss three recent papers from his group (which Ken discussed in New Orleans) in this post.

The Cope rearrangement, a fundamental organic reaction, has been studied extensively by computational means (see Chapter 4.2 of my book). Mackey, Yang, and Houk examine the degenerate Cope rearrangement of 1,5-hexadiene with molecular dynamics at the (U)B3LYP/6-31G(d) level.¹ They examined 230 trajectories, and find that of the 95% of them that are reactive, 94% are trajectories that directly cross through the transition zone. By this, Houk means that the time gap between the breaking and forming C-C bonds is less than 60 fs, the time for one C-C bond vibration. The average time in the transition zone is 35 fs. This can be thought of as “dynamically concerted”. For the other few trajectories, a transient diradical with lifetime of about 100 fs is found.

The dimerization of cyclopentadiene finds the two [4+2] pathways merging into a single bispericylic transition state. ² Only a small minority (13%) of the trajectories sample the region about the Cope rearrangement that interconverts the two mirror image dimers. These trajectories average about 60 fs in this space, which comes from the time separation between the formation of the two new C-C bonds. The majority of the trajectories quickly pass through the dimerization transition zone in about 18 fs, and avoid the Cope TS region entirely. These paths can be thought of as “dynamically concerted”, while the other set of trajectories are “dynamically stepwise”. It should be noted however that the value of S² in the Cope transition zone are zero and so no radicals are being formed.

Finally, Yang, Dong, Yu, Yu, Li, Jamieson, and Houk examined 15 different reactions that involve ambimodal (i.e. bispericyclic) transition states.³ They find a strong correlation between the differences in the bond lengths of the two possible new bond vs. their product distribution. So for example, in the reaction shown in Scheme 1, bond a is the one farthest along to forming. Bond b is slightly shorter than bond c. Which of these two is formed next is dependent on the dynamics, and it turns out the P_ab is formed from 73% of the trajectories while P_ac is formed only 23% of the time. This trend is seen across the 15 reaction, namely the shorter of bond b or c in the transition state leads to the larger product formation. When competing reactions involve bonds with differing elements, then a correlation can be found with bond order instead of with bond length.

Scheme 1

References

1) Mackey, J. L.; Yang, Z.; Houk, K. N., "Dynamically concerted and stepwise trajectories of the Cope rearrangement of 1,5-hexadiene." Chem. Phys. Lett. 2017, 683, 253-257, DOI: 10.1016/j.cplett.2017.03.011.

2) Yang, Z.; Zou, L.; Yu, Y.; Liu, F.; Dong, X.; Houk, K. N., "Molecular dynamics of the two-stage mechanism of cyclopentadiene dimerization: concerted or stepwise?" Chem. Phys. 2018, in press, DOI: 10.1016/j.chemphys.2018.02.020.

3) Yang, Z.; Dong, X.; Yu, Y.; Yu, P.; Li, Y.; Jamieson, C.; Houk, K. N., "Relationships between Product Ratios in Ambimodal Pericyclic Reactions and Bond Lengths in Transition Structures." J. Am. Chem. Soc. 2018, 140, 3061-3067, DOI: 10.1021/jacs.7b13562.

Click chemistry has been used in a broad range of applications. The use of metal catalysts has limited its application to biological system, but the development of strain-promoted cycloaddition to cyclooctyne has opened up click chemistry to bioorthogonal labeling.

An interesting variation on this is the use of 1,2-benzoquinone 1 and substituted analogues as the Diels-Alder diene component. Escorihuela and co-workers have reported on the use of this diene with a number of cyclooctyne derivatives, measuring kinetics and also using computations to assess the mechanism.¹

Their computations focused on two reactions using cyclooctyne 2 and the cyclopropane-fused analogue 3:

They examined these reactions with a variety of density functionals along with some post-HF methods. The transition states of the two reactions are shown in Figure 1. A variety of different density functionals and MP2 are consistent in finding synchronous or nearly synchronous transition states.

Figure 1. B97D/6-311+G(d,p) transition states for Reactions 1 and 2.

In terms of activation energies, all of the DFT methods consistently overestimate the barrier by about 5-10 kcal mol^-1, with B97D-D3 doing the best. MP2 drastically underestimates the barriers, though the SOS-MP2 or SCS-MP2 improve the estimate. Both CCSD(T) and MR-AQCC provide estimates of about 8.5 kcal mol^-1, still 3-4 kcal mol^-1 too high. The agreement between CCSD(T), a single reference method, and MR-AQCC, a multireference method, indicate that the transition states have little multireference character. Given the reasonable estimate of the barrier afforded by B97D-D3, and its tremendous performance advantage over SCS-MP2, CCSD(T) and MR-AQCC, this is the preferred method (at least with current technology) for examining Diels-Alder reactions like these, especially with larger molecules.

References

1) Escorihuela, J.; Das, A.; Looijen, W. J. E.; van Delft, F. L.; Aquino, A. J. A.; Lischka, H.; Zuilhof, H., "Kinetics of the Strain-Promoted Oxidation-Controlled Cycloalkyne-1,2-quinone Cycloaddition: Experimental and Theoretical Studies." J. Org. Chem. 2018, 83, 244-252, DOI: 10.1021/acs.joc.7b02614.

InChIs

1: InChI=1S/C6H4O2/c7-5-3-1-2-4-6(5)8/h1-4H
InChIKey=WOAHJDHKFWSLKE-UHFFFAOYSA-N

2: InChI=1S/C8H12/c1-2-4-6-8-7-5-3-1/h1-6H2
InChIKey=ZPWOOKQUDFIEIX-UHFFFAOYSA-N

3: InChI=1S/C9H12/c1-2-4-6-9-7-8(9)5-3-1/h8-9H,3-7H2
InChIKey=rQDNSAFCVPAMWCJ-UHFFFAOYSA-N

4: InChI=1S/C14H16O2/c15-13-11-7-8-12(14(13)16)10-6-4-2-1-3-5-9(10)11/h7-8,11-12H,1-6H2
InChIKey=OQMYZEFKUMPECV-UHFFFAOYSA-N

5: InChI=1S/C15H16O2/c16-14-12-5-6-13(15(14)17)11-4-2-9-7-8(9)1-3-10(11)12/h5-6,8-9,12-13H,1-4,7H2/t8-,9+,12?,13?
InChIKey=NKDGTIVNLDJQKR-RFZWMSCOSA-N

Bispericyclic transition states arise when two pericyclic reactions merge to a common transition state. This leads to a potential energy surface with a bifurcation such that reactions that traverse this type of transition state will head towards two different products. The classic example is the dimerization of cyclopentadiene, involving two [4+2] Diels-Alder reactions. Unusual PESs are discussed in my book and in past blog posts.

Houk and coworkers have now identified a bispericyclic transition state involving two [6+4] cycloadditions.¹ Reaching back to work Houk pursued as a graduate student with Woodward for inspiration, these authors examined the reaction of tropone 1 with dimethylfulvene 2. Each moiety can act as the diene or triene component of a [6+4] allowed cycloaddition:

The product with fulvene 2 as the 6 π-e component and tropone as the 4 π-e component [6_F+4_T] is 3, while reversing their participation in the [6_T+4_F] cycloaddition leads to 4. A variety of [4+2] reactions are also possible. All of these reactions were investigated at PCM/M06-2X/6-311+G(d,p)//B3LYP-D3/6-31G(d). The reaction leading to 3 is exothermic by 3.0 kcal mol^-1, while the reaction to 4 endothermic by 1.3 kcal mol^-1.

Interestingly, there is only one transition state that leads to both 3 and 4, the first known bispericyclic transition state for two conjoined [6+4] cycloadditions. The barrier is 27.9 kcal mol^-1. The structures of the two products and the transition state leading to them are shown in Figure 1. 3 and 4 can interconvert through a Cope transition state, also shown in Figure 1, with a barrier of 26.3 kcal mol^-1 (for 4 → 3).

3	4
TS [6+4]	TS Cope

Figure 1. B3LYP-D3/6-31G(d) optimized geometries.

Given that a single transition leads to two products, the product distribution is dependent on the molecular dynamics. A molecular dynamics simulation at B3LYP-D3/6-31G(d) with 117 trajectories indicates that 4 is formed 91% while 3 is formed only 9%. Once again, we are faced with the reality of much more complex reaction mechanisms/processes than simple models would suggest.

References

1) Yu, P.; Chen, T. Q.; Yang, Z.; He, C. Q.; Patel, A.; Lam, Y.-h.; Liu, C.-Y.; Houk, K. N., "Mechanisms and Origins of Periselectivity of the Ambimodal [6 + 4] Cycloadditions of Tropone to Dimethylfulvene." J. Am. Chem. Soc. 2017, 139 (24), 8251-8258, DOI: 10.1021/jacs.7b02966.

InChIs

1: InChI=1S/C7H6O/c8-7-5-3-1-2-4-6-7/h1-6H
InChIKey=QVWDCTQRORVHHT-UHFFFAOYSA-N

2: InChI=1S/C8H10/c1-7(2)8-5-3-4-6-8/h3-6H,1-2H3
InChIKey=WXACXMWYHXOSIX-UHFFFAOYSA-N

3:InChI=1S/C15H16O/c1-15(2)10-6-8-12(14(16)9-7-10)11-4-3-5-13(11)15/h3-12H,1-2H3
InChIKey=SEKRUGIZAIQCDA-UHFFFAOYSA-N

4: InChI=1S/C15H16O/c1-9(2)14-10-7-8-11(14)13-6-4-3-5-12(10)15(13)16/h3-8,10-13H,1-2H3
InChIKey=AQQAMUGJSGJKLC-UHFFFAOYSA-N

Bispericyclic reactions occur when two different pericyclic reactions merge to have a single transition state. An example of this is the joining of two [3,3]-sigmatopic rearrangements of 1 that merge to have a single transition state. Lopez, Faza, and Lopez have examined the dynamics of this reaction.¹

Because of the symmetry of the species along this reaction pathway, the products of the two different rearrangements are identical, and will be formed in equal amounts, though they are produced from a single transition state with the reaction pathway bifurcating due to a valley-ridge inflection post TS.

The interesting twist that is explored here is when 1 is substituted in order to break the symmetry. The authors have examined 3x with either fluorine, chlorine, or bromine. The critical points on the reactions surface were optimized at M06-2X/Def2TZVPP. In all three cases a single bispericyclic transition state 3TS1x is found, which leads to products 4a and 4b. A second transition state 4TSx corresponds to the [3,3]-rearrangement that interconverts the two products. The structures of 1TS, 3TS1F, and 3TS1Cl are shown in Figure 1.

1TS

3TS1F

3TS1Cl

Figure 1. M06-2X/Def2TZVPP optimized geometries of 1TS, 3TS1F, and 3TS1Cl.

The halogen substitution breaks the symmetry of the reaction path. This leads to a number of important changes. First, the C₄-C₅ and C₇-C₈ distances, which are identical in 1TS, are different in the halogen cases. Interestingly, the distortions are dependent on the halogen: in 3TS1F C₄-C₅ is 0.2 Å longer than C₇-C₈, but in 3TS1Cl C₇-C₈ is much longer (by 0.65 Å) than C₄-C₅. Second, the products are no longer equivalent with the halogen substitution. Again, this is halogen dependent: 4bF is 4.0 kcal mol^-1 lower in energy than 4aF, while 4aCl is 8.2 kcal mol^-1 lower than 4bCl.

These difference manifest in very different reaction dynamics. With trajectories initiated at the first (bispericyclic) transiting state, 89% end at 4bF and 9% end at 4aF, a ratio far from unity that might be expected from both products resulting from passage through the same TS. The situation is even more extreme for the chlorine case, where all 200 trajectories end in 4aCl. This is yet another example of the role that dynamics play in reaction outcomes (see these many previous posts).

References

1) Villar López, R.; Faza, O. N.; Silva López, C., "Dynamic Effects Responsible for High Selectivity in a [3,3] Sigmatropic Rearrangement Featuring a Bispericyclic Transition State." J. Org. Chem. 2017, 82 (9), 4758-4765, DOI: 10.1021/acs.joc.7b00425.

InChIs

1: InChI=1S/C9H12/c1-3-9-6-4-8(2)5-7-9/h1-2,4-7H2
InChIKey=RRXCPJIEZVQPSZ-UHFFFAOYSA-N

2: InChIInChIKey=AMBNQWVPTPHADI-UHFFFAOYSA-N

3F: InChI=1S/C9H8F4/c1-3-7-5-4-6(2)8(10,11)9(7,12)13/h1-2,4-5H2
InChIKey=VZFAQFJKHDWJDN-UHFFFAOYSA-N

3Cl: InChI=1S/C9H8Cl4/c1-3-7-5-4-6(2)8(10,11)9(7,12)13/h1-2,4-5H2
InChIKey=AIVUHFMHIMNOJB-UHFFFAOYSA-N

4aF: InChI=1S/C9H8F4/c1-5-4-6(8(10)11)2-3-7(5)9(12)13/h1-4H2
InChIKey=NAUUHIHYMAOMIF-UHFFFAOYSA-N

4aCl: InChI=1S/C9H8Cl4/c1-5-4-6(8(10)11)2-3-7(5)9(12)13/h1-4H2
InChIKey=MMCKDJXQYSGQEH-UHFFFAOYSA-N

4bF: InChI=1S/C9H8F4/c1-5-4-6(2)8(10,11)9(12,13)7(5)3/h1-4H2
InChIKey=LMFNAIRCNARWSX-UHFFFAOYSA-N

4bCl: InChI=1S/C9H8Cl4/c1-5-4-6(2)8(10,11)9(12,13)7(5)3/h1-4H2
InChIKey=NOFFASDSCUGRTP-UHFFFAOYSA-N

The Bergman cyclization and some competitive reactions are discussed in detail in Chapter 4 of by book. The Bergman cyclization makes the C₁-C₆ bond from an enediyne. Another, but rarer, option is to make the C₁-C₅ bond, the Schreiner-Pascal cyclization pathway. de Oteyza and coworkers have examined the competition between these two pathways for 1 on a gold surface, and used STM and computations to identify the reaction pathway.¹

The two pathways are shown below. The STM images identify 1 as the reactant on the gold surface and the product is 6. No other product is observed.

Projector augmented wave (PAW) pseudo-potential computations using the PBE functional were performed for the reaction on a Au (111) surface was modeled by a 7 x 7 x 3 supercell. The optimized geometries of the critical points are show in Figure 1.

1
TS(1→2)	TS(1→3)
2	3
TS(2→6)	TS(3→5)
6	5

Figure 1. Optimized geometries of the critical points on the two reaction pathways.

Explicit values of the relative energies are not given in either the paper or the supporting information, but rather a plot shows the relative positions of the critical points. The important points are the following: (a) the barrier for the C₁-C₅ cyclization is lower than the barrier for the C₁-C₆ cyclization and 3 is lower in energy than 2; (b) 5 is lower in energy than 6; and (c) the barrier for taking 2 to 6 is significantly below the barrier taking 3 into 5. The barrier for the phenyl migration taking 3 into 5 is so high because of a strong interaction between the carbon radical and a gold atom of the surface. The authors suggest that the two initial cyclizations are reversible, but the very high barrier for forming 5 precludes it from taking place, leaving only the route to 6 as a viable pathway.

References

(1) de Oteyza, D. G.; Paz, A. P.; Chen, Y.-C.; Pedramrazi, Z.; Riss, A.; Wickenburg, S.; Tsai, H.-Z.; Fischer, F. R.; Crommei, M. F.; Rubio, A. “Enediyne Cyclization on Au(111),” J. Amer. Chem. Soc. 2016, 138, 10963–10967, DOI: 10.1021/jacs.6b05203.

InChIs

1: InChI=1S/C22H14/c1-3-9-19(10-4-1)15-17-21-13-7-8-14-22(21)18-16-20-11-5-2-6-12-20/h1-14H
InChIKey=XOJSMLDMLXWRMT-UHFFFAOYSA-N

2: InChI=1S/C22H14/c1-3-9-17(10-4-1)21-15-19-13-7-8-14-20(19)16-22(21)18-11-5-2-6-12-18/h1-14H
InChIKey=DAUFPUDTOKPCMX-UHFFFAOYSA-N

3: InChI=1S/C22H14/c1-3-9-17(10-4-1)15-22-20-14-8-7-13-19(20)16-21(22)18-11-5-2-6-12-18/h1-14H
InChiKey=>FYBPBPGPMCJQNF-UHFFFAOYSA-N

4: InChI=1S/C22H14/c1-3-9-17(10-4-1)20-15-19-13-7-8-14-21(19)22(16-20)18-11-5-2-6-12-18/h1-14H
InChIKey=CYXVOOSYXXUHFV-UHFFFAOYSA-N

5: InChI=1S/C22H14/c1-3-9-17(10-4-1)15-19-16-22(18-11-5-2-6-12-18)21-14-8-7-13-20(19)21/h1-14H
InChIKey=BIKDAEZYYCKGSI-UHFFFAOYSA-N

6: InChI=1S/C22H14/c1-3-9-15(10-4-1)19-17-13-7-8-14-18(17)21-20(22(19)21)16-11-5-2-6-12-16/h1-14H
InChIKey=GAXPSSOZJDJRPN-UHFFFAOYSA-N

Enzyme SpnF is implicated in catalyzing the putative [4+2] cycloaddition taking 1 into 3. Houk, Singleton and co-workers have now examined the mechanism of this transformation in aqueous solution but without the enzyme.¹ As might be expected, this mechanism is not straightforward.

Reactant 1, transition states, and products 2 and 3 were optimized at SMD(H2O)/M06-2X/def2-TZVPP//B3LYP-D3(BJ)//6-31+G(d,p). Geometries and relative energies are shown in Figure 1. The reaction 1 → 2 is a formal [6+4] cycloaddition, and the reaction 1 → 3 is a formal [4+2] cycloaddition. Interestingly, only a single transition state could be located TS1. It is a bispericyclic TS (see Chapter 4 of my book), where these two pericyclic reaction sort of merge together. After TS1 is traversed the potential energy surface bifurcates, leading to 2 or 3. This is yet again an example of a single TS leading to two different products. (See the many posts I have written on this topic.) The barrier height is 27.6 kcal mol^-1, with 2 lying 13.1 kcal mol^-1 above 3. However, the steepest descent pathway from TS1 leads to 2. There is a second transition state TScope that describes a Cope rearrangement between 2 and 3. Using the more traditional TS theory description, 1 undergoes a [6+4] cycloaddition to form 2 which then crosses a lower barrier (TScope) to form the thermodynamically favored 3, which is the product observed in the enzymatically catalyzed reaction.

1 (0.0)
TS1 (27.6)
2 (4.0)	3 (-9.1)
(24.7)

Figure 1. B3LYP-D3(BJ)//6-31+G(d,p) optimized geometries and relative energies in kcal mol^-1.

Molecular dynamics computations were performed on this system by tracking trajectories starting in the neighborhood of TS1 on a B3LYP-D2/6-31G(d) PES. The results are that 63% of the trajectories end at 2, 25% end at 3, and 12% recross back to reactant 1, suggesting an initial formation ratio for 2:3 of 2.5:1. The reactions are very slow to cross through the “transition zone”, typically 2-3 times longer than for a usual Diels-Alder reaction (see this post).

Once again, we see an example of dynamic effects dictating a reaction mechanism. The authors pose a tantalizing question: Can an enzyme control the outcome of an ambimodal reaction by altering the energy surface such that the steepest downhill path from the transition state leads to the “desired” product(s)? The answer to this question awaits further study.

References

(1) Patel, A; Chen, Z. Yang, Z; Gutierrez, O.; Liu, H.-W.; Houk, K. N.; Singleton, D. A. “Dynamically
Complex [6+4] and [4+2] Cycloadditions in the Biosynthesis of Spinosyn A,” J. Amer. Chem. Soc. 2016, 138, 3631-3634, DOI: 10.1021/jacs.6b00017.

InChIs

1: InChI=1S/C24H34O5/c1-3-21-15-12-17-23(27)19(2)22(26)16-10-7-9-14-20(25)13-8-5-4-6-11-18-24(28)29-21/h4-11,16,18-21,23,25,27H,3,12-15,17H2,1-2H3/b6-4+,8-5+,9-7+,16-10+,18-11+/t19-,20+,21-,23-/m0/s1
InChIKey=JEKALMRMHDPSQK-ZTRRSECRSA-N

2: InChI=1S/C24H34O5/c1-3-19-8-6-10-22(26)15(2)23(27)20-12-11-17-14-18(25)13-16(17)7-4-5-9-21(20)24(28)29-19/h4-5,7,9,11-12,15-22,25-26H,3,6,8,10,13-14H2,1-2H3/b7-4-,9-5+,12-11+/t15-,16-,17-,18-,19+,20+,21-,22+/m1/s1
InChIKey=AVLPWIGYFVTVTB-PTACFXJJSA-N

3: InChI=1S/C24H34O5/c1-3-19-5-4-6-22(26)15(2)23(27)11-10-20-16(9-12-24(28)29-19)7-8-17-13-18(25)14-21(17)20/h7-12,15-22,25-26H,3-6,13-14H2,1-2H3/b11-10+,12-9+/t15-,16+,17-,18-,19+,20-,21-,22+/m1/s1
InChIKey=BINMOURRBYQUKD-MBPIVLONSA-N

Xu, Liu, Xu, Gao, and Zhao report a very efficient synthesis of paeoveitol 1 by the [4+2]-cycloaddition of paeveitol D 2 with the o-quinone methide 3.¹ What is interesting here is the selectivity of this reaction. In principle the cyloadditon can give four products (2 different regioisomeric additions along with endo/exo selectivity) and it could also proceed via a Michael addition.

They performed PCM(CH₂Cl₂)/M06-2x/6-311+G(d,p) computations on the reaction of 2 with 3 and located two different transition states for the Michael addition and the four cycloaddition transition states. The lowest energy Michael and cycloaddition transition states are shown in Figure 1. The barrier for the cycloaddition is 17.6 kcal mol^-1, 2.5 kcal mol^-1 below that of the Michael addition. The barriers for the other cycloaddition paths are at more than 10 kcal mol^-1 above the one shown. This cycloaddition TS is favored by a strong intermolecular hydrogen bond and by π-π-stacking. In agreement with experiment, it is the transition state that leads to the observed product.

Michael TS (20.1)

[4+2] TS (17.6)

Figure 1. Optimized geometries of the lowest energy TSs for the Michael and [4+2]cycloaddtion routes. Barrier heights (kcal mol^-1) are listed in parenthesis.

References

(1) Xu, L.; Liu, F.; Xu, L.-W.; Gao, Z.; Zhao, Y.-M. "A Total Synthesis of Paeoveitol," Org. Lett. 2016, ASAP, DOI: 10.1021/acs.orglett.6b01736.

paeoveitol 1: InChI=1S/C21H24O3/c1-5-21-10-14-6-11(2)17(22)8-15(14)13(4)20(21)24-19-7-12(3)18(23)9-16(19)21/h6-9,13,20,22-23H,5,10H2,1-4H3/t13-,20-,21-/m1/s1
InChIKey=LCLFTLPUJXVULB-OBVPDXSSSA-N

paeveitol D 2: InChI=1S/C9H10O2/c1-3-7-5-8(10)6(2)4-9(7)11/h3-5,10H,1-2H3/b7-3+
InChIKey=KWDDAFOCZGDLEG-XVNBXDOJSA-N

3: InChI=1S/C9H10O2/c1-3-7-5-8(10)6(2)4-9(7)11/h3-5,10H,1-2H3/b7-3+
InChIKey=KWDDAFOCZGDLEG-XVNBXDOJSA-N

I have been delinquent in writing about the dehydro-Diels-Alder reactions, but really can’t put it off any further. These sets of reactions really deserve a fuller analysis than I am going to summarize here, but this post will provide a good jumping off point for anyone interested in further investigation.

So the Diels-Alder reaction is among the most famous and most important reactions in organic chemistry. The reaction creates a 6-member ring and sets up to four stereocenters. In the past couple of years many chemists have expressed interest in the variant where the four-carbon component is more highly unsaturated, i.e. enyne or diyne. I will summarize the results of three recent computational papers dealing with the reaction of a diyne with an yne.

The first paper is by Skraba-Joiner, Johnson, and Agarwal.¹ They discuss, among a number of interesting pericyclic reactions, the intramolecular Diels-Alder reaction of triyne 1 to give 2. They examined a concerted and stepwise pathway at (U)M05-2X/6-311+G(d,p) and find the concerted to be favored by 6.0 kcal mol^-1. CCSD(T) using these geometries increases the difference to 8.2 kcal mol^-1. The T₁ diagnostic is fairly large for both the concerted and stepwise transition states, so they also performed CCSD(T)/CBS computations, which had much lower T₁ values. The concerted TS remained favorable, but by only 2.7 kcal mol^-1.

In the same special issue of the Journal of Organic Chemistry, Cramer, Hoye, and Kuwata examined a reaction closely related to what Johnson examined above.² They looked at the reaction taking 3 into 4 via both experiments and computations. The M06-2x/6-311+G(d,p) geometries for the concerted and first TS along the stepwise path (with R1=R2=H) are shown in Figure 1. Evaluating the energies at SMD(o-dichlorobenzene)/B3LYP-D3BJ/6-311+G-(d,p)//M06-2X/6-311+G(d,p) find in this case (along with all of the other R1/R2 variants they examined) that the stepwise path has a lower barrier than the concerted path. In the case where R1=R2=H, the stepwise path is favored by 6.0 kcal mol^-1. Additionally, these stepwise barriers are in reasonable agreement with the experimentally-derived barriers.

Concerted TS

Stepwise TS

Figure 1. M06-2x/6-311+G(d,p) optimized geometries of the concerted and stepwise TSs for the reaction of 3H going to 4H.

It should be pointed out that the wavefunctions for the concerted TSs were all found to be unstable with regard to a restricted to unrestricted relaxation. Given this problem, they also performed a CASPT2 energy evaluation of the concerted and stepwise transition states for the case R1=R2=H. CASPT2 finds the stepwise barrier to be 3.7 kcal mol^-1 lower than the concerted barrier.

The last paper comes from the Houk lab, and examines the simplest set of intermolecular dehdro-Diels-Alder reactions.³ I will focus here on the most unsaturated analogue, the reaction of 1,3-butadiyne 5 with ethyne to give benzyne 6.

The concreted and stepwise transition states for this reaction (at (U)M06-2X/6-311+G(d,p)) are shown in Figure 2. The concerted barrier is 36.0 kcal moml^-1 while the stepwise barrier is slightly lower: 35.2 kcal mol^-1. The distortion energy for the concerted reaction is large (43.2 kcal mol^-1) due mostly to angle changes in the diyne. Its interaction energy is -7.2 kcal mol^-1, similar to the interaction energy in other similar Diels-Alder reactions. In contrast, the distortion energy for the stepwise pathway is 27.5 kcal mol^-1, but the interaction energy is +7.7 kcal mol^-1. These values are very similar to the distortion and interaction energy of the related (but less saturated DA reactions).

Concerted TS

Stepwise TS

Figure 2. (U)M06-2X/6-311+G(d,p) optimized concerted and stepwise TS for the reaction of 1,3-diyne with ethyne.

Molecular dynamics trajectories for both the concerted and stepwise paths reveal interesting differences. The concerted trajectories show an oscillatory behaviour of bending the angles at the C2 and C3 carbons prior to the TS, and then near synchronous formation of the new C-C bonds. The trajectories initiated at the stepwise TS show no systematic motion. Once the bond is formed, the biradical exhibits a long lifetime, on the order of picoseconds, much longer than the trajectory runs.

These three studies indicate the nature of the dehydro Diels-Alder reaction is very sensitive to reaction conditions, substituents, solvation, and all other manner of effects and will likely prove an area of interest for some time. It should keep a number of computational chemists busy for some time!

References

(1) Skraba-Joiner, S. L.; Johnson, R. P.; Agarwal, J. "Dehydropericyclic Reactions: Symmetry-Controlled Routes to Strained Reactive Intermediates," J. Org. Chem. 2015, 80, 11779-11787, DOI: 10.1021/acs.joc.5b01488.

(2) Marell, D. J.; Furan, L. R.; Woods, B. P.; Lei, X.; Bendelsmith, A. J.; Cramer, C. J.; Hoye, T. R.; Kuwata, K. T. "Mechanism of the Intramolecular Hexadehydro-Diels–Alder Reaction," J. Org. Chem. 2015, 80, 11744-11754, DOI: 10.1021/acs.joc.5b01356.

(3) Yu, P.; Yang, Z.; Liang, Y.; Hong, X.; Li, Y.; Houk, K. N. "Distortion-Controlled Reactivity and Molecular Dynamics of Dehydro-Diels–Alder Reactions," J. Am. Chem. Soc. 2016, 138, 8247-8252, DOI: 10.1021/jacs.6b04113.

InChIs

1: InChI=1S/C9H8/c1-3-5-7-9-8-6-4-2/h1-2H,5,7,9H2
InChIKey=IYZAZSVBWMMSLQ-UHFFFAOYSA-N

2: InChI=1S/C9H8/c1-2-5-9-7-3-6-8(9)4-1/h1,4H,3,6-7H2
InChIKey=PZJMTUKDGZUDBH-UHFFFAOYSA-N

3H: InChI=1S/C8H4O2/c1-3-5-6-7-10-8(9)4-2/h1-2H,7H2
InChIKey=MGXDIFXPYGGQLF-UHFFFAOYSA-N

4H: InChI=1S/C10H8O4/c1-6(11)14-8-2-3-9-7(4-8)5-13-10(9)12/h2-4H,5H2,1H3
InChIKey=GEFLHLNIKGXWCA-UHFFFAOYSA-N

5: InChI=1S/C4H2/c1-3-4-2/h1-2H
InChIKey=LLCSWKVOHICRDD-UHFFFAOYSA-N

6: InChI=1S/C6H4/c1-2-4-6-5-3-1/h1-4H
InChIKey=KLYCPFXDDDMZNQ-UHFFFAOYSA-N