I know this post is off topic for the blog, but yesterday’s New York Times simply raised my blood pressure.

The Book Review of the New York Times on Sunday March 30 has a review on the book Bonk. The book discusses sex research – and while that certainly is of interest – I want to focus on the associated artwork.

Now chemistry is not usually considered a particularly sexy subject, but its graphics can be fascinating. The periodic table might be the most widely known scientific graphic. The structure of DNA has captured the imagination of more than just scientists. And so it’s not unreasonable that the Times Book Review editors would choose to present some chemical (2-D) drawings. Given the subject of the book, one might have expected perhaps the structures of testosterone and progesterone. Instead, we get the following:

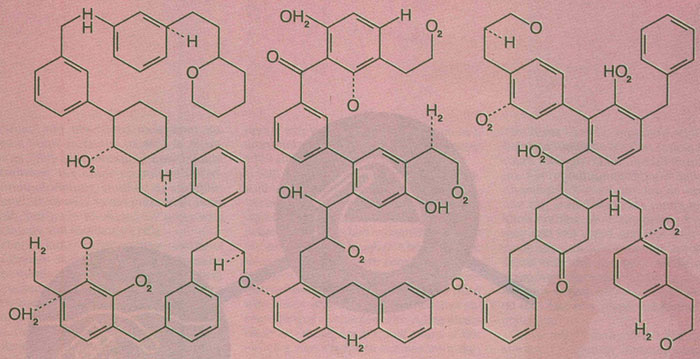
While these structures do not correspond to any known compound – not in and of itself a bad sin – the knowledge (or lack thereof) of chemistry displayed here is simply amazing. The graphic designer is certainly drawn to the preponderance of 6-member rings found in organic chemistry, but has taken this to an extreme! It seems that a random collection of atomic symbols were then willy-nilly added wherever the artist thought would be interesting. The result is simply absurd. One could only have hoped that some copy-editor with a chemistry background could have noticed some of the more glaring mistakes – oh, like five bonds to carbon! For the newspaper of record, these mistakes are simply unwarranted.

To make something good out of this, I am going to hold a contest with my first-semester organic students to identify the errors. The winner will get extra credit in the class – and I will post results here later on.

Here’s another interesting application of computed NMR spectra to resolve the structure of natural products. Braddock and Rzepa have examined obtusallenes V (1), VI (2) and VII (3).¹ The geometries were optimized at mPW1PW91/6-31G(d,p) and the chemical shifts were obtained at this level and using the aug-cc-pVDZ basis set. The larger basis reduces the error and no statistical correction need be applied. The coordinates of these compounds are available through this web-enhanced object of the paper.

The confusion in these structures relates to the position of the halide attachments. For 1 and 2, the problem is which halide (Br or Cl) is at C-7 and C-13. The original structures proposed had these halogens switched from what I’ve drawn, and the correlation between the computed chemical shifts for these original structures and the experiment shows significant deviation: a mean deviation of 1.42 ppm for 1 and 1.67 ppm for 2. Using the structures shown above, along with switching the assigned ¹³C chemical shifts gives much better agreement between the computed and experimental values; the mean deviation is 1.15 ppm for both 1 and 2. Unfortunately the stereochemistry about the allene cannot be determined using NMR – the two different isomers have similar chemical shifts. Similarly, the structure of 3 is predicted as shown above, though the experiment reported only some of the chemical shifts so some uncertainty remains.

References

(1) Braddock, D. C.; Rzepa, H. S., "Structural Reassignment of Obtusallenes V, VI, and VII by GIAO-Based Density Functional Prediction," J. Nat. Prod., 2008, DOI: 10.1021/np0705918.

InChIs

1: InChI=1/C15H18Br3ClO3/c1-8-14(18)12-6-13(17)15(22-12)7-10(19)11(21-15)5-9(20-8)3-2-4-16/h3-4,8-14H,5-7H2,1H3/t2-,8-,9+,10+,11-,12+,13-,14-,15-/m0/s1
InChIKey = PVIUYMGCQVXTIT-JUHTWQEGBT

2: InChI=1/C15H19Br2ClO3/c1-9-14(17)12-4-5-15(20-12)8-11(18)13(21-15)7-10(19-9)3-2-6-16/h3,6,9-14H,4-5,7-8H2,1H3/t2-,9-,10+,11+,12+,13-,14-,15-/m0/s1
InChIKey = WPEZFVRVOYPLJW-LXJGPXSEBA

3: InChI=1/C15H20Br3ClO3/c1-8-15(18)14-6-10(17)13(22-14)7-11(19)12(20)5-9(21-8)3-2-4-16/h3-4,8-15,20H,5-7H2,1H3/t2-,8-,9+,10-,11+,12-,13-,14+,15-/m0/s1
InChIKey = QTZNVLUNNGQAFG-SOAHCKLOBC

A real tour-de-force experimental and computational study of the ORD of 2,3-hexadiene 1 has been produced through the combined efforts of Wiberg, Jorgensen, Crawford, Cheeseman and colleagues.¹ You might not expect a simple compound like 1 to display anything particularly unusual, but you’d be wrong!

2,3-hexadiene exists as three conformations, shown in Figure 1. The cis conformers is the lowest energy form, but the other two are only 0.2 kcal mol^-1 higher in energy, meaning that all three will have significant mol fractions at 0 °C, as listed in Figure 1. The optical rotation for each conformer was determined using B3LYP/aug-cc-pVDZ and CCSD/aug-ccpVDZ. While there is some disagreement in the values determined by the two methods, what is most interesting is that large dependence of [α]_D on the conformation – see Table 1!

cis 0.0 (0.441)

gauche120 0.269 (0.280)

gauche240 0.272 (0.279)

Figure 1. CCSDT optimized geometries of 1, their relative energies (kcal mol^-1) and, in parenthesis, their mol fractions at 0 °C.¹

Table 1. Calculated [α]_D for 1.

^aUsing the aug-ccpVDZ basis set. ^aBoltzman averaged based on the populations shown in Figure 1.

The ORD spectrum of 1 was taken for neat liquid and in the gas phase. The computed and experimental optical rotations are listed in Table 2. Two interesting points can be made from this data. First, the optical activity of 1 is strongly affected by phase. Second, the computed optical rotations, especially the CCSD values, are in fairly good agreement with the gas-phase experimental values.

Table 2. Boltzmann-weighted computed and experimental optical rotations of 1.

A hypothesis to account for the large difference in the gas- and liquid-phase ORD for 1 is that the conformational distribution changes with the phase. The gas and liquid-phase ORD of 2,3-pentadiene shows the same strong phase dependence, even though this compound exists as only one conformer.

Next, a Monte Carlo simulation of gas- and liquid-phase 1 was performed to assess the conformational distributions. Though the range of dihedral angle distributions span about 60°, the population distribution is nearly identical in the two phases – there is no medium-dependence on the conformation distribution, and so this cannot explain the difference in the gas and liquid ORDs.

The authors also tested for the vibrational dependence on the optical rotation. While there is a small correction due to vibrations, it is not enough to account for the differences due to the medium. The origin of this effect remains unexplained.

References

(1) Wiberg, K. B.; Wang, Y. g.; Wilson, S. M.; Vaccaro, P. H.; Jorgensen, W. L.; Crawford, T. D.; Abrams, M. L.; Cheeseman, J. R.; Luderer, M., "Optical Rotatory Dispersion of 2,3-Hexadiene and 2,3-Pentadiene," J. Phys. Chem. A, 2008, DOI: 10.1021/jp076572o.

InChIs

1: InChI=1/C6H10/c1-3-5-6-4-2/h3,6H,4H2,1-2H3/t5-/m1/s1 InChIKey=DPUXQWOMYBMHRN-RXMQYKEDBA

Birney has published another study of a pseudopericyclic reaction to complement the many I discus in Chapter 3.4. Here he looks at that decarbonylation of benzothiophenedione 1, which if analogous to the furandione will first give the ketene 2 before forming 3.¹ Upon gentle heating, 3 dimerizes to 4. Interestingly, 2 has not been detected.²

2 does not exist as a local minimum on the B3LYP/6-31G(d,p) or G3MP2B3 surfaces; rather all optimizations collapse to 3. However, when optimized with PCM with the dielectric of DMSO, 2 is a local minimum.

The transition state for loss of CO from 1 leads directly to 3. This TS (TS1-3, see Figure 1) is non-planar, unlike for the analogous reaction of the furandione. TS1-3 does not correspond with a pseudopericyclic reaction.

TS1-3

TS3-4

Figure 1. B3LYP/6-31G(d,p) optimized geometries of TS1-3 andTS3-4.¹

The transition state for the dimerization of 3 (TS3-4), also shown in Figure 1, appears to be a [2σs + σs] cyclization, which is thermally forbidden. However, analysis of the molecular orbitals indicates the interaction of sets of orthogonal orbitals, exemplary of a pseudopericyclic reaction. The barrier for this reaction, 17.9 kcal mol^-1, is consistent with an allowed pseudopericyclic process.

References

(1) Sadasivam, D. V.; Birney, D. M., "A Computational Study of the Formation and Dimerization of Benzothiet-2-one," Org. Lett., 2008, 10, 245-248, DOI: 10.1021/ol702628v.

(2) Wentrup, C.; Bender, H.; Gross, G., "Benzothiet-2-ones: Synthesis, Reactions, and Comparison with Benzoxet-2-ones and Benzazetin-2-ones," J. Org. Chem., 1987, 52, 3838-3847, DOI: 10.1021/jo00226a022.

InChIs

1: InChI=1/C8H4O2S/c9-7-5-3-1-2-4-6(5)11-8(7)10/h1-4H

2: InChI=1/C7H4OS/c8-5-6-3-1-2-4-7(6)9/h1-4H

3: InChI=1/C7H4OS/c8-7-5-3-1-2-4-6(5)9-7/h1-4H

4: InChI=1/C14H8O2S2/c15-13-9-5-1-3-7-11(9)17-14(16)10-6-2-4-8-12(10)18-13/h1-8H