Dewar benzene has fascinated physical organic chemists for a long time. Just how does this open up? And why is is stable, given its large strain and the aromaticity of the ring-opened product? Most had rationalized this by recognizing that the route that takes Dewar benzene into benzene is the symmetry-forbidden disrotatory path, and the symmetry allowed conrotatory path leads to the benzene with a trans double bond.

Johnson¹ discovered that the conrotatory TS is high in energy, but below the C_2v structure thought to be the disrotatory TS, but is in fact a saddle point. Further, the IRC path through the conrotatory TS connects Dewar benzene to benzene, avoiding the trans-benzene (which is sometimes referred to as Möbius benzene).

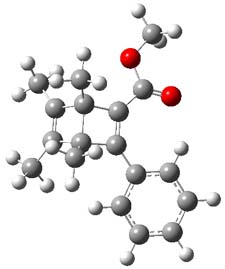
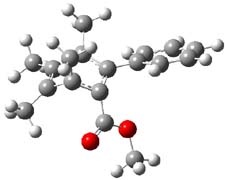
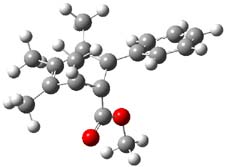
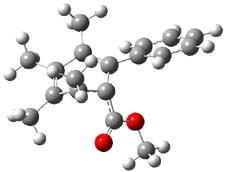
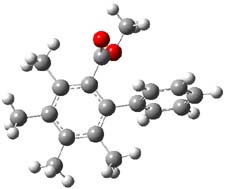
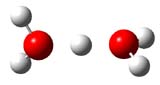
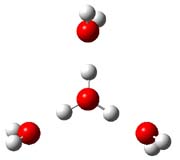
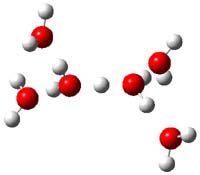
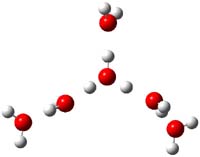
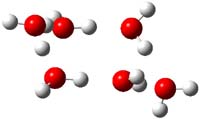
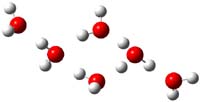
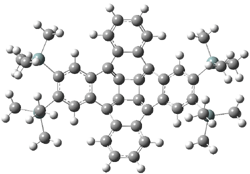
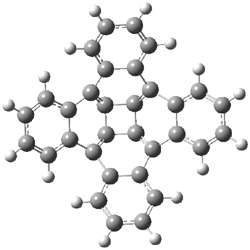
Now comes a report that the PES for opening of Dewar benzene is a bit more complicated.² B3LYP/6-311+G** computations of 1 going to 3 identifies not only the Möat;bius benzene intermediate 2 but a transition state connecting 1 to 2 and a second transition state that connects 2 with 3. These structures are shown in Figure 1.

1	TS1
2	TS2
3

Figure 1. B3LYP/6-311+G** optimized geometries of 1-3 and the two TSs.²

The first TS is 31.0 kcal mol^-1 above reactant and the Möbius benzene intermediate is only 2.1 kcal mol^-1 below this TS. The second TS is 1.6 kcal mol^-1 higher than the first TS, and so is rate-limiting.

The authors also examined a series of related Dewar benzenes and all have two TSs, with the second always higher than the first.

Molecular dynamics computations suggest that the Möbius benzene is likely avoided during the reaction. The fact that the two TSs are similar in energy disguised the fact that there is a second one – the energy of the first TS matches up nicely with experiments. Further MD studies would be interesting to see the interplay between the geometrically quite close intermediate and two TSs – some novel dynamics might be at work here.

References

(1) Johnson, R. P.; Daoust, K. J., "Electrocyclic Ring Opening Modes of Dewar Benzenes: Ab Initio Predictions for Möbius Benzene and trans-Dewar Benzene as New C₆H₆ Isomers," J. Am. Chem. Soc., 1996, 118, 7381-7385, DOI: 10.1021/ja961066q

(2) Dracinsky, M.; Castano, O.; Kotora, M.; Bour, P., "Rearrangement of Dewar Benzene Derivatives Studied by DFT," J. Org. Chem. 2010, ASAP, DOI: 10.1021/jo902065n

InChIs

1: InChI=1/C18H20O2/c1-11-12(2)18(4)15(16(19)20-5)14(17(11,18)3)13-9-7-6-8-10-13/h6-10H,1-5H3
InChIKey=LGAXKHBTTKUURV-UHFFFAOYAH

3: InChI=1/C18H20O2/c1-11-12(2)14(4)17(18(19)20-5)16(13(11)3)15-9-7-6-8-10-15/h6-10H,1-5H3
InChIKey=OUPUSCZNPIJJAH-UHFFFAOYAO

Stoyanov and Reed¹ have reported the IR spectrum of strong acid in water, trying to identify the true nature of the hydrated proton. In other words, what is n in the formula H(H₂O)_n⁺? The key to addressing this problem is to carefully measure the IR spectrum and then remove the signals due to (a) water associated with (or perturbed by) the anion and (b) bulk water. Simply subtracting out bulk water overcorrects because some waters are associated with the proton. By properly scaling the bulk water peak, they identify n as six. Deconvolution of the spectrum of H(H₂O)₆⁺ gives peaks at 3134 ±12, 2816 ± 40, 1746 ± 11, 1202 ± 4 and 654 ± 12 cm^-1.

They suggest that the hydrated proton has structure 3, which is distinguished from previous proposals of H(H₂O)₄⁺ 2 and H(H₂O)₂⁺ 1.

Somewhat surprising is that these authors did not compute the structures of these ions and their IR spectrum. So just to motivate further work I have computed the spectrum of the three ions at PBE1PBE/6-311++G(2df,p)//PBE1PBE/6-31+G(d,p) (Figure 1) and their uncorrected IR frequencies within the range 500-3000 cm^-1 (and intensities greater than 50) are listed in Table 1.

1

2

3

Figure 1. PBE1PBE/6-31+G(d,p) structures of 1 and 3 and PBE1PBE/6-311++G(2df,p) of 2.

Table 1. Computed frequencies (cm^-1) and intensities of 1-3.

The comparison between experiment and computation leaves something to be desired and more careful computation is clearly warranted. In addition, these types of complexes are likely to be dynamic, and so multiple different configurations and conformations will need to be sampled. So, again to promote contributions to this problem, I offer three other configurations of H(H₂O)₆⁺, shown in Figure 2. Their computed IR frequencies are listed in Table 2. Any additional interested takers?

3b -0.48 (0.60)

3c 3.41 (4.80)

3d -1.33 (1.33)

Figure 2. PBE1PBE/6-31+G(d,p) structures of 3b-3d along with their relative (to 3) electronic energy (kcal mol^-1 and electronic energy with ZPE (in parenthesis).

Table 2. Computed frequencies and intensities of 3b-3d.

References

(1) Stoyanov, E. S.; Stoyanova, I. V.; Reed, C. A., "The Structure of the Hydrogen Ion (H_aq⁺) in Water," J. Am. Chem. Soc., 2010, 132, 1484-1485, DOI: 10.1021/ja9101826

Predicting the pK_a of a compound from first principles remains a challenge, despite all of the many algorithmic and methodological advantages within the sphere of computational chemistry. Predicting the gas-phase deprotonation energy is relatively straightforward as I detail in Section 2.2 of my book. The difficulty is in treating the solvent and the interaction of the acid and its conjugate base in solution. Considering that we are most interested in acidities in water, a very polar solvent, the interactions between water and the conjugate base and the proton are likely to be large and important!

Baker and Pulay report a procedure for determining acidities with the aim of high throughput.¹ Thus, computational efficiency is a primary goal. Their approach is to compute the enthalpy change for deprotonation in solution using a continuum treatment and then employ a linear fit to predict the pK_a with the equation:

pK_a(c) = α_cΔH + β_c

where c designates a class of compound, such as alcohol, carboxylic acid, amine, etc. Fitting constants α_c and β_c need to be found then for each unique class of compound, where the fitting is to experimental pK_as in water. In their test suite, they employed eleven anilines and amines, seven pyridines, nine alcohols and phenols, and seven carboxylic acids.

They test a number of different computational variants: (a) what functional to employ, (b) what basis set to use for optimizing structures, and (c) what basis set to use for the enthalpy computation. They opt to employ COSMO for treating the solvent and quickly reject the use of gas phase structures (and particularly use of geometries obtained with molecular mechanics. Their ultimate model is OLYP/6-311+G**//3-21G(d) with the COSMO solvation model. Mean deviation is less than 0.4 pK units. They do note that use of HF or PW91 provides similar small errors, but ultimately favor OLYP for its computational performance.

While this procedure offers some guidance for future computation of acidity, I find a couple of issues. First, it relies on fitted parameters for every class of compound. If one is interested in a new class, then one must develop the appropriate parameters – and the experimental values may not be available or perhaps an insufficient number of them are experimentally available. Second, the parameters cover-up a great deal of problematic computational sins, like the solvation energy of the proton, small basis sets, missing correlation energies, missing dispersion corrections etc. A purist might hope for a computational algorithm that allows for systematic correction and improvement in the estimation of pK_as. Further work needs to be done to meet this higher goal.

References

(1) Zhang, S.; Baker, J.; Pulay, P., "A Reliable and Efficient First Principles-Based Method for Predicting pKa Values. 1. Methodology," J. Phys. Chem. A 2010, 114 , 425-431, DOI: 10.1021/jp9067069

The recent synthesis and characterization of the quadrannulene 1 once again stretches
our notions of aromaticity.¹

The core of this system is a four-member ring with four fused-phenyl rings, forming the very small circulene (see this earlier post on circulenes). One might write other resonance structures for the molecule, which could include a central cyclobutadienyl fragment. However, the X-ray structure and computational analysis rejects any significant contribution of the cyclobutadienyl character. First, the four C-C bond of this central ring are 1.45 Å long, with an NBO bond order of 1.08, signifying single bonds. The bonds from the central 4-member ring are 1.36 Å long with bond order of 1.77 – these are double bonds. NICS computations attest to the central ring (+4.5 ppm) being more like [4]radialene (with a NICS value of -2.6 ppm) than like cyclobutadiene (with a NICS value of +16.5 ppm). The 6-member rings fused to the central ring have NICS values of -2.33 ppm, suggesting a non aromatic character, while the outer rings have NICS values of -10.7ppm, similar to that of benzene. The structure is clearly of radialene form. Nonetheless, the central ring possess extremely pyramidalized carbons, as seen in Figure 1, and their π-orbital axis vector, a measure of the pyramidalization, is 107°, which is similar to the idealized tetrahedral value of 109.47°. Despite this stain, the molecule is thermally stable to 170°C and reacts only slowly with air or base. This molecule will surely inspire further work in the small circulenes.

1

1a

Fig 1. B3LYP/6-311G** structures of 1 and its parent 1a (lacking the TMS groups).¹

References

(1) Bharat, R. B.; Bally, T.; Valente, A.; Cyranski, M. K.; Dobrzycki, L.; Spain, S. M.; Rempala, P.; Chin, M. R.; King, B. T., "Quadrannulene: A Nonclassical Fullerene Fragment," Angew. Chem. Int. Ed. 2009, DOI: 10.1002/anie.200905633

InChIs

1: InChI=1/C44H48Si4/c1-45(2,3)33-21-29-30(22-34(33)46(4,5)6)38-27-19-15-16-20-28(27)40-32-24-36(48(10,11)12)35(47(7,8)9)23-31(32)39-26-18-14-13-17-25(26)37(29)41-42(38)44(40)43(39)41/h13-24H,1-12H3
InChIKey=CDVRNAINHDQBCN-UHFFFAOYAM

1a: InChI=1/C32H16/c1-2-10-18-17(9-1)25-19-11-3-4-12-20(19)27-23-15-7-8-16-24(23)28-22-14-6-5-13-21(22)26(18)30-29(25)31(27)32(28)30/h1-16H
InChIKey=QTVPEOVCCYEZNL-UHFFFAOYAK