If you hadn’t noticed, I am a big fan of the work that Dan Singleton is doing concerning the role of dynamics in discerning reaction mechanisms. Dan’s group has reported another outstanding study combining experiments, traditional QM computations, and molecular dynamics – this time on the Wittig reaction.¹

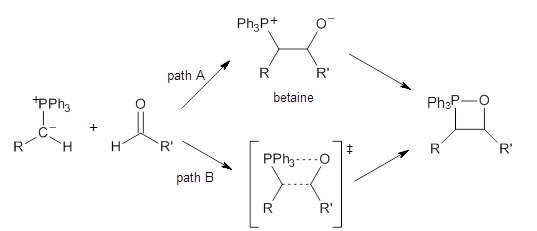
The key question concerning the mechanism is whether a betaine intermediate is accessed along the reaction (path A) or whether the reaction proceeds in a concerted manner (path B). Earlier computations had supported the concerted pathway (B).

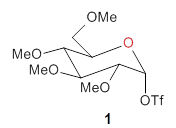
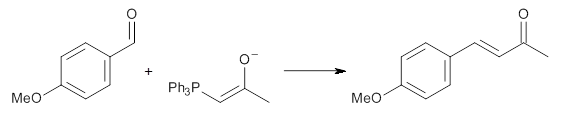
Experimental determination of the heavy atom kinetic isotope effect was made for Reaction 1.

Reaction 1

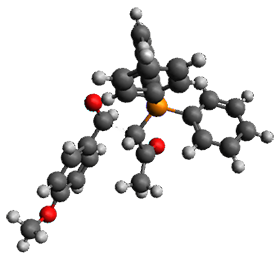
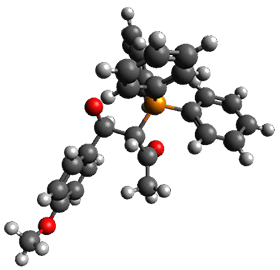
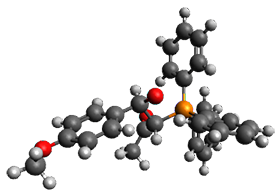
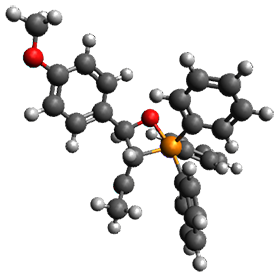
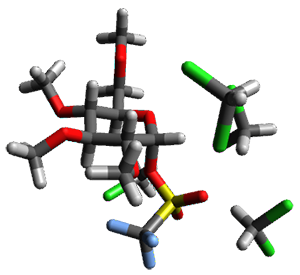
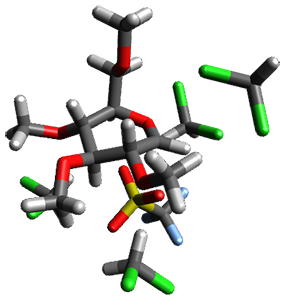
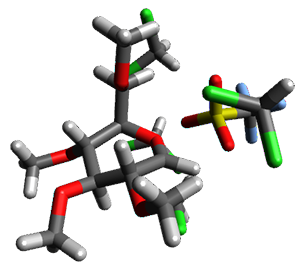
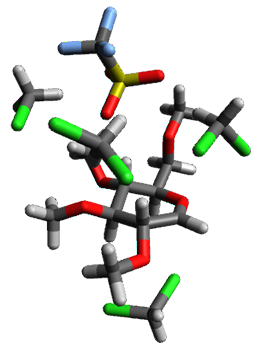
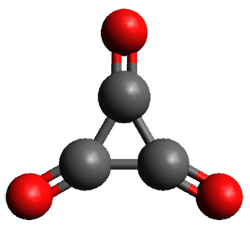
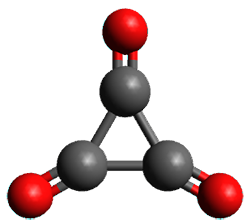
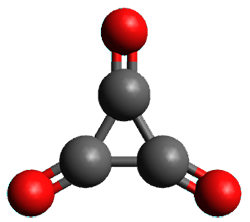
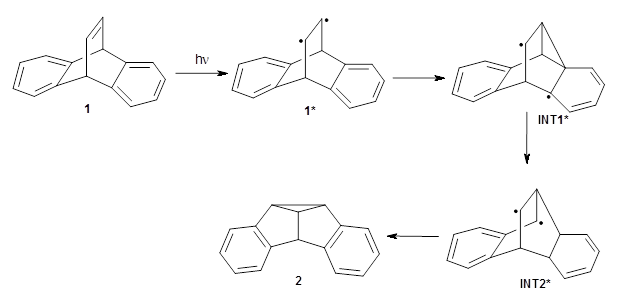
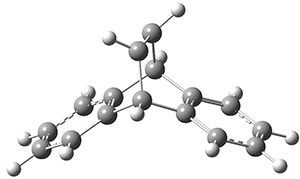
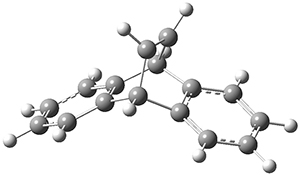
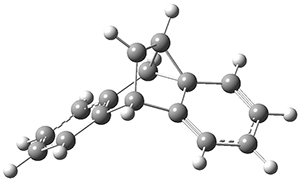
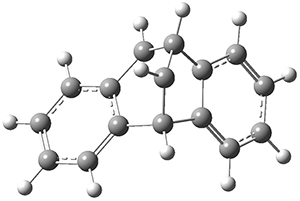
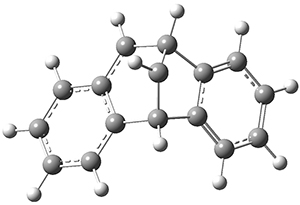
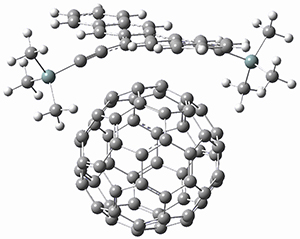
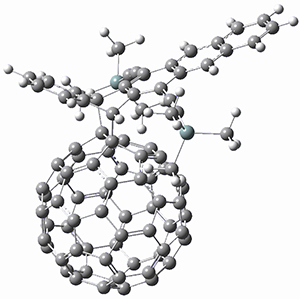
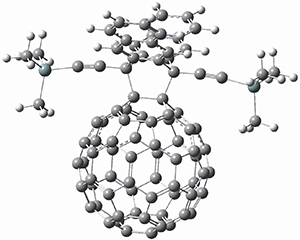
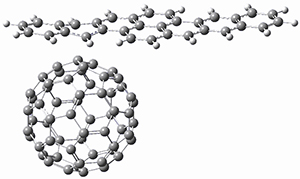
Using the 6-31+G(2df,p) basis set, three different density functionals predict three different potential energy surfaces. With M06-2x, the surface indicates path A (stepwise), with the first step rate-limiting. B3P86 also predicts the stepwise reaction, but the second step is rate-limiting. The Lc-wPBE functional predicts a concerted reaction. Using these surfaces, they predicted the carbon isotope effect and compared it to the experimental values. The best agreement is with the M06-2x surface with a weighting of the vibrational energies of the two different TSs. The optimized structures of the two transition states, the betaine intermediate, and the product are shown in Figure 1.

TS1	Betaine
TS2	Product

Figure 1. M06-2x/6-31+G(2df,p) optimized geometry of the critical points of Reaction 1.

The agreement of the predicted and experimental KIE is not ideal. So, they performed molecular dynamics computations with the ONIOM approach using M06-2x/6-31G* for Reaction 1 and 53 THF molecules treated at PM3. 360 trajectories were begun in the region of the first transition state (TS1), and they can be organized into 4 groups. The first group (128 trajectories) are reactions that produce product. The second group (76 cases) form the C-C bond but then it ruptures and returns to reactant. The third group (82 cases) have an immediate recrossing back to reactant, and the last group (16 cases) takes product back to the first TS and then returns to product. The predicted KIE using this weighted MD results gives values in outstanding agreement with the experiments.

Of the first group, about 50% pass from TS1 to TS2 in less than 150 fs, or in other words look like a concerted path. But a good number of trajectories reside in the betaine region for 1-2 ps.

In contrast, trajectories initiated from the betaine with equilibrated THF molecules indicate a median of 600 ps to travel from TS1 to TS2 and do not resemble a concerted path.

They argue that this bimodal distribution is in part associated with a solvent effect. When the first TS is crossed the solvent molecules are not equilibrated about the solute, and 10-20% of the trajectories immediately pass through the betaine region due to “dynamic matching” where the entering motion matches with exiting over the second transition state. The longer trajectories result from improper dynamic matching, but faster motion in the solute than motion amongst the solvent needed to stabilize the betaine. So, not only do we need to be concerned about dynamic effects involving the reactants, we need to be concerned about dynamics associated with the solvent too!

References

(1) Chen, Z.; Nieves-Quinones, Y.; Waas, J. R.; Singleton, D. A. "Isotope Effects, Dynamic Matching, and Solvent Dynamics in a Wittig Reaction. Betaines as Bypassed Intermediates," J. Am. Chem. Soc. 2014, 136, 13122-13125, DOI: 10.1021/ja506497b.

π-π-stacking has been a major theme of my blog, and is discussed in Chapter 3.5.4 in the Second Edition of my book. Most examples involved molecules that are nearly circular (like benzene or triphenylene). Hartley and co-workers discuss the π-π-stacking of the oblong molecule 1, comparing its experimental features with computed features of the model compound 2.¹

The key spectroscopic feature associated with assembly of 1 are the changes in the ¹H chemical shifts with increasing concentration. For example, the chemical shift of the three protons on the triphenylene unit shift upfield by 0.30 to 0.66 ppm as the concentration increases from 10^-5 to 10^-2 M.

To see if these NMR shift changes are due to association of 1, they employed a computational approach. First they optimized the structure of model compound 2 at B3LYP/6-31G(d) (Shown in Figure 1a). Then using this fixed geometry, they computed the ¹H chemical shifts of the dimer of 2. They explored the stacking distance (ranging from 3.2 to 4.0 Å along with varying the displacement of the two molecules along the major axis from 0.0 to 6.0 Å, finding the best fit to the chemical shifts with a separation of 3.6 Å and a displacement along the major axis of 3.5 Å. Using these two fixed values, they explored displacement of the molecules along the minor axis, along with rotation of the two molecules. The best fit to the experimental chemical shifts was with a displacement of 0.5 Å along the short axis and no rotation. This structure is shown in Figure 1b, with a RMS error of only 0.09 ppm from experiment. Models of the trimer show poorer fit to the experimental data.

(a) 2

(b) 2 dimer

Figure 1. B3LYP/6-31G(d) (a) optimized structure of 2 and the (b) structure of the best fit of the dimer of 2. (As always, clicking on these images will allow you to manipulate the 3-D structure using JMol – highly recommended for the dimer.)

Using some smaller models and the B97-D functional, they argue that the displacement, which is substantially larger than the displacement found in stacked triphenylene, results from the need to minimize the steric interactions between the alkoxyl chains.

References

(1) Chu, M.; Scioneaux, A. N.; Hartley, C. S. "Solution-Phase Dimerization of an Oblong Shape-Persistent Macrocycle," J. Org. Chem. 2014, 79, 9009–9017; DOI: 10.1021/jo501260c.

InChIs:

1: InChI=1S/C116H148O10/c1-11-21-31-41-59-117-95-71-87-51-55-91-75-97-99(103-81-111(121-63-45-35-25-15-5)115(125-67-49-39-29-19-9)85-107(103)105-83-113(123-65-47-37-27-17-7)109(79-101(97)105)119-61-43-33-23-13-3)77-93(91)57-53-89-70-90(74-96(73-89)118-60-42-32-22-12-2)54-58-94-78-100-98(76-92(94)56-52-88(69-87)72-95)102-80-110(120-62-44-34-24-14-4)114(124-66-48-38-28-18-8)84-106(102)108-86-116(126-68-50-40-30-20-10)112(82-104(100)108)122-64-46-36-26-16-6/h69-86H,11-50,59-68H2,1-10H3
InChIKey=UUKOQEVXPHHWBJ-UHFFFAOYSA-N

2: InChI=1S/C76H68O10/c1-11-77-55-31-47-21-25-51-35-57-59(63-41-71(81-15-5)75(85-19-9)45-67(63)65-43-73(83-17-7)69(79-13-3)39-61(57)65)37-53(51)27-23-49-30-50(34-56(33-49)78-12-2)24-28-54-38-60-58(36-52(54)26-22-48(29-47)32-55)62-40-70(80-14-4)74(84-18-8)44-66(62)68-46-76(86-20-10)72(82-16-6)42-64(60)68/h29-46H,11-20H2,1-10H3
InChIKey=NGTKTKCZPONPTB-UHFFFAOYSA-N

Comparing S_N2 and S_N1 reactions using computational methods is often quite difficult. The problem is that the heterolytic cleavage in the S_N1 reaction leads to an ion pair, and in the gas phase this is a highly endothermic process. Optimization of the ion pair in the gas phase invariably leads to recombination. This is disturbingly the result even when one uses PCM to mimic the solvent, which one might have hoped would be sufficient to stabilize the ions.

The computational study of the glycoside cleavage by Hosoya and colleagues offers some guidance towards dealing with this problem.¹ They examined the cleavage of triflate from 2,3,4,6-tetra-O-methyl-α-D-glucopyranosyl triflate 1.

Benchmarking the dissociation energy for the cleavage of 1 and considering computational performance, they settled on M06-2X/BS-III//M06-2X/BS-I, where BS-III is aug-cc-pVTZ basis set for O, F, and Cl and cc-pVTZ for H, C, and S and BS-I is 6-31G(d,p) basis sets were employed for H, C, and S, and 6-31+G(d) for O, F, and Cl. Solvent (dichloromethane) was included through PCM.

Attempted optimization of the contact ion pair formed from cleavage of 1 invariably led back to the covalent bound 1. PCM is not capable of properly stabilizing these types of ions in proximity. To solve this problem, they incorporated four explicit dichloromethane molecules. A minor drawback to their approach is that they did not sample much of configuration space and so their best geometries may not be the lowest energy configurations. Nonetheless, with four solvent molecules, they were able to locate contact ion pairs and solvent-separated ion pairs. Representative examples are shown in Figure 1. This method of explicit incorporation of a few solvent molecules seems to be the direction we must take to treat ions or even highly polar molecules in solution.

1 0.0	1-CIP 8.5
1-SSIPa 8.4	1-SSIPb 11.1

Figure 1. Representative examples of microsolvated 1, its contact ion pair (CIP) and solvent separated ion pair (SSIP) computed at M06-2X/BS-III//M06-2X/BS-I, and relative energies (kcal mol^-1)

References

(1) Hosoya, T.; Takano, T.; Kosma, P.; Rosenau, T. "Theoretical Foundation for the Presence of Oxacarbenium Ions in Chemical Glycoside Synthesis," J. Org. Chem. 2014, 79, 7889-7894, DOI: 10.1021/jo501012s.

InChIs

1: InChI=1S/C11H19F3O8S/c1-17-5-6-7(18-2)8(19-3)9(20-4)10(21-6)22-23(15,16)11(12,13)14/h6-10H,5H2,1-4H3/t6-,7-,8+,9-,10-/m1/s1
InChIKey=RPZNYYCDDYUPJR-HOTMZDKISA-N

Organic chemists are beginning to recognize that tunneling may be more pervasive than previously thought. This blog has noted a number of interesting occurrences of tunneling, and here’s one more, by Karmakar and Datta.¹

The barrier for the intramolecular earrangement (Reaction 1) taking the carbene 1 into 2 is estimated to be 44.1 kcal mol^-1 at M06-2X/6-31+G(d,p), prohibitively large. However, the intermolecular rearrangement (Reaction 2) has a much smaller barrier of 11.4 kcal mol^-1. The structures of the transition states for these two reactions are shown in Figure 1.

TSintra

TSinter

Figure 1. M06-2X/6-31+G(d,p) optimized transition states for Reactions 1 and 2.

Given that the barrier width is likely to be very small for the intramolecular route, perhaps tunneling may play a role. The rate predicted with canonical variational transition-state theory (CVT) and small curvature tunneling (SCT) at 298K is negligible. However, for the intermolecular process, the rate at 298K including tunneling is 3.56 x 10⁴ s^-1, more than 10 times great than predicted with CVT alone, and tunneling makes a dramatically larger difference at lower temperatures.

The intermolecular barrier for the rearrangement of 3 into 4 is very small, only 1.6 kcal mol^-1.
This manifests in a very interesting rate prediction: the reaction is actually predicted to be slower at temperatures above 150K when tunneling is included than when tunneling is omitted. This is a result of quantum mechanical reflection off of the barrier, and this becomes noticeable with the very small barrier. In addition, the kinetic isotope effects are smaller than expected when D is substituted in for H. These predictions await experimental confirmation.

References

(1) Karmakar, S.; Datta, A. "Tunneling Assists the 1,2-Hydrogen Shift in N-Heterocyclic Carbenes," Angew. Chem. Int. Ed. 2014, 53, 9587-9591, DOI: 10.1002/anie.201404368.

InChIs:

1: InChI=1S/C3H6N2/c1-2-5-3-4-1/h4-5H,1-2H2
InChIKey=JKQUEGZDRZXJNY-UHFFFAOYSA-N

2: InChI=1S/C3H6N2/c1-2-5-3-4-1/h3H,1-2H2,(H,4,5)
InChIKey=MTNDZQHUAFNZQY-UHFFFAOYSA-N

3: InChI=1S/C3H2F2N2/c4-2-3(5)7-1-6-2/h6-7H
InChIKey=LHUPDFSUHVZFPD-UHFFFAOYSA-N

4: InChI=1S/C3H2F2N2/c4-2-3(5)7-1-6-2/h1H,(H,6,7)
InChIKey=KXXZDIFMEWOLPE-UHFFFAOYSA-N

As recently explicated by Wang and Borden using NIPE spectroscopy and computations, the potential energy surface of cyclopropyl-1,2,3-trione 1 is remarkably complex.¹ (U)CCSD(T)//aug-cc-pVTZ computations of the D_3h singlet (the ¹A_1’ state shown in Figure 1) is actually a hilltop, possessing two imaginary frequencies. Distorting the structure as indicated by these imaginary frequencies and then optimizing the structure leads directly to dissociation to three CO molecules. Thus, (CO)₃ does not exist as a stable minima on the singlet surface.

The D_3h triplet (the ³E” state shown in Figure 1) is not a critical point on the surface; due to the Jahn-Teller effect is distorts into two different states: the ³B₁ state which is a local energy minimum, and the ³A₂ state which is a transition state between the symmetry-related ³B₁ states.

So, this implies the possibility of a very interesting NIPE experiment. If the radical anion (CO)₃^-.
loses an electron and goes to the singlet surface, it lands at a hilltop(!) and should have a very short lifetime. If it goes to the triplet surface, it lands at either a transition state (³A₂) and again should have a short lifetime, or it can land at the ³B₁ state and perhaps have some lifetime before it dissociates by losing one CO molecule.

1-.
1A1’	3E”
3B1	3A2

Figure 1. (U)CCSD(T)//aug-cc-pVTZ optimized geometries of 1 and its radical anion.

The NIPE spectrum identifies three transitions. By comparing the energies of the electron loss seen in the experiment with the computations, along with calculating the Franck-Condon factors using the computed geometries and vibrational frequencies, the lowest energy transition is to the ¹A_1’ state, and the second transition is part of the vibrational progression also to the ¹A_1’ state. This is the first identification of vibrational frequencies associated with a hilltop structure. The third transition is to the ³A₂ state. No transition to the ³B₁ state is found due to the large geometric difference between the radical anion and the ³B₁ state; the Franck-Condon factors are zero due to no overlap of their wavefunctions.

Once again, the power of the symbiotic relationship between experiment and computation is amply demonstrated in this paper.

References

(1) Chen, B.; Hrovat, D. A.; West, R.; Deng, S. H. M.; Wang, X.-B.; Borden, W. T. "The Negative Ion Photoelectron Spectrum of Cyclopropane-1,2,3-Trione Radical Anion, (CO)₃^•– — A Joint Experimental and Computational Study," J. Am. Chem. Soc. 2014, 136, 12345-12354, DOI: 10.1021/ja505582k.

InChIs

1: InChI=1S/C3O3/c4-1-2(5)3(1)6
InChIKey=RONYDRNIQQTADL-UHFFFAOYSA-N

Herges and co-workers have prepared a triply-twisted Möbius molecule. ¹ The key element is recognizing that most of the “twisting” needs to be accomplished through writhe, a twisting that produces figure-8-like crossing, the way an old-school phone cord twists about itself or the way a pretzel is formed. Herges employs three bi-naphthelene subunits to provide the template for the writhe needed. The prepared compound is 1. A clever, relatively straightforword synthesis produces this amazing molecule, along with the single-twisted 2.

The B3LYP/6-31G* optimized geometries of 1 and the single-twisted analogue 2 are shown in Figure 1. Table 1 presents the key topological parameters of 1 and 2, comparing the computed and X-ray crystal structure. The absolute value of the linking number L_k is 3, indicating the three twists, and the reason that this highly twisted molecule can be made is that half of the twist actually results from writhe.

1

2

Figure 1. B3LYP/6-31G* optimized geometries of the two diastereomers if 1. (Be sure to click on these images to launch JMol and interactively manipulate the structures!)

Table 1. Topological parameters of 1. ^a

^aL_k is the linking number, T_w is the twist number, and W_r is the writhe number, with the condition that T_k + W_r = L_k.

References

(1) Schaller, G. R.; Topić, F.; Rissanen, K.; Okamoto, Y.; Shen, J.; Herges, R. "Design and synthesis of the first triply twisted Möbius annulene," Nat. Chem. 2014, 6, 608-613, DOI: 10.1038/nchem.1955.

InChIs

1: InChI=1S/C72H36/c1-2-26-56-44-38-50-20-8-14-32-62(50)68(56)70-58(46-40-52-22-10-16-34-64(52)70)28-5-6-30-60-48-42-54-24-12-18-36-66(54)72(60)71-59(47-41-53-23-11-17-35-65(53)71)29-4-3-27-57-45-39-51-21-9-15-33-63(51)69(57)67-55(25-1)43-37-49-19-7-13-31-61(49)67/h7-24,31-48H/b69-67-,70-68-,72-71-
InChIkey=ICOGWBLQQNBWJA-UWFYZODKSA-N

I suspect that the majority of my readers have no experience in drawing chemical structures by hand for publication purposes. That’s because of one software product: ChemDraw. I remember using the Fieser triangle (unfortunately no longer sold by Aldrich – click to see a pic and product description!), a plastic template that had standard-sized rings, like nice pentagons and hexagons, and chair and boat conformations of cyclohaxane, and you’d take your fancy ink pen and careful follow the template. Then you moved the template to draw say a bond off of the ring and hoped to god that the ink didn’t smudge. There were other templates for drawing letters and numbers – or you used scratch-off transfer decals. (By this point all of you under 40 are thinking “what the hell is he talking about?”)

Well all of that changed with three seminal events for organic chemists: the introduction of the original Macintosh computer, the introduction of the Apple LaserWriter and the introduction of ChemDraw. The Mac allowed one to sketch in a much more intuitive way – again for those less than 50, computers use to come without a mouse! Imagine trying to draw a chemical structure using a keyboard. That’s why there were no structure drawing tools prior to the Mac. The LaserWriter meant that you could print an output that looked as good as what you had on the screen, and could thus be submitted for publication. And ChemDraw – well this was just astonishing! I still remember the day during my post-doc when the Mac and LaserWriter arrived and we launched ChemDraw and were able to quickly draw molecules – steroid, and conformations, and stereoisomers and they all looked beautiful and we could get them done in a flash!

When I started my first academic position at Northern Illinois University in August 1987 I purchased a Mac and a LaserWriter and ChemDraw as part of my start-up – and I was the first in the department to have a Mac – but that changed rapidly!

So, why all of the teary reminiscences? Well David Evans has just published¹ a nice romp through the mid-1980s recalling how Stewart Rubinstein, aided by Evans and his wife, developed ChemDraw and started CambridgeSoft, and as Stuart Schreiber says “ChemDraw changed the field in a way that has not been replicated since.”

Today, there are other chemical structure drawing tools available, and in fact I no longer use ChemDraw, but it is still a wonder to be able to create drawings so easily and so nicely. Maybe one day I’ll reminisce about the day I got EndNote and my life changed again!

References

1) Evans, D. A. “History of the Harvard ChemDraw Project,” Angew. Chem. Int. Ed. 2014, 53, 1521-3773, DOI 10.1002/anie.201405820.

The di-π-methane photorearrangement has been known for many years, first studied by Zimmerman.^1,2 The triplet photorearrangement gives an interesting rearranged product; and the mechanism of this photorearrangement of 1 into 2 has now been examined by the Houk group using computational techniques, including trajectory analysis. The proposed mechanism is that excitation to the triplet state 1* is followed by rearrangement to the triplet intermediate INT1* which then rearranges to the triplet INT2*. Intersystem crossing then leads to the singlet product 2.

The PES for this rearrangement was explored³ at CASMP2(10,10)/6-31G(d)//CASSCF(10,10)/6-31G(d), with geometries and relative energies shown in Figure 1, as well as at (U)M06-2x/6-31G(d) and (U)B3LYP/6-31G(d); they all give qualitatively the same result. The first TS is the rate limiting step, and the second TS lies only 1-2 kcal mol^-1 above the intermediate INT1. So, the reaction appears to be two steps, but with such a low barrier for the second step, dynamic effects might be important as trajectories might cross INT1* and go over TS2* without residing in the intermediate well for any appreciable time – a seemingly one step reaction. Note than no TS for directly traversing from 1* to INT2* was found.

1* 0.0
TS1* 12.9	INT1* 7.1
TS2* 8.2	INT2* -15.4

Figure 1. CASSCF(10,10)/6-31G(d) geometries and CASMP2 energies in kcal mol^-1.

Now in a follow-up study, Houk and co-workers⁴ performed trajectories analysis on the M06-2x/6-31G(d) PES. A total of 256 trajectories were initiated at TS1* and 241 ended at INT2* within 1500fs. Of these, 24 trajectories resided for less than 60fs within the region of INT1, a time less than a C-C vibration. Furthermore, the lifetime of INT1 that is predicted by RRKM is much longer (about 500fs) than what is observed in the trajectories (about 200 fs). Thus, there is significant dynamic effects in this excited state rearrangement, though INT1 is always sampled.

References

(1) Zimmerman, H. E.; Grunewald, G. L. "The Chemistry of Barrelene. III. A Unique Photoisomerization to Semibullvalene," J. Am. Chem. Soc. 1966, 88, 183-184, DOI: 10.1021/ja00953a045.

(2) Zimmerman, H. E.; Binkley, R. W.; Givens, R. S.; Sherwin, M. A. "Mechanistic organic photochemistry. XXIV. The mechanism of the conversion of barrelene to semibullvalene. A general photochemical process," J. Am. Chem. Soc. 1967, 89, 3932-3933, DOI: 10.1021/ja00991a064.

(3)  Matute, R. A.; Houk, K. N. "The Triplet Surface of the Zimmerman Di-π-Methane Rearrangement of Dibenzobarrelene," Angew. Chem. Int. Ed. 2012, 51, 13097-13100, DOI: 10.1002/anie.201208002.

(4) Jiménez-Osés, G.; Liu, P.; Matute, R. A.; Houk, K. N. "Competition Between Concerted and Stepwise Dynamics in the Triplet Di-π-Methane Rearrangement," Angew. Chem. Int. Ed. 2014,
53, 8664-8667, DOI: 10.1002/anie.201310237.

InChIs

1: InChI=1S/C16H12/c1-2-6-12-11(5-1)15-9-10-16(12)14-8-4-3-7-13(14)15/h1-10,15-16H
InChIKey=VWDKVBGOVYWYFZ-UHFFFAOYSA-N

2: InChI=1S/C16H12/c1-3-7-11-9(5-1)13-10-6-2-4-8-12(10)15-14(11)16(13)15/h1-8,13-16H
InChIKey=RATAQXOLJVRERC-UHFFFAOYSA-N

Diels-Alder reaction involving fullerenes have been known for some time. They occur across the [6,6] double bond of C₆₀, the one between two fused 6-member rings. Houk and Briseno report on the Diels-Alder reaction of C₆₀ with pentacene 1 and bistetracene 2 and compare their computations with experiments.¹

Pentacene and bistetracene ring numbering convention

Computations were performed for the reaction of 1 and 2 with C₆₀ at M06-2x/6-31G(d)//M062x-3-21G*. The reaction can occur with the dienophile being either ring 1, 2, or 3 of pentacene and ring 1, 2, 3, or 4 of bistetracene. They located TSs and products for all of these possibilities. Select TSs and products are shown in Figure 1.

For the reaction of 1a, the lowest energy TS is for the reaction at the central ring (ring 3), and the resulting product is the lowest energy product. The transition state (PT_TS3) is shown in Figure 1. This TS has the least distortion energy of the three possibilities, because reacting at this central ring destroys the least amount of aromaticity of pentacene. For the reaction of 1b, the lowest barrier is again for reaction of ring 3 (through TMSPT_TS3). However, the product from the reaction with ring 2 (TMSPT_P2) is lower in free energy than TMSPT­_P3, likely caused by steric interactions with the silyl substituents. This actually matches up with experiments which indicate that an analogue of TMSPT_P2 is the kinetic product but TMSPT_P3 is the thermodynamic product.

PT_TS3
TMSPT_­TS3
TMSPT_P2	TMSPT_P3
BT_TS2	BT_P2

Figure 1. M06-2x/3-21G* optimized geometries.
(Once again a reminder that clicking on any of these structures will launch JMol and you’ll be able to visualize and manipulate this structure in 3-D.)

The computations involving the Diels-Alder reaction of C₆₀ with either 2a or 2b come to the same conclusion. In both cases, the lowest barrier is for the reaction at ring 2, and the product of the reaction at this same ring is the only one that is endoergonic. The geometries of BT_TS2 and BT_P2 are shown in Figure 1. More importantly, the barrier for the Diels-Alder reaction involving 2a and 2b are at least 6 kcal mol^-1 higher than the barriers for the reaction of 1a and 1b, in complete agreement with experiments that show little reaction involving analogues of 2b with C₆₀, while analogues of 1b are reasonably rapid.

References

(1) Cao, Y.; Liang, Y.; Zhang, L.; Osuna, S.; Hoyt, A.-L. M.; Briseno, A. L.; Houk, K. N. "Why Bistetracenes Are Much Less Reactive Than Pentacenes in Diels–Alder Reactions with Fullerenes," J. Am. Chem. Soc. 2014, 136, 10743-10751, DOI: 10.1021/ja505240e.

The Journal of Chemical Physics has produced a Special Topics issue on Advances in Density Functional Theory. I want to call to your attention the Perspective article by Becke titled “Perspective: Fifty years of density-functional theory in chemical physics”.¹ Becke writes a personal account of the history of DFT and makes a number of interesting points and observations. He rightly notes that DFT is exact and we should more properly refer to our actual implementations as Density Functional Approximations (DFA). He also notes that use of the term ab initio as a synonym for wavefunction theory is inappropriate as DFT is just as ab initio as HF and post-HF theories.

A common perception about DFT (well, DFA) is that there is no way to systematically improve functionals. Becke exposes a true underlying logic that has driven much of DFA development.

Lastly, Becke is discouraged by the more recent developments that have included virtual orbitals, such as double hybrid methods. His approach is that true DFT is occupied orbitals only (for which he pointedly does not want to adopt the acronym OOO), and that developments that include the virtual orbitals might toll the “death knell” for DFT.

For those interested in a pretty accessible account of the history of DFT, Becke’s Perspective is an excellent place to get started.

References

(1) Becke, A. D. "Perspective: Fifty years of density-functional theory in chemical physics," J. Chem. Phys. 2014, 140, 18A301 DOI: 10.1063/1.4869598.