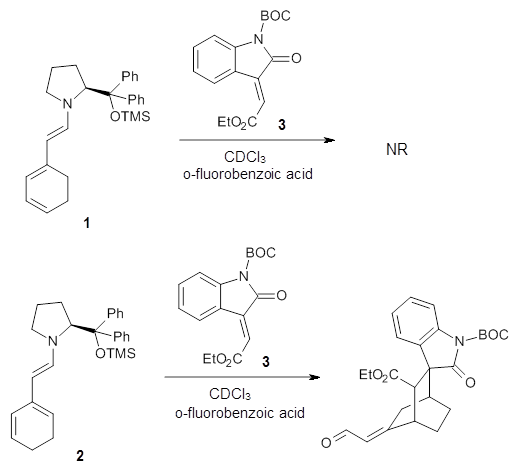
Halskov, et al.1 reported the interesting Diels-Alder selectivity shown in Scheme 1. The linear trienamine 1 did not undergo the Diels-Alder addition, while the less stable cross-conjugated diene 2 does react with 3 with high diastereo- and enantioselectivity. Their MPW1K/6-31+G(d,p) computations on a model system, carried out for a gas-phase environment, indicated a concerted mechanism, with thermodynamic control. However, the barrier for the reverse reaction for the kinetic product was computed to be greater than 30 kcal mol-1, casting doubt on the possibility of thermodynamic control.
Scheme 1.
Houk and co-workers2 have re-examined this reaction with the critical addition of performing the computation including the solvent effects. Since the stepwise alternatives involve the formation of zwitterions, solvent can be critical in stabilizing these charge-separated species, intermediates that might be unstable in the gas phase. Henry Rzepa has pointed out in his blog and on many comments in this blog about the need to include solvent, and this case is a prime example of the problems inherent in neglecting solvation.
Using models of the above reaction Houk located two zwitterionic intermediates of the Michael addition for both the reactions of 4 with 6 and of 5 with 6. The second step then involves the closure of the ring to give what would be Diels-Alder products. This is shown in Scheme 2. They were unable to locate transition states for any concerted pathways. The computations were done at M06-2x/def2-TZVPP/IEFPCM//B97D/6-31+G(d,p)/IEFPCM, modeling trichloromethane as the solvent.
Scheme 2. Numbers in italics are energies relative to 4 + 6.
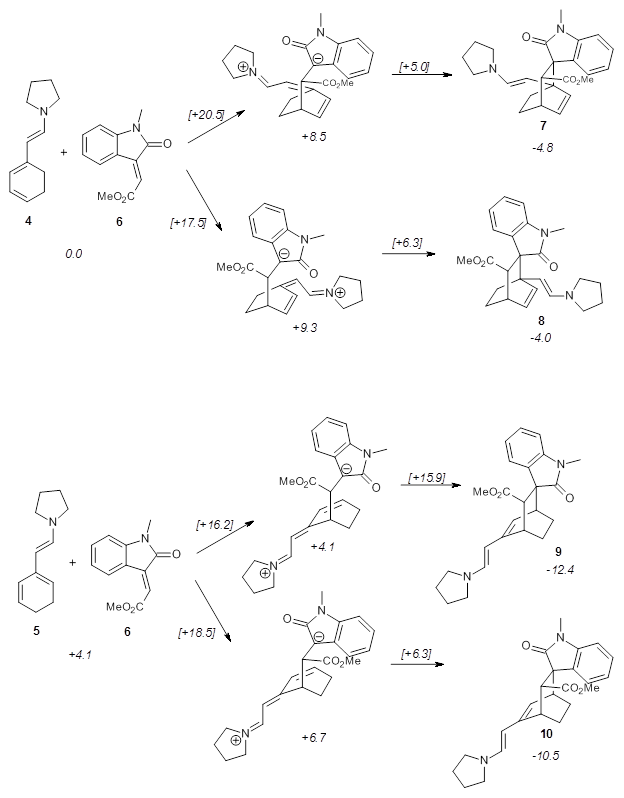
The activation barrier for the second step in each reaction is very small, typically less than 5 kcal mol-1, so the first step is rate determining. The lowest barrier is for the reaction of 5 leading to 9, analogous to the observed product. Furthermore, 9 is also the thermodynamic product. Thus, the regioselectivity is both kinetically and thermodynamically controlled through a stepwise reaction. This conclusion is only possible by including solvent in order to stabilize the zwitterionic intermediates, and should be a word of caution for everyone doing computations: be sure to include solvent for any reactions that involved charged or charge-separated species at any point along the reaction pathway!
References
(1) Halskov, K. S.; Johansen, T. K.; Davis, R. L.; Steurer, M.; Jensen, F.; Jørgensen, K. A. "Cross-trienamines in Asymmetric Organocatalysis," J. Am. Chem. Soc. 2012, 134, 12943-12946,
DOI: 10.1021/ja3068269.
(2) Dieckmann, A.; Breugst, M.; Houk, K. N. "Zwitterions and Unobserved Intermediates in Organocatalytic Diels–Alder Reactions of Linear and Cross-Conjugated Trienamines," J. Am. Chem. Soc. 2013, 135, 3237-3242, DOI: 10.1021/ja312043g.

Henry Rzepa responded on 03 Apr 2013 at 12:18 am #
A simple rule of thumb can also help to decide on the importance of solvation. If a putative transition state has a dipole moment of > 10D, you will certainly need to include it. Even ~6-10D can have a noticeable effect on the (relative) energies. More or less fully charge separated intermediates (we might call them eg zwitterions) will have dipole moments upwards of 15D, and the largest I have seen myself can reach >30D.
I also suspect that one should inspect the quadrupole moment as well.
Henry Rzepa responded on 04 Apr 2013 at 11:01 am #
Life used to be simple; single kinetic steps were either stepwise or concerted. Charge separation either resulted in a stepwise reaction with an ionic intermediate or not. But things are nowdays seen to be rather more blurred. Thus the concept of hidden intermediates in reactions, where a concerted mechanism almost but not quite is interrupted by incursion of intermediates. I came across a rather nice example of this in looking at the rearrangement of enantiomerically pure derivatives of Feist’s acid (methylene cyclopropane to purists). This rearranges without loss of optical activity, and so might be inferred as concerted. But there is much speculation about the role or not of intermediates, either explicit (biradicals) or indeed hidden.
Actually, when one sees quite how common such features actually are, one realises how important dynamics and the statistical distribution of reaction trajectories is. We may only hope that computers continue to (exceed) Moore’s law, and that algorithms continue to speedily improve, so that dynamic treatment of reaction mechanisms becomes the norm rather than the exception.
Christine responded on 13 Jun 2013 at 10:59 am #
Is it just me who finds it a bit sketchy that the transition state going from the zwitterionic intermediate to compound 7 is lower in energy than the previous intermediate? (Apologies if this is a dumb question. I am an organic chemistry PhD student and amateur computational chemist.)
Steven Bachrach responded on 13 Jun 2013 at 12:23 pm #
Christine – the TS in question is 5.0 kcal mol-1 above the intermediate, or 13.5 kcal mol-1 above reactants. The numbers above the arrows are the barrier heights (relative to the reactant of that step and not to the starting materials).